Sullivan Microsite DigiSample

Sullivan AP˙Sullivan˙Chapter01 October 8, 2016 17:4
Sullivan
134 Chapter 1 • Limits and Continuity
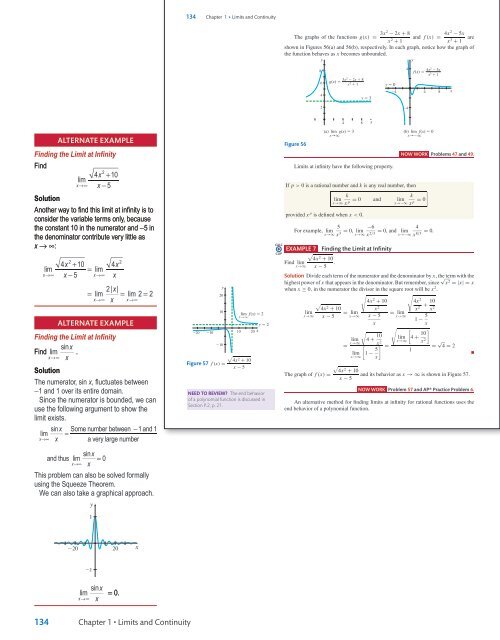
The graphs of the functions g(x) = 3x 2 − 2x + 8
and f (x) = 4x 2 − 5x
are
x 2 + 1
x 3 + 1
shown in Figures 56(a) and 56(b), respectively. In each graph, notice how the graph of
the function behaves as x becomes unbounded.
y
y
8
6
4
g(x)
3x 2 2x 8
x 2 1
y 3
y 0
4
4
f(x)
4x 2 5x
x 3 1
4 8
x
2
4
Alternate Example
Finding the Limit at Infinity
Find
x +
lim 4 2
10
x→∞
x − 5
Solution
Another way to find this limit at infinity is to
consider the variable terms only, because
the constant 10 in the numerator and −5 in
the denominator contribute very little as
x → ∞:
x +
x
lim 4 2 10 = lim 4 2
x→∞
x − 5 x→∞
x
x
= lim 2| | = lim 2=
2
x→∞
x x→∞
Alternate Example
Finding the Limit at Infinity
x
Find lim sin .
x→∞
x
Solution
The numerator, sin x, fluctuates between
−1 and 1 over its entire domain.
Since the numerator is bounded, we can
use the following argument to show the
limit exists.
sinx
Some number between −1 and 1
lim ≈
x→∞
x a very large number
x
and thus lim sin = 0
x→∞
x
This problem can also be solved formally
using the Squeeze Theorem.
We can also take a graphical approach.
y
1
20
10
y
20
10
10
Figure 57 f (x) =
lim f (x) 2
x →∞
y 2
10 20 x
√
4x 2 + 10
x − 5
NEED TO REVIEW? The end behavior
of a polynomial function is discussed in
Section P.2, p. 21.
CALC
CLIP
Figure 56
4
8
x
(a) lim g(x) 3 (b) lim
f(x) 0
x→∞ x→ ∞
Limits at infinity have the following property.
If p > 0 is a rational number and k is any real number, then
provided x p is defined when x < 0.
k
lim = 0 and lim
x→∞ x
p
5
−6
For example, lim = 0, lim = 0, and
x→∞ x
3 x→∞ x lim
2/3
EXAMPLE 7 Finding the Limit at Infinity
√
4x
2
+ 10
Find lim
.
x→∞ x − 5
NOW WORK Problems 47 and 49.
x→−∞
x→−∞
k
x = 0 p
4
= 0.
x
8/3
Solution Divide each term of the numerator and the denominator by x, the term with the
highest power of x that appears in the denominator. But remember, since √ x 2 =|x| =x
when x ≥ 0, in the numerator the divisor in the square root will be x 2 .
lim
x→∞
√
4x
2
+ 10
x − 5
= lim
x→∞
√
4x 2 + 10
x 2
x − 5
= lim
x→∞
x
√
√
lim 4 + 10
x→∞
= [ x 2
lim 1 − 5 ] =
x→∞ x
lim
x→∞
√
4x 2
x + 10
2 x 2
1 − 5 x
[
4 + 10 ]
x 2
1
= √ 4 = 2
√
4x
2
+ 10
The graph of f (x) =
x − 5
and its behavior as x →∞is shown in Figure 57.
NOW WORK Problem 57 and AP® Practice Problem 6.
An alternative method for finding limits at infinity for rational functions uses the
end behavior of a polynomial function.
■
220
20
x
21
x→∞
x
x
lim sin
= 0.
134
Chapter 1 • Limits and Continuity
TE_Sullivan_Chapter01_PART II.indd 17
11/01/17 9:56 am