Sullivan Microsite DigiSample

Teaching Tip
Alternate Example
Finding the Limit of a Trigonometric
Function
x x+
x
Find lim 2 csc 2
csc .
x→0
2
xcsc
x
Solution
This time, we rewrite in terms of sines:
x x+
x
lim 2 csc 2
csc
x→0
2
xcsc
x
⎛ 2x
1 ⎞
⎜ 2 ⎟
= lim sin x
⎜ + sin x
⎟
x→0
⎜
x x
⎟
⎝
2 2
sin x sin x ⎠
⎛ x x x ⎞
= lim ⎜
2 sin 2 2
sin
+
x→0
2 ⎟
⎝ xsin
x xsin
x⎠
⎛ sin x ⎞
= lim +
⎝
⎜ 2
⎠
⎟ = 2 + 1 = 3
x→0
x
Chapter objective 3: Determine where the
trigonometric functions are continuous is a
good review. This knowledge is a calculus
prerequisite, so if the students are well
prepared, you may be able to address this
objective briefly to save time.
AP® CaLC skill builder
for example 4
Finding the Limit of a Trigonometric
Function
Sullivan AP˙Sullivan˙Chapter01 October 8, 2016 17:4
122 Chapter 1 • Limits and Continuity
2π π π π
π
2 2
y
1
1
y
1
1
y cos x
y sin x
3π
2
2π π π π π 3π
2π x
2 2
2
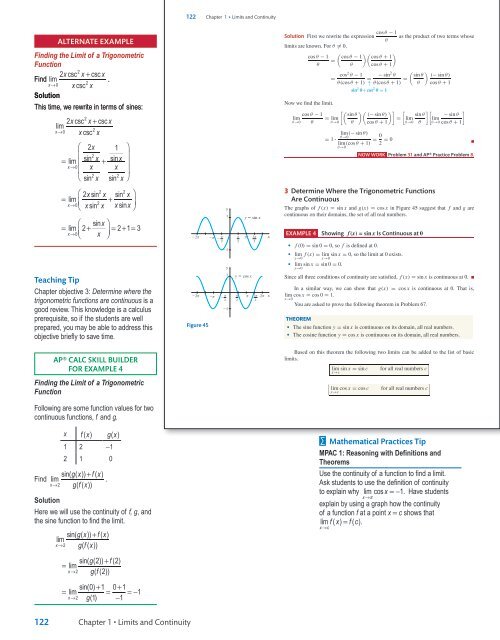
Figure 45
x
Sullivan
Solution First we rewrite the expression cos θ − 1 as the product of two terms whose
θ
limits are known. For θ = 0,
( )( )
cos θ − 1 cos θ − 1 cos θ + 1
=
θ
θ cos θ + 1
= cos2 θ − 1
θ(cos θ + 1) = − sin 2 ( )
θ sin θ (− sin θ)
↑ θ(cos θ + 1) = θ cos θ + 1
sin 2 θ+ cos 2 θ = 1
Now we find the limit.
[( )( )] [ ][
]
cos θ − 1 sin θ (− sin θ)
sin θ − sin θ
lim = lim
= lim lim
θ→0 θ θ→0 θ cos θ + 1 θ→0 θ θ→0 cos θ + 1
lim(− sin θ)
θ→0
= 1 ·
lim (cos θ + 1) = 0 2 = 0
■
θ→0
NOW WORK Problem 31 and AP® Practice Problem 8.
3 Determine Where the Trigonometric Functions
Are Continuous
The graphs of f (x) = sin x and g(x) = cos x in Figure 45 suggest that f and g are
continuous on their domains, the set of all real numbers.
EXAMPLE 4 Showing f(x) = sin x Is Continuous at 0
• f (0) = sin 0 = 0, so f is defined at 0.
• lim f (x) = lim sin x = 0, so the limit at 0 exists.
x→0 x→0
• lim sin x = sin 0 = 0.
x→0
Since all three conditions of continuity are satisfied, f (x) = sin x is continuous at 0. ■
In a similar way, we can show that g(x) = cos x is continuous at 0. That is,
lim cos x = cos 0 = 1.
x→0
You are asked to prove the following theorem in Problem 67.
THEOREM
• The sine function y = sin x is continuous on its domain, all real numbers.
• The cosine function y = cos x is continuous on its domain, all real numbers.
Based on this theorem the following two limits can be added to the list of basic
limits.
lim sin x = sin c for all real numbers c
x→c
lim cos x = cos c for all real numbers c
x→c
Following are some function values for two
continuous functions, f and g.
x fx ( ) gx ( )
1 2 −1
2 1 0
sin( gx ( )) + fx ( )
Find lim .
x→2
gf (( x))
Solution
Here we will use the continuity of f, g, and
the sine function to find the limit.
sin( gx ( )) + fx ( )
lim
x→2
gf (( x))
∑ Mathematical Practices Tip
MPAC 1: Reasoning with Definitions and
Theorems
Use the continuity of a function to find a limit.
Ask students to use the definition of continuity
to explain why lim cosx
=−1.
Have students
x→π
explain by using a graph how the continuity
of a function f at a point x = c shows that
lim f( x) = f( c).
x→c
sin( g(2)) + f(2)
= lim
x→2
gf ((2))
+ +
= lim sin(0) 1 0 1 =
− =− 1
x→2
g(1)
1
122
Chapter 1 • Limits and Continuity
TE_Sullivan_Chapter01_PART II.indd 5
11/01/17 9:55 am