Proceedings of International Conference on Physics in ... - KEK
Proceedings of International Conference on Physics in ... - KEK
Proceedings of International Conference on Physics in ... - KEK
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
Next, let us c<strong>on</strong>sider the orig<strong>in</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g> the divergence <str<strong>on</strong>g>of</str<strong>on</strong>g> w <strong>in</strong><br />
terms <str<strong>on</strong>g>of</str<strong>on</strong>g> effective dimensi<strong>on</strong>al reducti<strong>on</strong> <strong>in</strong> a str<strong>on</strong>g magnetic<br />
field. When a magnetic field exists, the spectrum <str<strong>on</strong>g>of</str<strong>on</strong>g><br />
the transverse directi<strong>on</strong> is discretized by Landau quantizati<strong>on</strong>.<br />
Actually, the energy spectrum for E=0 is given by<br />
ε = ± √ p 2 z + 2eB(n + 1/2 ∓ sz) + m 2 , (7)<br />
where n = 0, 1, · · · corresp<strong>on</strong>d to the Landau levels,<br />
and sz = ±1/2 is the sp<strong>in</strong>. The system effectively becomes<br />
1+1 dimensi<strong>on</strong>al system with <strong>in</strong>f<strong>in</strong>ite tower <str<strong>on</strong>g>of</str<strong>on</strong>g> massive<br />
state: m 2 n,eff ≡ 2eBn + m2 . For the lowest Landau<br />
level (LLL), n = 0 and s = +1/2, the energy is<br />
ε = ± √ p 2 z + m 2 . This is the spectrum <strong>in</strong> 1+1 dimensi<strong>on</strong>s.<br />
This LLL causes the divergence <str<strong>on</strong>g>of</str<strong>on</strong>g> w as will be shown below.<br />
The divergence <str<strong>on</strong>g>of</str<strong>on</strong>g> w does not mean the divergence <str<strong>on</strong>g>of</str<strong>on</strong>g><br />
the <strong>in</strong>f<strong>in</strong>ite pair producti<strong>on</strong> per unit space-time. The divergence<br />
<str<strong>on</strong>g>of</str<strong>on</strong>g> w rather implies that the vacuum always decays<br />
and produces pairs <str<strong>on</strong>g>of</str<strong>on</strong>g> fermi<strong>on</strong>. The questi<strong>on</strong> is where the<br />
vacuum goes. In the coexistence <str<strong>on</strong>g>of</str<strong>on</strong>g> B and E, <strong>on</strong>e can obta<strong>in</strong><br />
the probability <str<strong>on</strong>g>of</str<strong>on</strong>g> the n pairs <str<strong>on</strong>g>of</str<strong>on</strong>g> fermi<strong>on</strong> with LLL as<br />
|〈n pairs|Ω<strong>in</strong>〉| 2<br />
= exp<br />
[<br />
V eB<br />
−<br />
4π2 ( ∫<br />
eET −<br />
dpznpz<br />
)<br />
ln eE<br />
πm2 ]<br />
.<br />
The vacuum persistency probability corresp<strong>on</strong>ds to all<br />
npz’s be<strong>in</strong>g zero <strong>in</strong> Eq. (8), and w is equal to Eq. (5), so<br />
that w diverges at m = 0. At m = 0, this probability is<br />
f<strong>in</strong>ite <strong>on</strong>ly if the follow<strong>in</strong>g equati<strong>on</strong> is satisfied:<br />
∫<br />
eET − dpznpz = 0. (9)<br />
Therefore, the number <str<strong>on</strong>g>of</str<strong>on</strong>g> the particle with the LLL is restricted<br />
by Eq. (9), and l<strong>in</strong>early <strong>in</strong>creases with time. The<br />
higher Landau levels give heavy effective masses <str<strong>on</strong>g>of</str<strong>on</strong>g> order<br />
eB, so that all the c<strong>on</strong>tributi<strong>on</strong>s to the pair producti<strong>on</strong>s<br />
from such modes are suppressed. The total number <str<strong>on</strong>g>of</str<strong>on</strong>g> the<br />
particle pairs can be calculated:<br />
N = V T e2 E 2<br />
4π 3<br />
πB<br />
E<br />
(8)<br />
πB<br />
coth . (10)<br />
E<br />
At B = 0, N = V T e 2 E 2 /(4π 3 ). The c<strong>on</strong>tributi<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g> LLL<br />
is obta<strong>in</strong>ed as<br />
N = V T e2 E 2<br />
4π 3<br />
πB<br />
, (11)<br />
E<br />
which is equal to tak<strong>in</strong>g coth(πB/E) → 1 <strong>in</strong> Eq. (10). In<br />
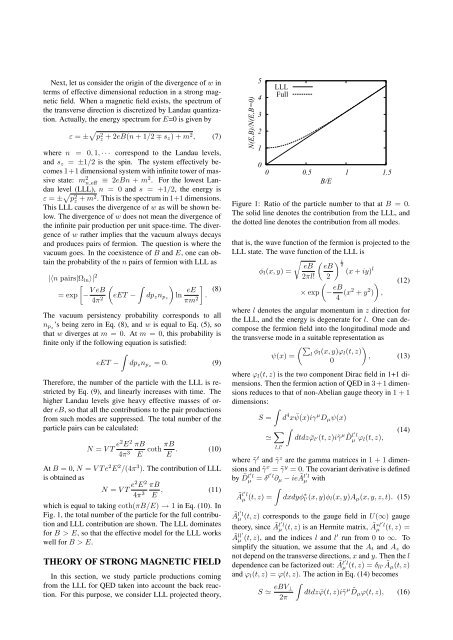
Fig. 1, the total number <str<strong>on</strong>g>of</str<strong>on</strong>g> the particle for the full c<strong>on</strong>tributi<strong>on</strong><br />
and LLL c<strong>on</strong>tributi<strong>on</strong> are shown. The LLL dom<strong>in</strong>ates<br />
for B > E, so that the effective model for the LLL works<br />
well for B > E.<br />
THEORY OF STRONG MAGNETIC FIELD<br />
In this secti<strong>on</strong>, we study particle producti<strong>on</strong>s com<strong>in</strong>g<br />
from the LLL for QED taken <strong>in</strong>to account the back reacti<strong>on</strong>.<br />
For this purpose, we c<strong>on</strong>sider LLL projected theory,<br />
N(E,B)/N(E,B=0)<br />
5<br />
4<br />
3<br />
2<br />
1<br />
0<br />
LLL<br />
Full<br />
0 0.5 1 1.5<br />
B/E<br />
Figure 1: Ratio <str<strong>on</strong>g>of</str<strong>on</strong>g> the particle number to that at B = 0.<br />
The solid l<strong>in</strong>e denotes the c<strong>on</strong>tributi<strong>on</strong> from the LLL, and<br />
the dotted l<strong>in</strong>e denotes the c<strong>on</strong>tributi<strong>on</strong> from all modes.<br />
that is, the wave functi<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g> the fermi<strong>on</strong> is projected to the<br />
LLL state. The wave functi<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g> the LLL is<br />
√ ( ) l<br />
2<br />
eB eB<br />
φl(x, y) =<br />
(x + iy)<br />
2πl! 2<br />
l<br />
(<br />
× exp − eB<br />
4 (x2 + y 2 ) (12)<br />
) ,<br />
where l denotes the angular momentum <strong>in</strong> z directi<strong>on</strong> for<br />
the LLL, and the energy is degenerate for l. One can decompose<br />
the fermi<strong>on</strong> field <strong>in</strong>to the l<strong>on</strong>gitud<strong>in</strong>al mode and<br />
the transverse mode <strong>in</strong> a suitable representati<strong>on</strong> as<br />
(∑<br />
ψ(x) = l φl(x,<br />
)<br />
y)ϕl(t, z)<br />
, (13)<br />
0<br />
where ϕl(t, z) is the two comp<strong>on</strong>ent Dirac field <strong>in</strong> 1+1 dimensi<strong>on</strong>s.<br />
Then the fermi<strong>on</strong> acti<strong>on</strong> <str<strong>on</strong>g>of</str<strong>on</strong>g> QED <strong>in</strong> 3+1 dimensi<strong>on</strong>s<br />
reduces to that <str<strong>on</strong>g>of</str<strong>on</strong>g> n<strong>on</strong>-Abelian gauge theory <strong>in</strong> 1 + 1<br />
dimensi<strong>on</strong>s:<br />
∫<br />
S = d 4 x ¯ ψ(x)iγ µ Dµψ(x)<br />
∑<br />
∫<br />
dtdz ¯ϕl ′(t, z)i˜γµ D˜ l ′ l<br />
µ ϕl(t, z),<br />
(14)<br />
l,l ′<br />
where ˜γ t and ˜γ z are the gamma matrices <strong>in</strong> 1 + 1 dimensi<strong>on</strong>s<br />
and ˜γ x = ˜γ y = 0. The covariant derivative is def<strong>in</strong>ed<br />
by ˜ Dl′ l<br />
µ = δl′ l∂µ − ieÃl′ l<br />
µ with<br />
à l′ l<br />
µ (t, z) =<br />
∫<br />
dxdyφ ∗ l ′(x, y)φl(x, y)Aµ(x, y, z, t). (15)<br />
à l′ l<br />
µ (t, z) corresp<strong>on</strong>ds to the gauge field <strong>in</strong> U(∞) gauge<br />
theory, s<strong>in</strong>ce Ãl′ l<br />
µ (t, z) is an Hermite matrix, Ã∗l′ l<br />
µ (t, z) =<br />
à ll′<br />
µ (t, z), and the <strong>in</strong>dices l and l ′ run from 0 to ∞. To<br />
simplify the situati<strong>on</strong>, we assume that the At and Az do<br />
not depend <strong>on</strong> the transverse directi<strong>on</strong>s, x and y. Then the l<br />
dependence can be factorized out: Ãl′ l<br />
µ (t, z) = δll ′õ(t, z)<br />
and ϕl(t, z) = ϕ(t, z). The acti<strong>on</strong> <strong>in</strong> Eq. (14) becomes<br />
S eBV⊥<br />
2π<br />
∫<br />
dtdz ¯ϕ(t, z)i˜γ µ Dµϕ(t, ˜ z), (16)