Polymers in Confined Geometry.pdf

2.5. REAL CHAINS 15
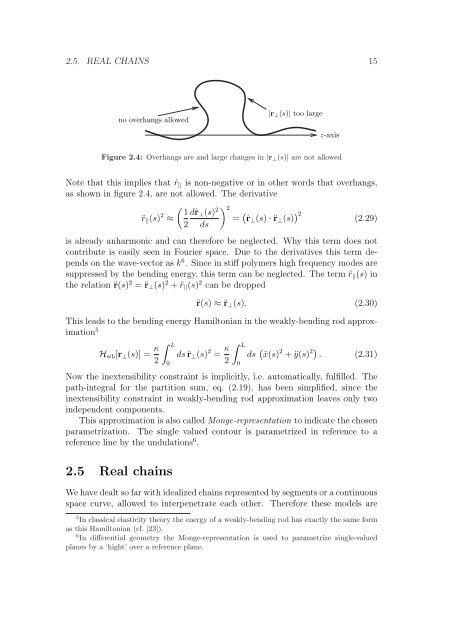
no overhangs allowed
|r⊥(s)| too large
z-axis
Figure 2.4: Overhangs are and large changes in |r⊥(s)| are not allowed
Note that this implies that ˙r|| is non-negative or in other words that overhangs,
as shown in figure 2.4, are not allowed. The derivative
¨r||(s) 2
1 d˙r⊥(s)
≈
2
2 2 =
ds
˙r⊥(s) · ¨r⊥(s) 2 (2.29)
is already anharmonic and can therefore be neglected. Why this term does not
contribute is easily seen in Fourier space. Due to the derivatives this term depends
on the wave-vector as k 6 . Since in stiff polymers high frequency modes are
suppressed by the bending energy, this term can be neglected. The term ¨r||(s) in
the relation ¨r(s) 2 = ¨r⊥(s) 2 + ¨r||(s) 2 can be dropped
¨r(s) ≈ ¨r⊥(s). (2.30)
This leads to the bending energy Hamiltonian in the weakly-bending rod approximation5
Hwb[r⊥(s)] = κ
2
L
0
ds ¨r⊥(s) 2 = κ
2
L
0
ds ¨x(s) 2 + ¨y(s) 2 . (2.31)
Now the inextensibility constraint is implicitly, i.e. automatically, fulfilled. The
path-integral for the partition sum, eq. (2.19), has been simplified, since the
inextensibility constraint in weakly-bending rod approximation leaves only two
independent components.
This approximation is also called Monge-representation to indicate the chosen
parametrization. The single valued contour is parametrized in reference to a
reference line by the undulations 6 .
2.5 Real chains
We have dealt so far with idealized chains represented by segments or a continuous
space curve, allowed to interpenetrate each other. Therefore these models are
5In classical elasticity theory the energy of a weakly-bending rod has exactly the same form
as this Hamiltonian (cf. [23]).
6In differential geometry the Monge-representation is used to parametrize single-valued
planes by a ‘hight’ over a reference plane.