Wave Propagation in Linear Media | re-examined
Wave Propagation in Linear Media | re-examined
Wave Propagation in Linear Media | re-examined
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
3.4 A simple thought experiment<br />
I0<br />
Z0<br />
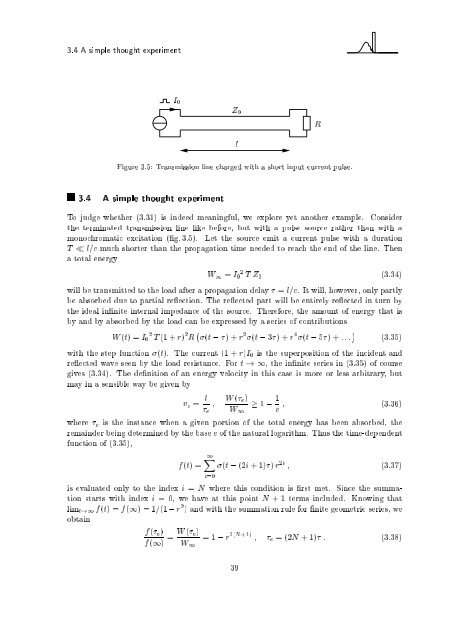
Figu<strong>re</strong> 3.5: Transmission l<strong>in</strong>e charged with a short <strong>in</strong>put cur<strong>re</strong>nt pulse.<br />
3.4 A simple thought experiment<br />
To judge whether (3.31) is <strong>in</strong>deed mean<strong>in</strong>gful, we explo<strong>re</strong> yet another example. Consider<br />
the term<strong>in</strong>ated transmission l<strong>in</strong>e like befo<strong>re</strong>, but with a pulse source rather than with a<br />
monochromatic excitation ( g. 3.5). Let the source emit a cur<strong>re</strong>nt pulse with a duration<br />
T l=c much shorter than the propagation time needed to <strong>re</strong>ach the end of the l<strong>in</strong>e. Then<br />
a total energy<br />
W1 = I0 2 TZ0<br />
l<br />
R<br />
(3.34)<br />
will be transmitted to the load after a propagation delay = l=c. It will, however, only partly<br />
be absorbed due to partial <strong>re</strong> ection. The <strong>re</strong> ected part will be enti<strong>re</strong>ly <strong>re</strong> ected <strong>in</strong> turn by<br />
the ideal <strong>in</strong> nite <strong>in</strong>ternal impedance of the source. The<strong>re</strong>fo<strong>re</strong>, the amount of energy that is<br />
by and by absorbed by the load can be exp<strong>re</strong>ssed by a series of contributions<br />
W (t) =I0 2 T(1 + r) 2 R , (t , )+r 2 (t,3 )+r 4 (t,5 )+::: (3.35)<br />
with the step function (t). The cur<strong>re</strong>nt (1 + r)I0 is the superposition of the <strong>in</strong>cident and<br />
<strong>re</strong> ected wave seen by the load <strong>re</strong>sistance. For t !1,the <strong>in</strong> nite series <strong>in</strong> (3.35) of course<br />
gives (3.34). The de nition of an energy velocity <strong>in</strong> this case is mo<strong>re</strong> or less arbitrary, but<br />
may <strong>in</strong> a sensible way be given by<br />
ve = l<br />
e<br />
; W ( e)<br />
W1<br />
1 , 1<br />
; (3.36)<br />
e<br />
whe<strong>re</strong> e is the <strong>in</strong>stance when a given portion of the total energy has been absorbed, the<br />
<strong>re</strong>ma<strong>in</strong>der be<strong>in</strong>g determ<strong>in</strong>ed by the base e of the natural logarithm. Thus the time-dependent<br />
function of (3.35),<br />
f(t) =<br />
1X<br />
i=0<br />
(t , (2i +1) )r 2i ; (3.37)<br />
is evaluated only to the <strong>in</strong>dex i = N whe<strong>re</strong> this condition is rst met. S<strong>in</strong>ce the summation<br />
starts with <strong>in</strong>dex i = 0, we have at this po<strong>in</strong>t N +1 terms <strong>in</strong>cluded. Know<strong>in</strong>g that<br />
limt!1 f(t) =f(1)=1=(1 , r 2 ) and with the summation rule for nite geometric series, we<br />
obta<strong>in</strong><br />
f( e)<br />
f(1) = W ( e)<br />
=1,r<br />
W1<br />
2(N+1) ; e =(2N+1) : (3.38)<br />
39