1. magnetic confinement - ENEA - Fusione
1. magnetic confinement - ENEA - Fusione
1. magnetic confinement - ENEA - Fusione
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
<strong>1.</strong> MAGNETIC CONFINEMENT<br />
47<br />
<strong>1.</strong>3 Plasma Theory<br />
1<br />
0.8<br />
0.6<br />
0.4<br />
0.2<br />
(m-1,n)<br />
non-local<br />
continuum damping<br />
(m,n)<br />
0<br />
-2 -<strong>1.</strong>5 -1 -0.5 0 0.5 1 <strong>1.</strong>5<br />
x<br />
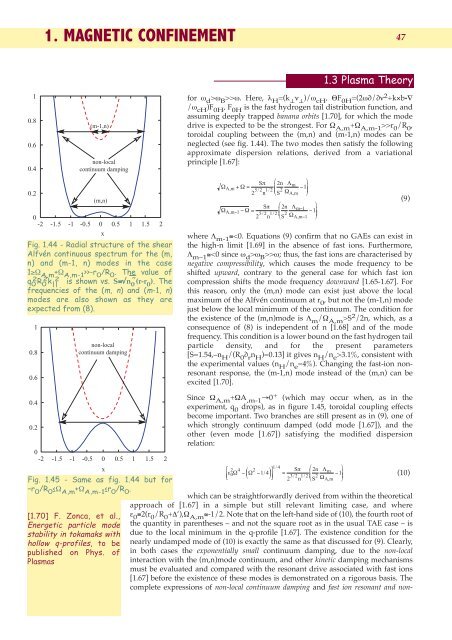
Fig. <strong>1.</strong>44 - Radial structure Fig <strong>1.</strong>44 of the shear<br />
Alfvén continuous spectrum for the (m,<br />
n) and (m-1, n) modes in the case<br />
1≥Ω A,m +Ω A,m-1 >>-r 0 /R 0 . The value of<br />
q 2 0 R 2 0 k 2<br />
|| is shown vs. S≡√n ” 0 (r-r 0 ). The<br />
frequencies of the (m, n) and (m-1, n)<br />
modes are also shown as they are<br />
expected from (8).<br />
1<br />
0.8<br />
0.6<br />
non-local<br />
continuum damping<br />
2<br />
for ω d >ω B >>ω. Here, λ H =(k ⊥ ν ⊥ )/ω cH , ΘF 0H =(2ω∂/∂ν 2 +k×b•∇<br />
/ω cH )F 0H , F 0H is the fast hydrogen tail distribution function, and<br />
assuming deeply trapped banana orbits [<strong>1.</strong>70], for which the mode<br />
drive is expected to be the strongest. For Ω A,m +Ω A,m-1 >>r 0 /R 0 ,<br />
toroidal coupling between the (m,n) and (m-1,n) modes can be<br />
neglected (see fig. <strong>1.</strong>44). The two modes then satisfy the following<br />
approximate dispersion relations, derived from a variational<br />
principle [<strong>1.</strong>67]:<br />
Sπ<br />
⎛<br />
2n<br />
Λ<br />
⎞<br />
ΩAm<br />
Ω<br />
m<br />
, + = −<br />
/ / ⎜<br />
1<br />
52 12 2<br />
n ⎝S<br />
Ω ⎟<br />
2<br />
Am , ⎠<br />
Sπ<br />
⎛<br />
2n<br />
Λ<br />
⎞<br />
ΩAm<br />
Ω<br />
m<br />
, − = −1<br />
−1 −<br />
/ / ⎜<br />
1<br />
52 12 2<br />
n ⎝S<br />
Ω ⎟<br />
2<br />
Am , −1<br />
⎠<br />
where Λ m-1 ≅>ω; thus, the fast ions are characterised by<br />
negative compressibility, which causes the mode frequency to be<br />
shifted upward, contrary to the general case for which fast ion<br />
compression shifts the mode frequency downward [<strong>1.</strong>65-<strong>1.</strong>67]. For<br />
this reason, only the (m,n) mode can exist just above the local<br />
maximum of the Alfvén continuum at r 0 , but not the (m-1,n) mode<br />
just below the local minimum of the continuum. The condition for<br />
the existence of the (m,n)mode is Λ m /Ω A,m >S 2 /2n, which, as a<br />
consequence of (8) is independent of n [<strong>1.</strong>68] and of the mode<br />
frequency. This condition is a lower bound on the fast hydrogen tail<br />
particle density, and for the present parameters<br />
[S=<strong>1.</strong>54,–n H /(R 0 ∂ r n H )=0.13] it gives n H /n e >3.1%, consistent with<br />
the experimental values (n H /n e =4%). Changing the fast-ion nonresonant<br />
response, the (m-1,n) mode instead of the (m,n) can be<br />
excited [<strong>1.</strong>70].<br />
(9)<br />
0.4<br />
0.2<br />
0<br />
-2 -<strong>1.</strong>5 -1 -0.5 0 0.5 1 <strong>1.</strong>5<br />
x<br />
Fig. <strong>1.</strong>45 - Same as fig. <strong>1.</strong>44 but for<br />
Fig <strong>1.</strong>45<br />
–r 0 /R 0 ≤Ω A,m +Ω A,m-1 ≤r 0 /R 0 .<br />
[<strong>1.</strong>70] F. Zonca, et al.,<br />
Energetic particle mode<br />
stability in tokamaks with<br />
hollow q-profiles, to be<br />
published on Phys. of<br />
Plasmas<br />
2<br />
Since Ω A,m +ΩA ,m-1 →0 + (which may occur when, as in the<br />
experiment, q 0 drops), as in figure <strong>1.</strong>45, toroidal coupling effects<br />
become important. Two branches are still present as in (9), one of<br />
which strongly continuum damped (odd mode [<strong>1.</strong>67]), and the<br />
other (even mode [<strong>1.</strong>67]) satisfying the modified dispersion<br />
relation:<br />
which can be straightforwardly derived from within the theoretical<br />
approach of [<strong>1.</strong>67] in a simple but still relevant limiting case, and where<br />
ε 0 ≡2(r 0 /R 0 +∆’),Ω A,m ≅-1/2. Note that on the left-hand side of (10), the fourth root of<br />
the quantity in parentheses – and not the square root as in the usual TAE case – is<br />
due to the local minimum in the q-profile [<strong>1.</strong>67]. The existence condition for the<br />
nearly undamped mode of (10) is exactly the same as that discussed for (9). Clearly,<br />
in both cases the exponentially small continuum damping, due to the non-local<br />
interaction with the (m,n)mode continuum, and other kinetic damping mechanisms<br />
must be evaluated and compared with the resonant drive associated with fast ions<br />
[<strong>1.</strong>67] before the existence of these modes is demonstrated on a rigorous basis. The<br />
complete expressions of non-local continuum damping and fast ion resonant and non-<br />
⎡<br />
π<br />
ε0<br />
2 4 2 14<br />
⎛<br />
2 Λ<br />
Ω −⎜<br />
⎞⎤<br />
/<br />
S<br />
⎛<br />
n<br />
⎞<br />
Ω −14<br />
/ ⎟ = m −1<br />
⎣⎢ ⎝ ⎠⎦⎥ 32 / 12 / ⎜ 2<br />
2 ⎝ Ω ⎟<br />
n S Am , ⎠<br />
(10)