Journal of Mechanics of Materials and Structures vol. 5 (2010 ... - MSP

726 BAHATTIN KILIC AND ERDOGAN MADENCI
where � + and � − are the volumes in which integration is performed and are defined to be the right and
left sides of the x-plane (Figure A1). The subscript x indicates the x- component of the resulting volume
integration. For a point, x embedded in a single material, the value of gx(x) will become 2.
Moreover, by applying the displacement gradient in the y-direction, the force density, gy(x), can
written as
� �
gy(x) =
� +
dV ′ f (u ′ , u, x ′ �
, x) −
�− dV ′ f (u ′ , u, x ′ �
, x) , (A3)
y
where � + and � − are the volumes in which integration is performed; they are defined to be the upper
and lower sides of the y-plane (Figure A1). The force densities, gx(x) and gy(x), are clearly different
due to dissimilar volumes of integrations. Hence, the domain under uniaxial tension exhibits directional
dependence. In other words, depending on the direction of uniaxial tension, the points closer to the free
surfaces or interfaces experience different force densities, which is not physically acceptable. Therefore,
the uniaxial tension is also applied in the y- and z-directions, which leads to three different responses at
each integration point and can be expressed in vector form
g T (x) = � �
gx gy gz , (A4)
in which x, y, and z represent the directions of applied uniaxial tension.
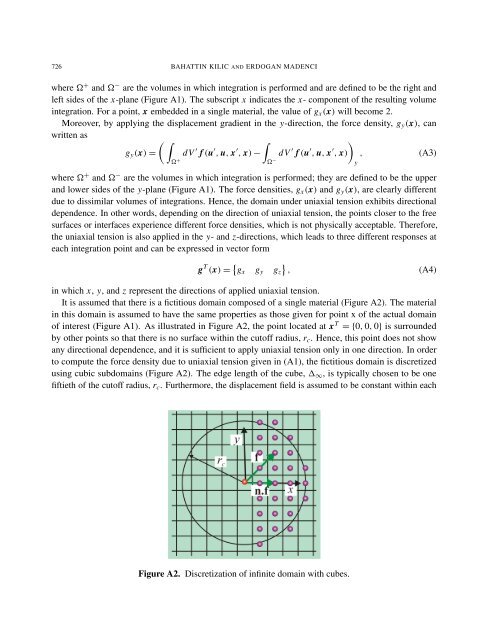
It is assumed that there is a fictitious domain composed of a single material (Figure A2). The material
in this domain is assumed to have the same properties as those given for point x of the actual domain
of interest (Figure A1). As illustrated in Figure A2, the point located at x T = {0, 0, 0} is surrounded
by other points so that there is no surface within the cutoff radius, rc. Hence, this point does not show
any directional dependence, and it is sufficient to apply uniaxial tension only in one direction. In order
to compute the force density due to uniaxial tension given in (A1), the fictitious domain is discretized
using cubic subdomains (Figure A2). The edge length of the cube, �∞, is typically chosen to be one
fiftieth of the cutoff radius, rc. Furthermore, the displacement field is assumed to be constant within each
Figure A2. Discretization of infinite domain with cubes.