Journal of Mechanics of Materials and Structures vol. 5 (2010 ... - MSP

760 XIAO-TING HE, QIANG CHEN, JUN-YI SUN, ZHOU-LIAN ZHENG AND SHAN-LIN CHEN
From (11), we obtain
t1 =
E + t 2 1
1 − µ + = E−t 2 2
.
1 − µ −
(13)
Combining t1 + t2 = t, we solve for the thicknesses of the plate in tension and compression as follows:
�
E− (1 − µ + )
� � t,
E + (1 − µ − ) + E− (1 − µ + )
�
E + (1 − µ − )
t2 = � � t.
E + (1 − µ − ) + E− (1 − µ + )
(14)
Thus, the position of the unknown neutral layer of the plate in pure bending is finally determined analytically.
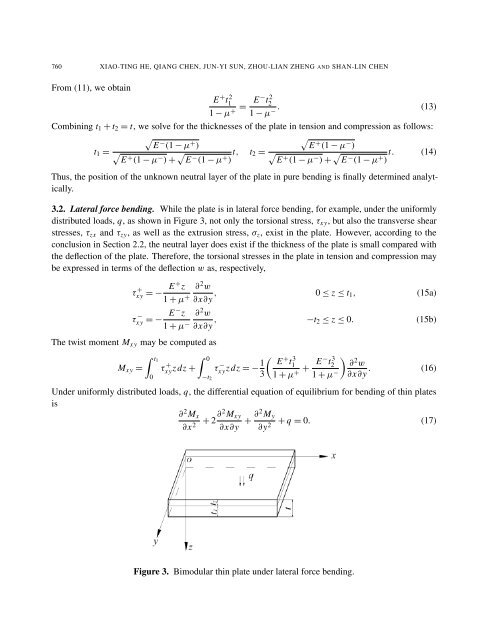
3.2. Lateral force bending. While the plate is in lateral force bending, for example, under the uniformly
distributed loads, q, as shown in Figure 3, not only the torsional stress, τxy, but also the transverse shear
stresses, τzx and τzy, as well as the extrusion stress, σz, exist in the plate. However, according to the
conclusion in Section 2.2, the neutral layer does exist if the thickness of the plate is small compared with
the deflection of the plate. Therefore, the torsional stresses in the plate in tension and compression may
be expressed in terms of the deflection w as, respectively,
τ + xy = − E+ z
1 + µ +
∂2w ∂x∂y , 0 ≤ z ≤ t1, (15a)
τ − xy = − E−z 1 + µ −
∂2w ∂x∂y , −t2 ≤ z ≤ 0. (15b)
The twist moment Mxy may be computed as
� t1
Mxy = τ
0
+ � 0
xy z dz + τ
−t2
− �
E + 3 t1 xy z dz = −1
3 1 + µ + + E−t 3 2
1 + µ −
�
∂2w . (16)
∂x∂y
Under uniformly distributed loads, q, the differential equation of equilibrium for bending of thin plates
is
∂2 Mx
∂x 2 + 2∂2 Mxy
∂x∂y + ∂2 My
+ q = 0. (17)
∂y 2
Figure 3. Bimodular thin plate under lateral force bending.