Physical Principles of Electron Microscopy: An Introduction to TEM ...
Physical Principles of Electron Microscopy: An Introduction to TEM ...
Physical Principles of Electron Microscopy: An Introduction to TEM ...
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
156 Chapter 6<br />
where h = 6.63 � 10 -34 Js is the Planck constant. Because the left-hand side<br />
<strong>of</strong> Eq. (6.2) represents the angular momentum <strong>of</strong> the electron and because n<br />
is any integer, known as the (principal) quantum number, Eq. (6.2)<br />
represents the quantization <strong>of</strong> angular momentum. Without this condition,<br />
the a<strong>to</strong>m is unstable: the centripetal acceleration <strong>of</strong> the electron would cause<br />
it <strong>to</strong> emit electromagnetic radiation and quickly spiral in<strong>to</strong> the nucleus. This<br />
problem does not arise in connection with planets in the solar system<br />
because<br />
they are electrically neutral.<br />
Using Eq. (6.2) <strong>to</strong> substitute for v in Eq. (6.1) provides the radius rn <strong>of</strong><br />
the<br />
orbit <strong>of</strong> quantum number n :<br />
rn = n 2 (h/2�) 2 /(KmZe 2 ) = n 2 a0 /Z (6.3)<br />
Here, a0 = 0.053 nm is the (first) Bohr radius, corresponding <strong>to</strong> the radius <strong>of</strong><br />
a hydrogen a<strong>to</strong>m (Z = 1) in its lowest-energy ground state (n = 1). We can<br />
now use Eq. (6.2) <strong>to</strong> solve for the orbital speed vn or, more usefully, employ<br />
Eq. (6.1) <strong>to</strong> calculate the <strong>to</strong>tal energy En <strong>of</strong> an orbiting electron as the sum <strong>of</strong><br />
its<br />
kinetic and potential energies:<br />
En = mv 2 /2 � K(Ze)(e)/rn = KZe 2 /(2rn) � KZe 2 /rn<br />
= � K Z e 2 /(2rn) = � R (Z 2 /n 2 ) (6.4)<br />
Here, R = Ke 2 /(2a0) = 13.6 eV is the Rydberg energy, the energy needed <strong>to</strong><br />
remove the electron from a hydrogen a<strong>to</strong>m (Z 2 = 1) in its ground state (n = 1)<br />
and therefore equal <strong>to</strong> the ionization energy <strong>of</strong> hydrogen.<br />
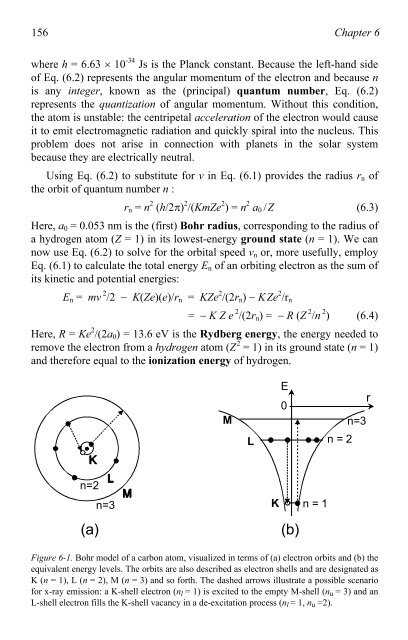
n=2<br />
n=3<br />
(a) (b)<br />
M<br />
L<br />
E<br />
0<br />
K n = 1<br />
n=3<br />
n = 2<br />
Figure 6-1. Bohr model <strong>of</strong> a carbon a<strong>to</strong>m, visualized in terms <strong>of</strong> (a) electron orbits and (b) the<br />
equivalent energy levels. The orbits are also described as electron shells and are designated as<br />
K (n = 1), L (n = 2), M (n = 3) and so forth. The dashed arrows illustrate a possible scenario<br />
for x-ray emission: a K-shell electron (nl = 1) is excited <strong>to</strong> the empty M-shell (nu = 3) and an<br />
L-shell electron fills the K-shell vacancy in a de-excitation process (nl = 1, nu =2).<br />
r