DIFFERENTIAL GEOMETRY: A First Course in Curves and Surfaces

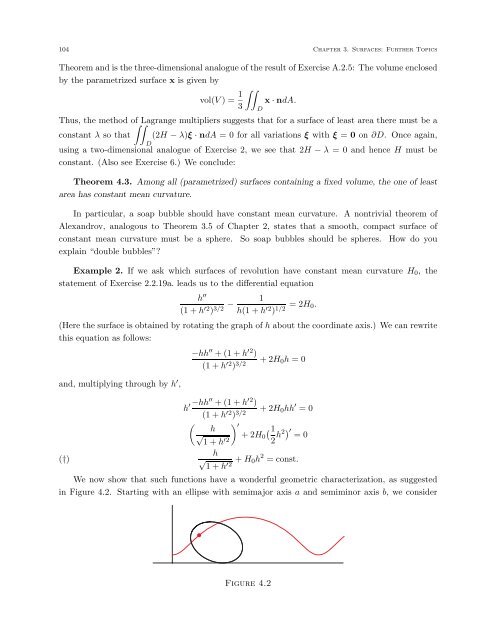
104 Chapter 3. Surfaces: Further TopicsTheorem and is the three-dimensional analogue of the result of Exercise A.2.5: The volume enclosedby the parametrized surface x is given byvol(V )= 1 ∫∫x · ndA.3 DThus, the method of ∫∫Lagrange multipliers suggests that for a surface of least area there must be aconstant λ so that (2H − λ)ξ · ndA =0for all variations ξ with ξ = 0 on ∂D. Once again,Dusing a two-dimensional analogue of Exercise 2, we see that 2H − λ =0and hence H must beconstant. (Also see Exercise 6.) We conclude:Theorem 4.3. Among all (parametrized) surfaces containing a fixed volume, the one of leastarea has constant mean curvature.In particular, a soap bubble should have constant mean curvature. A nontrivial theorem ofAlexandrov, analogous to Theorem 3.5 of Chapter 2, states that a smooth, compact surface ofconstant mean curvature must be a sphere. So soap bubbles should be spheres. How do youexplain “double bubbles”?Example 2. If we ask which surfaces of revolution have constant mean curvature H 0 , thestatement of Exercise 2.2.19a. leads us to the differential equationh ′′(1 + h ′2 ) 3/2 − 1h(1 + h ′2 ) 1/2 =2H 0.(Here the surface is obtained by rotating the graph of h about the coordinate axis.) We can rewritethis equation as follows:and, multiplying through by h ′ ,−hh ′′ +(1+h ′2 )(1 + h ′2 ) 3/2 +2H 0 h =0(†)h ′ −hh′′ +(1+h ′2 )(1 + h ′2 ) 3/2 +2H 0 hh ′ =0( )h ′(1√ +2H 01+h ′2 2 h2) ′ =0h√ + H 0h 2 = const.1+h ′2We now show that such functions have a wonderful geometric characterization, as suggestedin Figure 4.2. Starting with an ellipse with semimajor axis a and semiminor axis b, weconsiderFigure 4.2