DIFFERENTIAL GEOMETRY: A First Course in Curves and Surfaces

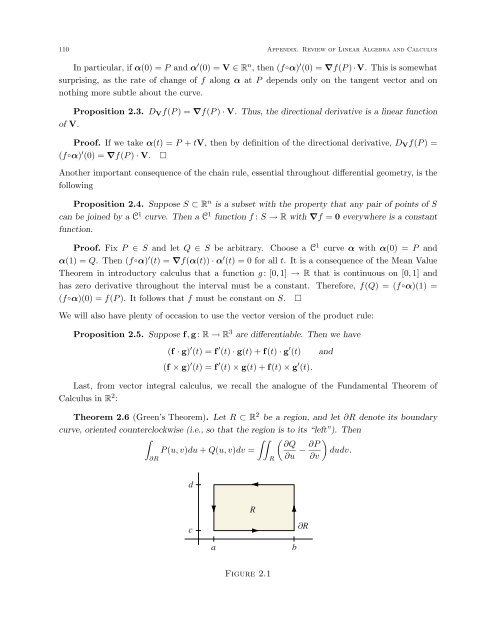
110 Appendix. Review of Linear Algebra and CalculusIn particular, if α(0) = P and α ′ (0) = V ∈ R n , then (f ◦α) ′ (0) = ∇f(P ) · V. This is somewhatsurprising, as the rate of change of f along α at P depends only on the tangent vector and onnothing more subtle about the curve.Proposition 2.3. D V f(P )=∇f(P ) · V. Thus, the directional derivative is a linear functionof V.Proof. If we take α(t) =P + tV, then by definition of the directional derivative, D V f(P )=(f ◦α) ′ (0) = ∇f(P ) · V. □Another important consequence of the chain rule, essential throughout differential geometry, is thefollowingProposition 2.4. Suppose S ⊂ R n is a subset with the property that any pair of points of Scan be joined by a C 1 curve. Then a C 1 function f : S → R with ∇f = 0 everywhere is a constantfunction.Proof. Fix P ∈ S and let Q ∈ S be arbitrary. Choose a C 1 curve α with α(0) = P andα(1) = Q. Then (f ◦α) ′ (t) =∇f(α(t)) · α ′ (t) =0for all t. Itisaconsequence of the Mean ValueTheorem in introductory calculus that a function g :[0, 1] → R that is continuous on [0, 1] andhas zero derivative throughout the interval must be a constant. Therefore, f(Q) =(f ◦α)(1) =(f ◦α)(0) = f(P ). It follows that f must be constant on S. □We will also have plenty of occasion to use the vector version of the product rule:Proposition 2.5. Suppose f, g : R → R 3 are differentiable. Then we have(f · g) ′ (t) =f ′ (t) · g(t)+f(t) · g ′ (t) and(f × g) ′ (t) =f ′ (t) × g(t)+f(t) × g ′ (t).Last, from vector integral calculus, we recall the analogue of the Fundamental Theorem ofCalculus in R 2 :Theorem 2.6 (Green’s Theorem). Let R ⊂ R 2 be a region, and let ∂R denote its boundarycurve, oriented counterclockwise (i.e., so that the region is to its “left”). Then∫∫∫ ( ∂QP (u, v)du + Q(u, v)dv =∂u − ∂P )dudv.∂v∂RRdcR∂RabFigure 2.1