DIFFERENTIAL GEOMETRY: A First Course in Curves and Surfaces

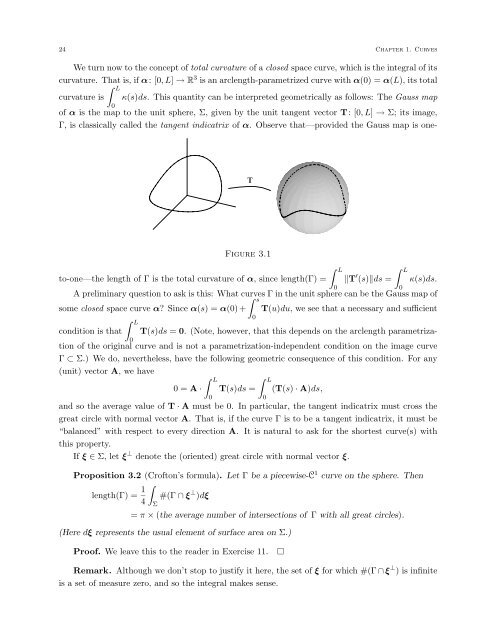
24 Chapter 1. CurvesWe turn now to the concept of total curvature of a closed space curve, which is the integral of itscurvature. That is, if α: [0,L] → R 3 is an arclength-parametrized curve with α(0) = α(L), its totalcurvature is∫ L0κ(s)ds. This quantity can be interpreted geometrically as follows: The Gauss mapof α is the map to the unit sphere, Σ, given by the unit tangent vector T: [0,L] → Σ; its image,Γ, is classically called the tangent indicatrix of α. Observe that—provided the Gauss map is one-TFigure 3.1to-one—the length of Γ is the total curvature of α, since length(Γ) =∫ L0‖T ′ (s)‖ds =∫ L0κ(s)ds.A preliminary question to ask is this: What curves Γ in the unit sphere can be the Gauss map ofsome closed space curve α? Since α(s) =α(0) +condition is that∫ L0∫ s0T(u)du, wesee that a necessary and sufficientT(s)ds = 0. (Note, however, that this depends on the arclength parametrizationof the original curve and is not a parametrization-independent condition on the image curveΓ ⊂ Σ.) We do, nevertheless, have the following geometric consequence of this condition. For any(unit) vector A, wehave0=A ·∫ L0T(s)ds =∫ L0(T(s) · A)ds,and so the average value of T · A must be 0. In particular, the tangent indicatrix must cross thegreat circle with normal vector A. That is, if the curve Γ is to be a tangent indicatrix, it must be“balanced” with respect to every direction A. Itisnatural to ask for the shortest curve(s) withthis property.If ξ ∈ Σ, let ξ ⊥ denote the (oriented) great circle with normal vector ξ.Proposition 3.2 (Crofton’s formula). Let Γ be apiecewise-C 1 curve on the sphere. Thenlength(Γ) = 1 ∫#(Γ ∩ ξ ⊥ )dξ4 Σ= π × (the average number of intersections of Γ with all great circles).(Here dξ represents the usual element of surface area on Σ.)Proof. We leave this to the reader in Exercise 11.□Remark. Although we don’t stop to justify it here, the set of ξ for which #(Γ ∩ ξ ⊥ )isinfiniteis a set of measure zero, and so the integral makes sense.