DIFFERENTIAL GEOMETRY: A First Course in Curves and Surfaces

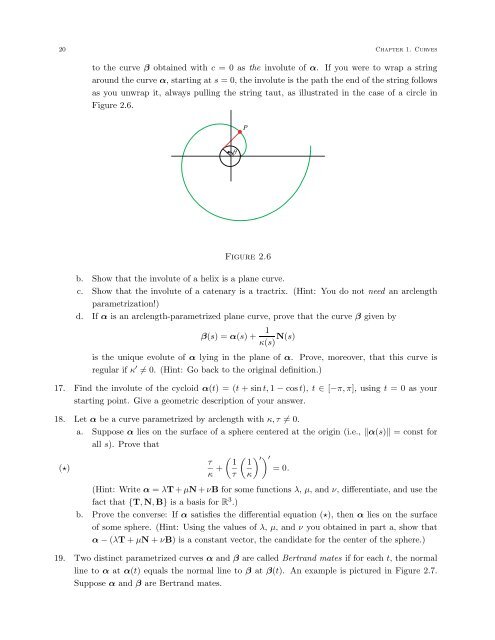
20 Chapter 1. Curvesto the curve β obtained with c =0asthe involute of α. Ifyou were to wrap a stringaround the curve α, starting at s =0,the involute is the path the end of the string followsas you unwrap it, always pulling the string taut, as illustrated in the case of a circle inFigure 2.6.PθFigure 2.6b. Show that the involute of a helix is a plane curve.c. Show that the involute of a catenary is a tractrix. (Hint: You do not need an arclengthparametrization!)d. If α is an arclength-parametrized plane curve, prove that the curve β given byβ(s) =α(s)+ 1κ(s) N(s)is the unique evolute of α lying in the plane of α. Prove, moreover, that this curve isregular if κ ′ ≠0. (Hint: Go back to the original definition.)17. Find the involute of the cycloid α(t) =(t + sin t, 1 − cos t), t ∈ [−π, π], using t =0asyourstarting point. Give a geometric description of your answer.18. Let α be a curve parametrized by arclength with κ, τ ≠0.a. Suppose α lies on the surface of a sphere centered at the origin (i.e., ‖α(s)‖ = const forall s). Prove that(⋆)( ( )τ 1 1 ′ ) ′κ + =0.τ κ(Hint: Write α = λT + µN + νB for some functions λ, µ, and ν, differentiate, and use thefact that {T, N, B} is a basis for R 3 .)b. Prove the converse: If α satisfies the differential equation (⋆), then α lies on the surfaceof some sphere. (Hint: Using the values of λ, µ, and ν you obtained in part a, show thatα − (λT + µN + νB) isaconstant vector, the candidate for the center of the sphere.)19. Two distinct parametrized curves α and β are called Bertrand mates if for each t, the normalline to α at α(t) equals the normal line to β at β(t). An example is pictured in Figure 2.7.Suppose α and β are Bertrand mates.