DIFFERENTIAL GEOMETRY: A First Course in Curves and Surfaces

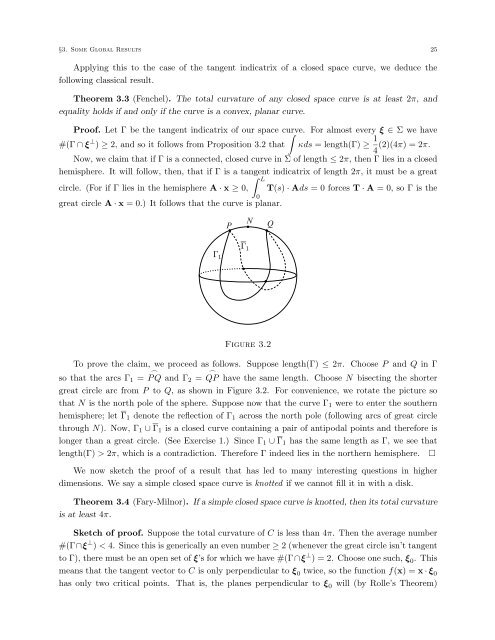
§3. Some Global Results 25Applying this to the case of the tangent indicatrix of a closed space curve, we deduce thefollowing classical result.Theorem 3.3 (Fenchel). The total curvature of any closed space curve is at least 2π, andequality holds if and only if the curve is a convex, planar curve.Proof. Let Γ be the tangent indicatrix of our space curve. ∫ For almost every ξ ∈ Σwehave#(Γ ∩ ξ ⊥ ) ≥ 2, and so it follows from Proposition 3.2 that κds = length(Γ) ≥ 1 (2)(4π) =2π.4Now, we claim that if Γ is a connected, closed curve in Σ of length ≤ 2π, then Γ lies in a closedhemisphere. It will follow, then, that if Γ is a tangent indicatrix of length 2π, itmust be a greatcircle. (For if Γ lies in the hemisphere A · x ≥ 0,∫ Lgreat circle A · x = 0.) It follows that the curve is planar.0T(s) · Ads =0forces T · A =0,soΓis thePNQΓ 1Γ 1Figure 3.2To prove the claim, we proceed as follows. Suppose length(Γ) ≤ 2π. Choose P and Q in Γso that the arcs Γ 1 = PQ ⌢and Γ 2 = QP ⌢have the same length. Choose N bisecting the shortergreat circle arc from P to Q, asshown in Figure 3.2. For convenience, we rotate the picture sothat N is the north pole of the sphere. Suppose now that the curve Γ 1 were to enter the southernhemisphere; let Γ 1 denote the reflection of Γ 1 across the north pole (following arcs of great circlethrough N). Now, Γ 1 ∪ Γ 1 is a closed curve containing a pair of antipodal points and therefore islonger than a great circle. (See Exercise 1.) Since Γ 1 ∪ Γ 1 has the same length as Γ, we see thatlength(Γ) > 2π, which is a contradiction. Therefore Γ indeed lies in the northern hemisphere. □We now sketch the proof of a result that has led to many interesting questions in higherdimensions. We say a simple closed space curve is knotted if we cannot fill it in with a disk.Theorem 3.4 (Fary-Milnor). If a simple closed space curve is knotted, then its total curvatureis at least 4π.Sketch of proof. Suppose the total curvature of C is less than 4π. Then the average number#(Γ∩ξ ⊥ ) < 4. Since this is generically an even number ≥ 2 (whenever the great circle isn’t tangentto Γ), there must be an open set of ξ’s for which we have #(Γ∩ξ ⊥ )=2. Choose one such, ξ 0 . Thismeans that the tangent vector to C is only perpendicular to ξ 0 twice, so the function f(x) =x · ξ 0has only two critical points. That is, the planes perpendicular to ξ 0 will (by Rolle’s Theorem)