DIFFERENTIAL GEOMETRY: A First Course in Curves and Surfaces

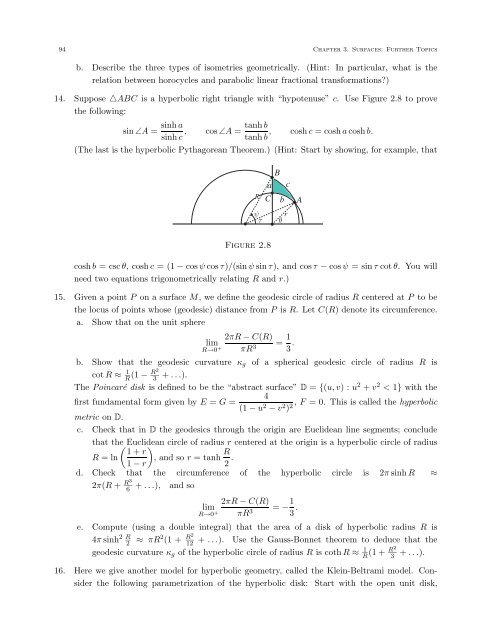
94 Chapter 3. Surfaces: Further Topicsb. Describe the three types of isometries geometrically. (Hint: In particular, what is therelation between horocycles and parabolic linear fractional transformations?)14. Suppose △ABC is a hyperbolic right triangle with “hypotenuse” c. Use Figure 2.8 to provethe following:sin ∠A = sinh asinh c ,tanh bcos ∠A = , cosh c = cosh a cosh b.tanh b(The last is the hyperbolic Pythagorean Theorem.) (Hint: Start by showing, for example, thatRψτBa cC brθAFigure 2.8cosh b = csc θ, cosh c =(1− cos ψ cos τ)/(sin ψ sin τ), and cos τ − cos ψ = sin τ cot θ. You willneed two equations trigonometrically relating R and r.)15. Given a point P on a surface M, wedefine the geodesic circle of radius R centered at P to bethe locus of points whose (geodesic) distance from P is R. Let C(R) denote its circumference.a. Show that on the unit sphere2πR − C(R)limR→0 + πR 3 = 1 3 .b. Show that the geodesic curvature κ g of a spherical geodesic circle of radius R iscot R ≈ 1 R2R(1 −3 + ...).The Poincaré disk is defined to be the “abstract surface” D = {(u, v) :u 2 + v 2 < 1} with the4first fundamental form given by E = G =(1 − u 2 − v 2 , F =0. This is called the hyperbolic) 2metric on D.c. Check that in D the geodesics through the origin are Euclidean line segments; concludethat the ( Euclidean ) circle of radius r centered at the origin is a hyperbolic circle of radius1+rR =ln , and so r = tanh R 1 − r2 .d. Check that the circumference of the hyperbolic circle is 2π sinh R ≈2π(R + R36 + ...), and so 2πR − C(R)limR→0 + πR 3 = − 1 3 .e. Compute (using a double integral) that the area of a disk of hyperbolic radius R is4π sinh 2 R 2≈ πR2 (1 + R212+ ...). Use the Gauss-Bonnet theorem to deduce that thegeodesic curvature κ g of the hyperbolic circle of radius R is coth R ≈ 1 R2R(1 +3 + ...).16. Here we give another model for hyperbolic geometry, called the Klein-Beltrami model. Considerthe following parametrization of the hyperbolic disk: Start with the open unit disk,