DIFFERENTIAL GEOMETRY: A First Course in Curves and Surfaces

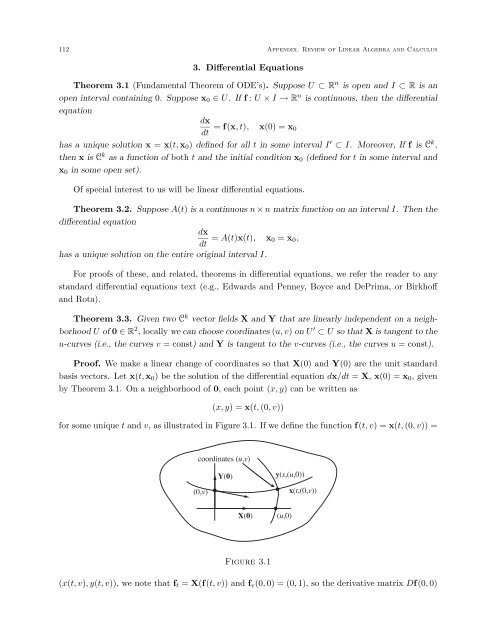
112 Appendix. Review of Linear Algebra and Calculus3. Differential EquationsTheorem 3.1 (Fundamental Theorem of ODE’s). Suppose U ⊂ R n is open and I ⊂ R is anopen interval containing 0. Suppose x 0 ∈ U. Iff : U × I → R n is continuous, then the differentialequationdxdt = f(x,t), x(0) = x 0has a unique solution x = x(t, x 0 ) defined for all t in some interval I ′ ⊂ I. Moreover, If f is C k ,then x is C k as a function of both t and the initial condition x 0 (defined for t in some interval andx 0 in some open set).Of special interest to us will be linear differential equations.Theorem 3.2. Suppose A(t) is a continuous n × n matrix function on an interval I. Then thedifferential equationdxdt = A(t)x(t), x 0 = x 0 ,has a unique solution on the entire original interval I.For proofs of these, and related, theorems in differential equations, we refer the reader to anystandard differential equations text (e.g., Edwards and Penney, Boyce and DePrima, or Birkhoffand Rota).Theorem 3.3. Given two C k vector fields X and Y that are linearly independent on a neighborhoodU of 0 ∈ R 2 , locally we can choose coordinates (u, v) on U ′ ⊂ U so that X is tangent to theu-curves (i.e., the curves v = const) and Y is tangent to the v-curves (i.e., the curves u = const).Proof. We make a linear change of coordinates so that X(0) and Y(0) are the unit standardbasis vectors. Let x(t, x 0 )bethe solution of the differential equation dx/dt = X, x(0) = x 0 , givenby Theorem 3.1. On a neighborhood of 0, each point (x, y) can be written as(x, y) =x(t, (0,v))for some unique t and v, asillustrated in Figure 3.1. If we define the function f(t, v) =x(t, (0,v)) =coordinates (u,v)(0,v)Y(0)y(s,(u,0))x(t,(0,v))X(0)(u,0)Figure 3.1(x(t, v),y(t, v)), we note that f t = X(f(t, v)) and f v (0, 0) =(0, 1), so the derivative matrix Df(0, 0)