DIFFERENTIAL GEOMETRY: A First Course in Curves and Surfaces

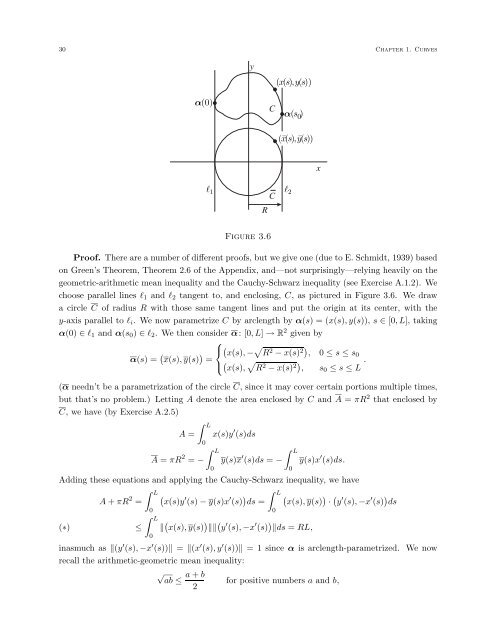
30 Chapter 1. Curvesy( x(s) ,y(s))α(0)Cα(s 0 )( x(s),y(s) )xl 1 l 2CRFigure 3.6Proof. There are a number of different proofs, but we give one (due to E. Schmidt, 1939) basedon Green’s Theorem, Theorem 2.6 of the Appendix, and—not surprisingly—relying heavily on thegeometric-arithmetic mean inequality and the Cauchy-Schwarz inequality (see Exercise A.1.2). Wechoose parallel lines l 1 and l 2 tangent to, and enclosing, C, aspictured in Figure 3.6. We drawa circle C of radius R with those same tangent lines and put the origin at its center, with they-axis parallel to l i .Wenow parametrize C by arclength by α(s) =(x(s),y(s)), s ∈ [0,L], takingα(0) ∈ l 1 and α(s 0 ) ∈ l 2 .Wethen consider α: [0,L] → R 2 given by⎧α(s) = ( x(s), y(s) ) ⎨( √x(s), − R 2 − x(s) 2) , 0 ≤ s ≤ s 0= ( √⎩ x(s), R 2 − x(s) 2) , s 0 ≤ s ≤ L .(α needn’t be a parametrization of the circle C, since it may cover certain portions multiple times,but that’s no problem.) Letting A denote the area enclosed by C and A = πR 2 that enclosed byC, wehave (by Exercise A.2.5)A =∫ LA = πR 2 = −0∫ L0x(s)y ′ (s)dsy(s)x ′ (s)ds = −∫ L0y(s)x ′ (s)ds.Adding these equations and applying the Cauchy-Schwarz inequality, we have∫ LA + πR 2 (= x(s)y ′ (s) − y(s)x ′ (s) ) ∫ L ( ) (ds = x(s), y(s) · y ′ (s), −x ′ (s) ) ds(∗)≤0∫ L0‖ ( x(s), y(s) ) ‖‖ ( y ′ (s), −x ′ (s) ) ‖ds = RL,inasmuch as ‖(y ′ (s), −x ′ (s))‖ = ‖(x ′ (s),y ′ (s))‖ =1since α is arclength-parametrized. We nowrecall the arithmetic-geometric mean inequality:√ a + b ab ≤ for positive numbers a and b,20