Carsten Timm: Theory of superconductivity

Note that
√
n F (E k ) depends on β or temperature both explicitly and through the temperature dependence of
E k = ξk 2 + |∆ k(T )| 2 :
C = −2k B β 2 ∑ k
E k
(
∂n F
∂β
} {{ }
= E k
β
+ ∂n F
∂n F
∂E k
)
1 d |∆ k | 2
= −2k B β ∑ ∂E k 2E k dβ
k
(
)
∂n F
Ek 2 + 1 ∂E k 2 β d |∆ k| 2
. (10.58)
dβ
The first term is due to the explicit β dependence, i.e., to the change of occupation of quasiparticle states with
temperature. The second term results from the temperature dependence of the quasiparticle spectrum and is
absent for T ≥ T c , where |∆ k | 2 = 0 = const. The sum over k contains the factor
∂n F ∂ 1
e βE k
= β
∂E k ∂ βE k e βE = −β
k + 1 (e βE 2 = −β n F (E k )[1 − n F (E k )] (10.59)
k + 1)
so that
C = 2k B β 2 ∑ k
(
)
n F (1 − n F ) Ek 2 + 1 2 β d |∆ k| 2
. (10.60)
dβ
Here, n F (1 − n F ) is exponentially small for E k ≫ k B T . This means that for k B T ≪ ∆ min , where ∆ min is the
minimum superconducting gap, all terms in the sum are exponentially suppressed since k B T ≪ ∆ min ≤ E k . Thus
the heat capacity is exponentially small at low temperatures. This result is not specific to superconductors—all
systems with an energy gap for excitations show this behavior.
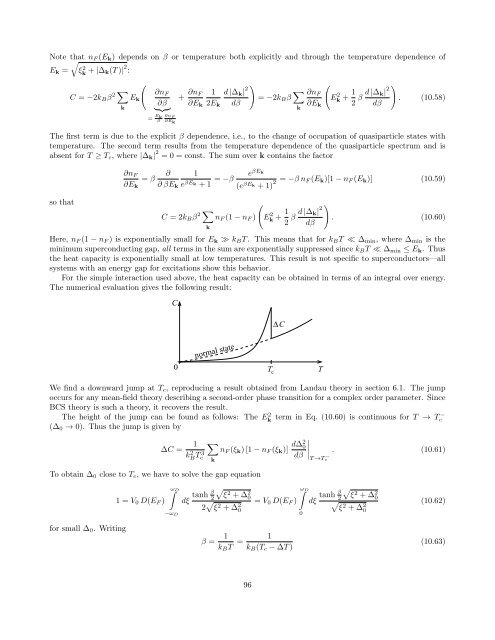
For the simple interaction used above, the heat capacity can be obtained in terms of an integral over energy.
The numerical evaluation gives the following result:
C
∆C
0
normal state
T c
T
We find a downward jump at T c , reproducing a result obtained from Landau theory in section 6.1. The jump
occurs for any mean-field theory describing a second-order phase transition for a complex order parameter. Since
BCS theory is such a theory, it recovers the result.
The height of the jump can be found as follows: The Ek 2 term in Eq. (10.60) is continuous for T → T c
−
(∆ 0 → 0). Thus the jump is given by
∆C = 1
k 2 B T 3 c
∑
To obtain ∆ 0 close to T c , we have to solve the gap equation
k
n F (ξ k ) [1 − n F (ξ k )] d∆2 0
dβ
∣ . (10.61)
T →T
−
c
∫ω D
1 = V 0 D(E F ) dξ tanh √ β
2 ξ2 + ∆ 2 0
2 √ ξ 2 + ∆ 2 0
−ω D
∫
= V 0 D(E F )
ω D
0
dξ tanh √ β
2 ξ2 + ∆ 2 0
√
ξ2 + ∆ 2 0
(10.62)
for small ∆ 0 . Writing
β = 1
k B T = 1
k B (T c − ∆T )
(10.63)
96