Set of supplementary notes.

More documents

Recommendations

Info

50 CHAPTER 3. FROM ATOMS TO SOLIDS m A ∂ 2 u nA ∂t 2 = K(u nB − u nA ) + K ′ (u n−1,B − u nA ) m B ∂ 2 u nB ∂t 2 = K ′ (u n+1A − u nB ) + K(u n,A − u nB ) (3.29) The solution of this is a little more complicated than before, but we can now intuitively see that there ought to be a new type of phonon mode by considering a particular limit of the parameters. Suppose the two atoms are quite strongly bound together in pairs, as sketched in the figure above: then we might expect that K ≫ K ′ , and to a first approximation the pairs can be treated as independent molecules. (We will also simplify the analysis by taking m A = m B = m.) Then every molecule will have a vibrational mode where the two atoms oscillate out of phase with each other with a frequency The corresponding coodinate which undergoes this oscillation is ω 2 o = 2K/m . (3.30) u opt (q = 0) = u A − u B (3.31) where I have explicitly remarked that this is at q = 0 if each molecule undergoes the oscillation in phase with the next. We can of course make a wavelike solution by choosing the correct phase relationship from one unit cell to the next — as sketched in Fig. 3.13, but if K ′ ≪ K this will hardly change the restoring force at all, and so the frequency of this so-called optical phonon mode will be almost independent of q. Figure 3.13: Dispersion of the optical and acoustic phonon branches in a diatomic chain, and a schematic picture of the atomic displacements in the optical mode at q=0 There are now two branches of the dispersion curve, along one of which the frequency vanishes linearly with wavevector, and where the other mode has a finite frequency as q → 0(see Fig. 3.14). The name “optical” arises because at these long wavelengths the optical phonons can interact (either by absorption, or scattering) with light, and are therefore prominent features in the absorption and Raman spectra of solids in the infrared spectrum. Phonons in three-dimensional solids The descriptions above are not too hard to generalise to three- dimensional solids, although the algebra gets overloaded with suffices.
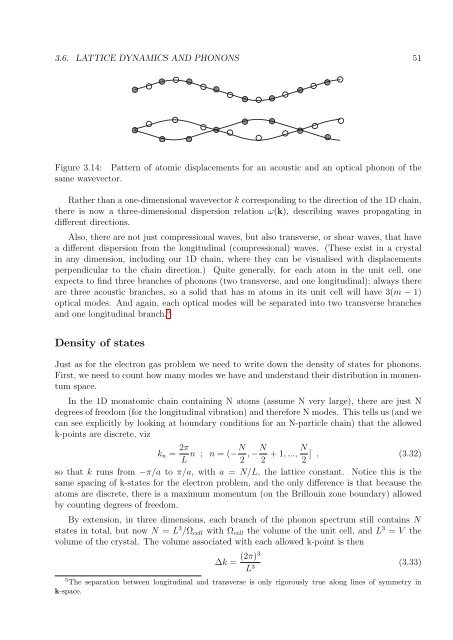
3.6. LATTICE DYNAMICS AND PHONONS 51 Figure 3.14: Pattern of atomic displacements for an acoustic and an optical phonon of the same wavevector. Rather than a one-dimensional wavevector k corresponding to the direction of the 1D chain, there is now a three-dimensional dispersion relation ω(k), describing waves propagating in different directions. Also, there are not just compressional waves, but also transverse, or shear waves, that have a different dispersion from the longitudinal (compressional) waves. (These exist in a crystal in any dimension, including our 1D chain, where they can be visualised with displacements perpendicular to the chain direction.) Quite generally, for each atom in the unit cell, one expects to find three branches of phonons (two transverse, and one longitudinal); always there are three acoustic branches, so a solid that has m atoms in its unit cell will have 3(m − 1) optical modes. And again, each optical modes will be separated into two transverse branches and one longitudinal branch. 5 Density of states Just as for the electron gas problem we need to write down the density of states for phonons. First, we need to count how many modes we have and understand their distribution in momentum space. In the 1D monatomic chain containing N atoms (assume N very large), there are just N degrees of freedom (for the longitudinal vibration) and therefore N modes. This tells us (and we can see explicitly by looking at boundary conditions for an N-particle chain) that the allowed k-points are discrete, viz k n = 2π L n ; n = (−N 2 , −N 2 + 1, ..., N 2 ] , (3.32) so that k runs from −π/a to π/a, with a = N/L, the lattice constant. Notice this is the same spacing of k-states for the electron problem, and the only difference is that because the atoms are discrete, there is a maximum momentum (on the Brillouin zone boundary) allowed by counting degrees of freedom. By extension, in three dimensions, each branch of the phonon spectrum still contains N states in total, but now N = L 3 /Ω cell with Ω cell the volume of the unit cell, and L 3 = V the volume of the crystal. The volume associated with each allowed k-point is then ∆k = (2π)3 L 3 (3.33) 5 The separation between longitudinal and transverse is only rigorously true along lines of symmetry in k-space.
Page 1: Quantum Condensed Matter Physics Pa
Page 4 and 5: 4 CONTENTS 5.1 Bands and Brillouin
Page 6 and 7: 6 CONTENTS also serves to give impo
Page 8 and 9: 8 CONTENTS
Page 10 and 11: 10 CHAPTER 1. CLASSICAL MODELS FOR
Page 24 and 25: 24 CHAPTER 2. SOMMERFELD THEORY Fig
Page 26 and 27: 26 CHAPTER 2. SOMMERFELD THEORY 2.4
Page 28 and 29: 28 CHAPTER 2. SOMMERFELD THEORY Res
Page 30 and 31: 30 CHAPTER 2. SOMMERFELD THEORY Thi
Page 32 and 33: 32 CHAPTER 2. SOMMERFELD THEORY
Page 34 and 35: 34 CHAPTER 3. FROM ATOMS TO SOLIDS
Page 56 and 57: 56 CHAPTER 4. ELECTRONIC STRUCTURE
Page 74 and 75: 74 CHAPTER 5. BANDSTRUCTURE OF REAL
Page 88 and 89: 88 CHAPTER 6. EXPERIMENTAL PROBES O
Page 100 and 101:
100 CHAPTER 7. SEMICONDUCTORS Figur
Page 102 and 103:
102 CHAPTER 7. SEMICONDUCTORS is fo
Page 104 and 105:
104 CHAPTER 7. SEMICONDUCTORS Figur
Page 106 and 107:
106 CHAPTER 8. SEMICONDUCTOR DEVICE
Page 108 and 109:
Page 110 and 111:
Page 112 and 113:
Page 114 and 115:
Page 116 and 117:
ecessitates variances in circuit th
Page 118 and 119:
nc... Freescale Semiconductor, I
Page 120 and 121:
Page 122 and 123:
Page 124 and 125:
124 CHAPTER 9. ELECTRONIC INSTABILI
Page 126 and 127:
Page 128 and 129:
Page 130 and 131:
Page 132 and 133:
Page 134 and 135:
Page 136 and 137:
Page 138 and 139:
Page 140 and 141:
Page 142 and 143:
Page 144 and 145:
Page 146 and 147:
146 CHAPTER 10. FERMI LIQUID THEORY
Page 148 and 149:
Page 150 and 151:
Page 152 and 153:
Page 154 and 155:
Page 156 and 157:
Page 158:
show all

Set of supplementary notes.

You also want an ePaper? Increase the reach of your titles

Delete template?

Save as template?