Conformally Invariant Variational Problems. - SAM

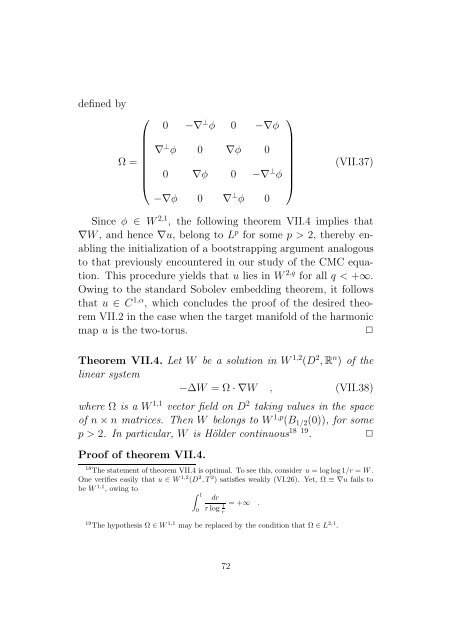
defined by
⎛
Ω =
⎜
⎝
0 −∇ ⊥ φ 0 −∇φ
∇ ⊥ φ 0 ∇φ 0
0 ∇φ 0 −∇ ⊥ φ
−∇φ 0 ∇ ⊥ φ 0
⎞
⎟
⎠
(VII.37)
Since φ ∈ W 2,1 , the following theorem VII.4 implies that
∇W, and hence ∇u, belong to L p for some p > 2, thereby enabling
the initialization of a bootstrapping argument analogous
to that previously encountered in our study of the CMC equation.
This procedure yields that u lies in W 2,q for all q < +∞.
Owing to the standard Sobolev embedding theorem, it follows
that u ∈ C 1,α , which concludes the proof of the desired theorem
VII.2 in the case when the target manifold of the harmonic
map u is the two-torus.
✷
Theorem VII.4. Let W be a solution in W 1,2 (D 2 ,R n ) of the
linear system
−∆W = Ω·∇W , (VII.38)
where Ω is a W 1,1 vector field on D 2 taking values in the space
of n×n matrices. Then W belongs to W 1,p (B 1/2 (0)), for some
p > 2. In particular, W is Hölder continuous 18 19 . ✷
Proof of theorem VII.4.
18 The statement of theorem VII.4 is optimal. To see this, consider u = loglog1/r = W.
One verifies easily that u ∈ W 1,2 (D 2 ,T 2 ) satisfies weakly (VI.26). Yet, Ω ≡ ∇u fails to
be W 1,1 , owing to
∫ 1
dr
0 rlog 1 = +∞ .
r
19 The hypothesis Ω ∈ W 1,1 may be replaced by the condition that Ω ∈ L 2,1 .
72