PERCOLATION AND DISORDERED SYSTEMS Geoffrey GRIMMETT

More documents

Recommendations

Info

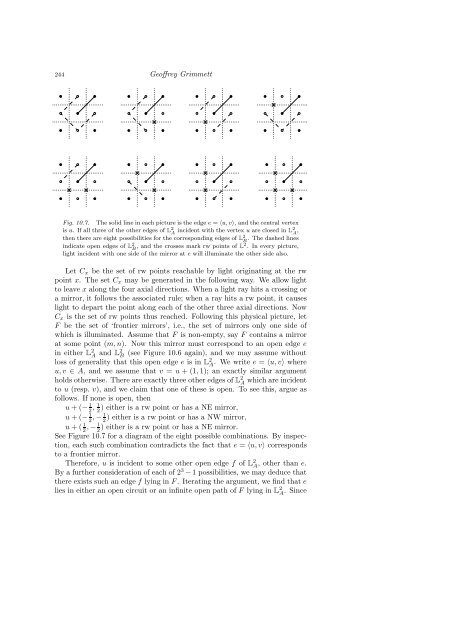
244 <strong>Geoffrey</strong> Grimmett Fig. 10.7. The solid line in each picture is the edge e = 〈u, v〉, and the central vertex is u. If all three of the other edges of L2 A incident with the vertex u are closed in L2 A , then there are eight possibilities for the corresponding edges of L2 B . The dashed lines indicate open edges of L2 B , and the crosses mark rw points of L2 . In every picture, light incident with one side of the mirror at e will illuminate the other side also. Let Cx be the set of rw points reachable by light originating at the rw point x. The set Cx may be generated in the following way. We allow light to leave x along the four axial directions. When a light ray hits a crossing or a mirror, it follows the associated rule; when a ray hits a rw point, it causes light to depart the point along each of the other three axial directions. Now Cx is the set of rw points thus reached. Following this physical picture, let F be the set of ‘frontier mirrors’, i.e., the set of mirrors only one side of which is illuminated. Assume that F is non-empty, say F contains a mirror at some point (m, n). Now this mirror must correspond to an open edge e in either L2 A and L2B (see Figure 10.6 again), and we may assume without loss of generality that this open edge e is in L2 A . We write e = 〈u, v〉 where u, v ∈ A, and we assume that v = u + (1, 1); an exactly similar argument holds otherwise. There are exactly three other edges of L2 A which are incident to u (resp. v), and we claim that one of these is open. To see this, argue as follows. If none is open, then u + (−1 1 2 , 2 ) either is a rw point or has a NE mirror, u + (−1 2 , −1 2 ) either is a rw point or has a NW mirror, u + ( 1 2 , −1 2 ) either is a rw point or has a NE mirror. See Figure 10.7 for a diagram of the eight possible combinations. By inspection, each such combination contradicts the fact that e = 〈u, v〉 corresponds to a frontier mirror. Therefore, u is incident to some other open edge f of L2 A , other than e. By a further consideration of each of 23 −1 possibilities, we may deduce that there exists such an edge f lying in F. Iterating the argument, we find that e lies in either an open circuit or an infinite open path of F lying in L2 A . Since
Percolation and Disordered Systems 245 there exists (by assumption) no infinite open path, this proves that f lies in an open circuit of F in L2 A . By taking the union over all e ∈ F, we obtain that F is a union of open circuits of L 2 A and L2 B . Each such circuit has an interior and an exterior, and x lies (by assumption, above) in every exterior. There are various ways of deducing that x is Z-non-localised, and here is such a way. Assume that x is Z-localised. Amongst the set of vertices {x + (n, 0) : n ≥ 1}, let y be the rightmost vertex at which there lies a frontier mirror. By the above argument, y belongs to some open circuit G of F (belonging to either L 2 A or L2 B ), whose exterior contains x. Since y is rightmost, we have that y ′ = y + (−1, 0) is illuminated by light originating at x, and that light traverses the edge 〈y ′ , y〉. Similarly, light does not traverse the edge 〈y, y ′′ 〉, where y ′′ = y +(1, 0). Therefore, the point y +( 1 2 , 0) of R2 lies in the interior of G, which contradicts the fact that y is rightmost. This completes the proof for part (b). � 10.3 General Labyrinths There are many possible types of reflector, especially in three and more dimensions. Consider Z d where d ≥ 2. Let I = {u1, u2, . . . , ud} be the set of positive unit vectors, and let I ± = {−1, +1} × I; members of I ± are written as ±uj. We make the following definition. A reflector is a map ρ : I ± → I ± satisfying ρ(−ρ(u)) = −u for all u ∈ I ± . We denote by R the set of reflectors. The ‘identity reflector’ is called a crossing (this is the identity map on I ± ), and denoted by +. The physical interpretation of a reflector is as follows. If light impinges on a reflector ρ, moving in a direction u (∈ I ± ) then it is required to depart the reflector in the direction ρ(u). The condition ρ(−ρ(u)) = −u arises from the reversibility of reflections. Using elementary combinatorics, one may calculate that the number of distinct reflectors in d dimensions is d� s=0 (2d)! (2s)! 2 d−s (d − s)! . A random labyrinth is constructed as follows. Let prw and p+ be nonnegative reals satisfying prw +p+ ≤ 1. Let Z = (Zx : x ∈ Zd ) be independent random variables taking values in R ∪ {∅}, with common mass function ⎧ ⎪⎨ prw if α = ∅ P(Z0 = α) = p+ if α = + ⎪⎩ (1 − prw − p+)π(ρ) if α = ρ ∈ R\{+}, where π is a prescribed probability mass function on R\{+}. We call a point x a crossing if Zx = +, and a random walk (rw) point if Zx = ∅.
Page 1:
PERCOLATION AND DISORDERED SYSTEMS
Page 4 and 5:
144 Geoffrey Grimmett ÈÊÇ��
Page 6 and 7:
146 Geoffrey Grimmett 8. Critical P
Page 8 and 9:
148 Geoffrey Grimmett θ(p) 1 pc 1
Page 10 and 11:
150 Geoffrey Grimmett physical imag
Page 12 and 13:
152 Geoffrey Grimmett Fig. 2.1. Par
Page 14 and 15:
154 Geoffrey Grimmett Fig. 2.2. An
Page 16 and 17:
156 Geoffrey Grimmett 3.1 Percolati
Page 18 and 19:
158 Geoffrey Grimmett Kesten [201]
Page 20 and 21:
160 Geoffrey Grimmett whence d dp P
Page 22 and 23:
162 Geoffrey Grimmett Fig. 4.1. An
Page 24 and 25:
164 Geoffrey Grimmett s 1 θ = 0 pc
Page 26 and 27:
166 Geoffrey Grimmett ∂B(n) 0 e f
Page 28 and 29:
168 Geoffrey Grimmett Fig. 4.5. A s
Page 30 and 31:
170 Geoffrey Grimmett Theorem 5.5 (
Page 32 and 33:
172 Geoffrey Grimmett Proof of Theo
Page 34 and 35:
174 Geoffrey Grimmett 5.3 Site and
Page 36 and 37:
176 Geoffrey Grimmett 6.1 Using Sub
Page 38 and 39:
178 Geoffrey Grimmett Now φ(p) = 0
Page 40 and 41:
180 Geoffrey Grimmett so that (3.10
Page 42 and 43:
182 Geoffrey Grimmett x 2 y 2 x 4 x
Page 44 and 45:
184 Geoffrey Grimmett Similarly, Pp
Page 46 and 47:
186 Geoffrey Grimmett It remains to
Page 48 and 49:
188 Geoffrey Grimmett Fix β < pc a
Page 50 and 51:
190 Geoffrey Grimmett where δ 2 =
Page 52 and 53:
192 Geoffrey Grimmett Fig. 7.1. Tak
Page 54 and 55: 194 Geoffrey Grimmett 7.2 Percolati
Page 56 and 57: 196 Geoffrey Grimmett 4. The open c
Page 58 and 59: 198 Geoffrey Grimmett For n > m, le
Page 60 and 61: 200 Geoffrey Grimmett Lemma 7.17. I
Page 62 and 63: 202 Geoffrey Grimmett Lemma 7.24. S
Page 64 and 65: 204 Geoffrey Grimmett 000 111 000 1
Page 66 and 67: 206 Geoffrey Grimmett Fig. 7.7. An
Page 68 and 69: 208 Geoffrey Grimmett Fig. 7.9. The
Page 70 and 71: 210 Geoffrey Grimmett Fig. 7.10. Il
Page 72 and 73: 212 Geoffrey Grimmett 8.1 Percolati
Page 74 and 75: 214 Geoffrey Grimmett may be shown
Page 76 and 77: 216 Geoffrey Grimmett Lemma 8.11. L
Page 78 and 79: 218 Geoffrey Grimmett 0 u noting th
Page 80 and 81: 220 Geoffrey Grimmett where α ′
Page 82 and 83: 222 Geoffrey Grimmett The infra-red
Page 84 and 85: 224 Geoffrey Grimmett 9. PERCOLATIO
Page 86 and 87: 226 Geoffrey Grimmett Fig. 9.2. If
Page 88 and 89: 228 Geoffrey Grimmett x Fig. 9.3. I
Page 90 and 91: 230 Geoffrey Grimmett for crossing
Page 92 and 93: 232 Geoffrey Grimmett 10. RANDOM WA
Page 94 and 95: 234 Geoffrey Grimmett the volume of
Page 96 and 97: 236 Geoffrey Grimmett We define sim
Page 98 and 99: 238 Geoffrey Grimmett We now use th
Page 100 and 101: 240 Geoffrey Grimmett p+ 1 pc(site)
Page 102 and 103: 242 Geoffrey Grimmett Fig. 10.6. Th
Page 106 and 107: 246 Geoffrey Grimmett A L d -path i
Page 108 and 109: 248 Geoffrey Grimmett where pc(site
Page 110 and 111: 250 Geoffrey Grimmett now make two
Page 116 and 117: 256 Geoffrey Grimmett II. P(C �=
Page 118 and 119: 258 Geoffrey Grimmett Theorem 11.13
Page 120 and 121: 260 Geoffrey Grimmett (b) Under wha
Page 122 and 123: 262 Geoffrey Grimmett is obtained b
Page 124 and 125: 264 Geoffrey Grimmett 13.2 Weak Lim
Page 126 and 127: 266 Geoffrey Grimmett where p = 1
Page 128 and 129: 268 Geoffrey Grimmett Evidently pg(
Page 130 and 131: 270 Geoffrey Grimmett That is to sa
Page 132 and 133: 272 Geoffrey Grimmett which, via Le
Page 134 and 135: 274 Geoffrey Grimmett Turning to th
Page 136 and 137: 276 Geoffrey Grimmett Each circuit
Page 138 and 139: 278 Geoffrey Grimmett If A(q) < 1 (
Page 140 and 141: 280 Geoffrey Grimmett REFERENCES 1.
Page 142 and 143: 282 Geoffrey Grimmett 30. Alexander
Page 144 and 145: 284 Geoffrey Grimmett 63. Berg, J.
Page 146 and 147: 286 Geoffrey Grimmett 93. Chayes, J
Page 148 and 149: 288 Geoffrey Grimmett 123. Durrett,
Page 150 and 151: 290 Geoffrey Grimmett 158. Grimmett
Page 152 and 153: 292 Geoffrey Grimmett 191. Higuchi,
Page 154 and 155:
294 Geoffrey Grimmett 224. Koteck´
Page 156 and 157:
296 Geoffrey Grimmett 259. Meester,
Page 158 and 159:
298 Geoffrey Grimmett 290. Newman,
Page 160 and 161:
300 Geoffrey Grimmett 323. Roy, R.
Page 162 and 163:
302 Geoffrey Grimmett 355. Wierman,
show all

PERCOLATION AND DISORDERED SYSTEMS Geoffrey GRIMMETT

Create successful ePaper yourself

Delete template?

Save as template?