PERCOLATION AND DISORDERED SYSTEMS Geoffrey GRIMMETT

xk(i, j)
Percolation and Disordered Systems 235
y
B
y ′
Ik(i, j)
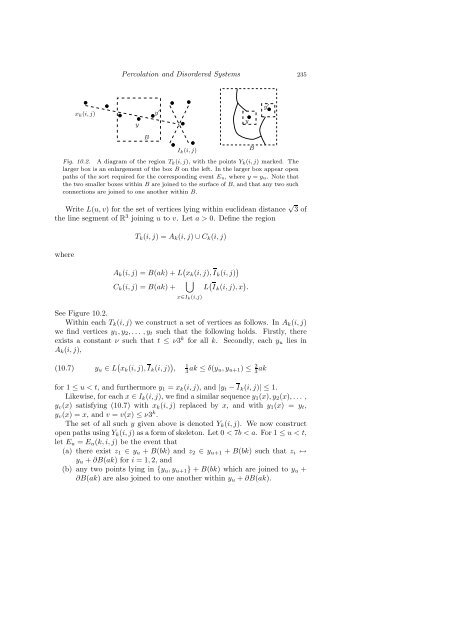
Fig. 10.2. A diagram of the region Tk(i, j), with the points Yk(i, j) marked. The
larger box is an enlargement of the box B on the left. In the larger box appear open
paths of the sort required for the corresponding event Eu, where y = yu. Note that
the two smaller boxes within B are joined to the surface of B, and that any two such
connections are joined to one another within B.
Write L(u, v) for the set of vertices lying within euclidean distance √ 3 of
the line segment of R 3 joining u to v. Let a > 0. Define the region
where
Tk(i, j) = Ak(i, j) ∪ Ck(i, j)
Ak(i, j) = B(ak) + L � xk(i, j), Ik(i, j) �
Ck(i, j) = B(ak) + �
L � Ik(i, j), x � .
x∈Ik(i,j)
See Figure 10.2.
Within each Tk(i, j) we construct a set of vertices as follows. In Ak(i, j)
we find vertices y1, y2, . . . , yt such that the following holds. Firstly, there
exists a constant ν such that t ≤ ν3 k for all k. Secondly, each yu lies in
Ak(i, j),
(10.7) yu ∈ L � xk(i, j), Ik(i, j) � , 1
3 ak ≤ δ(yu, yu+1) ≤ 2
3 ak
for 1 ≤ u < t, and furthermore y1 = xk(i, j), and |yt − Ik(i, j)| ≤ 1.
Likewise, for each x ∈ Ik(i, j), we find a similar sequence y1(x), y2(x), . . . ,
yv(x) satisfying (10.7) with xk(i, j) replaced by x, and with y1(x) = yt,
yv(x) = x, and v = v(x) ≤ ν3 k .
The set of all such y given above is denoted Yk(i, j). We now construct
open paths using Yk(i, j) as a form of skeleton. Let 0 < 7b < a. For 1 ≤ u < t,
let Eu = Eu(k, i, j) be the event that
(a) there exist z1 ∈ yu + B(bk) and z2 ∈ yu+1 + B(bk) such that zi ↔
yu + ∂B(ak) for i = 1, 2, and
(b) any two points lying in {yu, yu+1} + B(bk) which are joined to yu +
∂B(ak) are also joined to one another within yu + ∂B(ak).
y
B
y ′