PERCOLATION AND DISORDERED SYSTEMS Geoffrey GRIMMETT

226 Geoffrey Grimmett
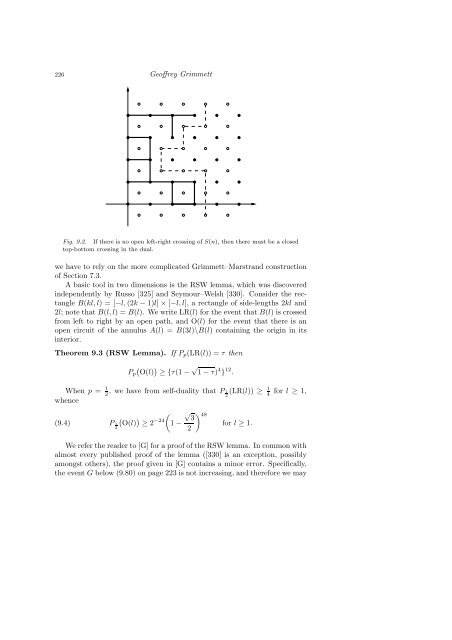
Fig. 9.2. If there is no open left-right crossing of S(n), then there must be a closed
top-bottom crossing in the dual.
we have to rely on the more complicated Grimmett–Marstrand construction
of Section 7.3.
A basic tool in two dimensions is the RSW lemma, which was discovered
independently by Russo [325] and Seymour–Welsh [330]. Consider the rectangle
B(kl, l) = [−l, (2k − 1)l] × [−l, l], a rectangle of side-lengths 2kl and
2l; note that B(l, l) = B(l). We write LR(l) for the event that B(l) is crossed
from left to right by an open path, and O(l) for the event that there is an
open circuit of the annulus A(l) = B(3l)\B(l) containing the origin in its
interior.
Theorem 9.3 (RSW Lemma). If Pp(LR(l)) = τ then
� � √
4 12
Pp O(l) ≥ {τ(1 − 1 − τ) } .
When p = 1
1
2 , we have from self-duality that P1 (LR(l)) ≥
2 4
whence
�
� � −24
(9.4) P1 O(l) ≥ 2 1 −
2
√ 3
2
� 48
for l ≥ 1.
for l ≥ 1,
We refer the reader to [G] for a proof of the RSW lemma. In common with
almost every published proof of the lemma ([330] is an exception, possibly
amongst others), the proof given in [G] contains a minor error. Specifically,
the event G below (9.80) on page 223 is not increasing, and therefore we may