PERCOLATION AND DISORDERED SYSTEMS Geoffrey GRIMMETT

More documents

Recommendations

Info

204 <strong>Geoffrey</strong> Grimmett 000 111 000 111 000 111 000 111 000 111 000 111 000 111 000 111 000 111 000 111 01 01 01 01 01 01 01 01 01 000 111 000 111 000 111 000 111 000 111 000 111 000 111 000 111 01 01 01 01 01 01 01 01 01 000 111 01 01 01 01 01 01 01 01 01 000 111 000 111 000 111 000 111 000 111 01 01 01 01 01 01 01 01 01 000 111 01 01 01 01 01 01 01 01 01 01 01 01 01 01 01 01 01 01 000 111 01 01 01 01 01 01 01 01 01 000 111 000 111 000 111 000 111 000 111 01 01 01 01 01 01 01 01 01 000 111 01 01 01 01 01 01 01 01 01 0 01 01 01 01 01 01 01 01 01 01 01 01 01 1 01 01 01 01 000 111 000 111 000 111 01 01 01 01 01 01 01 01 01 000 111 000 111 000 111 000 111 000 111 000 111 01 01 01 01 01 01 01 01 01 01 01 01 01 01 01 01 01 01 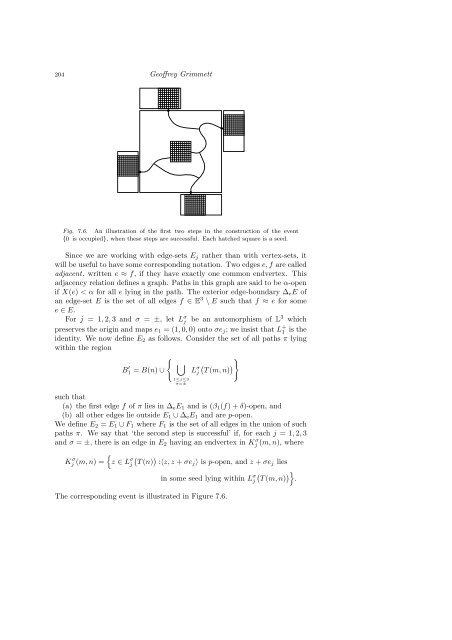Fig. 7.6. An illustration of the first two steps in the construction of the event {0 is occupied}, when these steps are successful. Each hatched square is a seed. Since we are working with edge-sets Ej rather than with vertex-sets, it will be useful to have some corresponding notation. Two edges e, f are called adjacent, written e ≈ f, if they have exactly one common endvertex. This adjacency relation defines a graph. Paths in this graph are said to be α-open if X(e) < α for all e lying in the path. The exterior edge-boundary ∆eE of an edge-set E is the set of all edges f ∈ E3 \ E such that f ≈ e for some e ∈ E. For j = 1, 2, 3 and σ = ±, let Lσ j be an automorphism of L3 which preserves the origin and maps e1 = (1, 0, 0) onto σej; we insist that L + 1 is the identity. We now define E2 as follows. Consider the set of all paths π lying within the region B ′ � � 1 = B(n) ∪ L σ� � j T(m, n) � 1≤j≤3 σ=± such that (a) the first edge f of π lies in ∆eE1 and is (β1(f) + δ)-open, and (b) all other edges lie outside E1 ∪ ∆eE1 and are p-open. We define E2 = E1 ∪ F1 where F1 is the set of all edges in the union of such paths π. We say that ‘the second step is successful’ if, for each j = 1, 2, 3 and σ = ±, there is an edge in E2 having an endvertex in Kσ j (m, n), where K σ � j (m, n) = z ∈ L σ� � j T(n) :〈z, z + σej〉 is p-open, and z + σej lies in some seed lying within L σ� � j T(m, n) � . The corresponding event is illustrated in Figure 7.6.
Percolation and Disordered Systems 205 Next we estimate the probability that the second step is successful, conditional on the first step being successful. Let G be the event that there exists a path in B(n)\B(m) from ∂B(m) to K(m, n), every edge e of which is p-open off ∆eE1 and whose unique edge f in ∆eE1 is (β1(f) + δ)-open. We write Gσ j for the corresponding event with K(m, n) replaced by Lσj (K(m, n)). We now apply Lemma 7.17 with R = B(m) and β = β1 to find that Therefore P � G σ j � � B(m) is a seed � > 1 − ǫ for j = 1, 2, 3, σ = ±. (7.30) P � G σ j occurs for all j, σ � � B(m) is a seed � > 1 − 6ǫ, so that the second step is successful with conditional probability at least 1 − 6ǫ. If the second step is successful, then we update the β, γ functions accordingly, setting (7.31) (7.32) ⎧ β1(e) if e �∈ EB ′ 1 , ⎪⎨ β1(e) + δ if e ∈ ∆eE1\E2, β2(e) = ⎪⎩ p if e ∈ ∆eE2\∆eE1, 0 otherwise, ⎧ γ1(e) if e ∈ E1, ⎪⎨ β1(e) + δ if e ∈ ∆eE1 ∩ E2, γ2(e) = p if e ∈ E2\(E1 ∪ ∆eE1), ⎪⎩ 1 otherwise. Suppose that the first two steps have been successful. We next aim to link the appropriate seeds in each Lσ j (T(m, n)) to a new seed lying in the bond-box 2σNej +B(N), i.e., the half-way box reached by exiting the origin in the direction σej. If we succeed with each of the six such extensions, then we terminate this stage of the process, and declare the vertex 0 of the renormalised lattice to be occupied; such success constitutes the definition of the term ‘occupied’. See Figure 7.7. We do not present all the details of this part of the construction, since they are very similar to those already described. Instead we concentrate on describing the basic strategy, and discussing any novel aspects of the construction. First, let B2 = b2+B(m) be the earliest seed (in some ordering of all copies of B(m)) all of whose edges lie in E2 ∩ ET(m,n). We now try to extend E2 to include a seed lying within the bond-box 2Ne1+B(N). Clearly B2 ⊆ Ne1 + B(N). In performing this extension, we encounter a ‘steering’ problem. It happens (by construction) that all coordinates of b2 are positive, implying that b2 + T(m, n) is not a subset of 2Ne1 + B(N). We therefore
Page 1:
PERCOLATION AND DISORDERED SYSTEMS
Page 4 and 5:
144 Geoffrey Grimmett ÈÊÇ��
Page 6 and 7:
146 Geoffrey Grimmett 8. Critical P
Page 8 and 9:
148 Geoffrey Grimmett θ(p) 1 pc 1
Page 10 and 11:
150 Geoffrey Grimmett physical imag
Page 12 and 13:
152 Geoffrey Grimmett Fig. 2.1. Par
Page 14 and 15: 154 Geoffrey Grimmett Fig. 2.2. An
Page 16 and 17: 156 Geoffrey Grimmett 3.1 Percolati
Page 18 and 19: 158 Geoffrey Grimmett Kesten [201]
Page 20 and 21: 160 Geoffrey Grimmett whence d dp P
Page 24 and 25: 164 Geoffrey Grimmett s 1 θ = 0 pc
Page 26 and 27: 166 Geoffrey Grimmett ∂B(n) 0 e f
Page 28 and 29: 168 Geoffrey Grimmett Fig. 4.5. A s
Page 30 and 31: 170 Geoffrey Grimmett Theorem 5.5 (
Page 32 and 33: 172 Geoffrey Grimmett Proof of Theo
Page 34 and 35: 174 Geoffrey Grimmett 5.3 Site and
Page 36 and 37: 176 Geoffrey Grimmett 6.1 Using Sub
Page 38 and 39: 178 Geoffrey Grimmett Now φ(p) = 0
Page 40 and 41: 180 Geoffrey Grimmett so that (3.10
Page 42 and 43: 182 Geoffrey Grimmett x 2 y 2 x 4 x
Page 44 and 45: 184 Geoffrey Grimmett Similarly, Pp
Page 46 and 47: 186 Geoffrey Grimmett It remains to
Page 48 and 49: 188 Geoffrey Grimmett Fix β < pc a
Page 50 and 51: 190 Geoffrey Grimmett where δ 2 =
Page 52 and 53: 192 Geoffrey Grimmett Fig. 7.1. Tak
Page 56 and 57: 196 Geoffrey Grimmett 4. The open c
Page 58 and 59: 198 Geoffrey Grimmett For n > m, le
Page 60 and 61: 200 Geoffrey Grimmett Lemma 7.17. I
Page 62 and 63: 202 Geoffrey Grimmett Lemma 7.24. S
Page 68 and 69: 208 Geoffrey Grimmett Fig. 7.9. The
Page 70 and 71: 210 Geoffrey Grimmett Fig. 7.10. Il
Page 74 and 75: 214 Geoffrey Grimmett may be shown
Page 76 and 77: 216 Geoffrey Grimmett Lemma 8.11. L
Page 78 and 79: 218 Geoffrey Grimmett 0 u noting th
Page 80 and 81: 220 Geoffrey Grimmett where α ′
Page 82 and 83: 222 Geoffrey Grimmett The infra-red
Page 84 and 85: 224 Geoffrey Grimmett 9. PERCOLATIO
Page 86 and 87: 226 Geoffrey Grimmett Fig. 9.2. If
Page 88 and 89: 228 Geoffrey Grimmett x Fig. 9.3. I
Page 90 and 91: 230 Geoffrey Grimmett for crossing
Page 92 and 93: 232 Geoffrey Grimmett 10. RANDOM WA
Page 94 and 95: 234 Geoffrey Grimmett the volume of
Page 96 and 97: 236 Geoffrey Grimmett We define sim
Page 98 and 99: 238 Geoffrey Grimmett We now use th
Page 100 and 101: 240 Geoffrey Grimmett p+ 1 pc(site)
Page 102 and 103: 242 Geoffrey Grimmett Fig. 10.6. Th
Page 106 and 107: 246 Geoffrey Grimmett A L d -path i
Page 108 and 109: 248 Geoffrey Grimmett where pc(site
Page 110 and 111: 250 Geoffrey Grimmett now make two
Page 114 and 115:
254 Geoffrey Grimmett Fig. 11.2. Th
Page 116 and 117:
256 Geoffrey Grimmett II. P(C �=
Page 118 and 119:
258 Geoffrey Grimmett Theorem 11.13
Page 120 and 121:
260 Geoffrey Grimmett (b) Under wha
Page 122 and 123:
262 Geoffrey Grimmett is obtained b
Page 124 and 125:
264 Geoffrey Grimmett 13.2 Weak Lim
Page 126 and 127:
266 Geoffrey Grimmett where p = 1
Page 128 and 129:
268 Geoffrey Grimmett Evidently pg(
Page 130 and 131:
270 Geoffrey Grimmett That is to sa
Page 132 and 133:
272 Geoffrey Grimmett which, via Le
Page 134 and 135:
274 Geoffrey Grimmett Turning to th
Page 136 and 137:
276 Geoffrey Grimmett Each circuit
Page 138 and 139:
278 Geoffrey Grimmett If A(q) < 1 (
Page 140 and 141:
280 Geoffrey Grimmett REFERENCES 1.
Page 142 and 143:
282 Geoffrey Grimmett 30. Alexander
Page 144 and 145:
284 Geoffrey Grimmett 63. Berg, J.
Page 146 and 147:
286 Geoffrey Grimmett 93. Chayes, J
Page 148 and 149:
288 Geoffrey Grimmett 123. Durrett,
Page 150 and 151:
290 Geoffrey Grimmett 158. Grimmett
Page 152 and 153:
292 Geoffrey Grimmett 191. Higuchi,
Page 154 and 155:
294 Geoffrey Grimmett 224. Koteck´
Page 156 and 157:
296 Geoffrey Grimmett 259. Meester,
Page 158 and 159:
298 Geoffrey Grimmett 290. Newman,
Page 160 and 161:
300 Geoffrey Grimmett 323. Roy, R.
Page 162 and 163:
302 Geoffrey Grimmett 355. Wierman,
show all

PERCOLATION AND DISORDERED SYSTEMS Geoffrey GRIMMETT

Create successful ePaper yourself

Delete template?

Save as template?