PERCOLATION AND DISORDERED SYSTEMS Geoffrey GRIMMETT

Percolation and Disordered Systems 197
B(n) B(m)
010101010101010101 00 1 1 0 0 1 1 0 0 1 1 0 0 1 1 0 0 1 1 0 0 1 1 0 0 1 1 0 0 1 1 0 0 1 1
T(m, n)
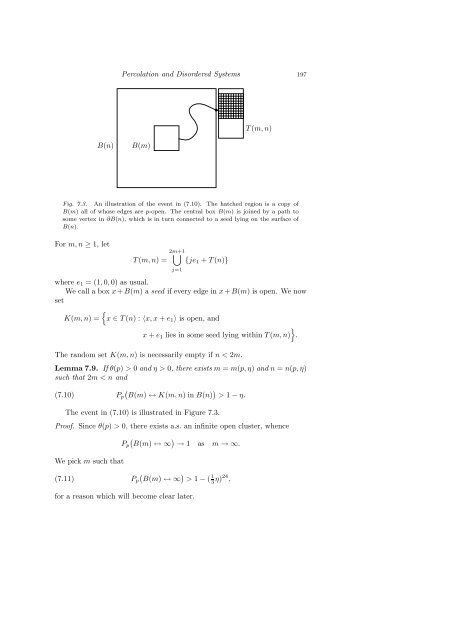
Fig. 7.3. An illustration of the event in (7.10). The hatched region is a copy of
B(m) all of whose edges are p-open. The central box B(m) is joined by a path to
some vertex in ∂B(n), which is in turn connected to a seed lying on the surface of
B(n).
For m, n ≥ 1, let
T(m, n) =
�
2m+1
j=1
{je1 + T(n)}
where e1 = (1, 0, 0) as usual.
We call a box x+B(m) a seed if every edge in x+B(m) is open. We now
set
�
K(m, n) = x ∈ T(n) : 〈x, x + e1〉 is open, and
�
x + e1 lies in some seed lying within T(m, n) .
The random set K(m, n) is necessarily empty if n < 2m.
Lemma 7.9. If θ(p) > 0 and η > 0, there exists m = m(p, η) and n = n(p, η)
such that 2m < n and
� �
(7.10) Pp B(m) ↔ K(m, n) in B(n) > 1 − η.
The event in (7.10) is illustrated in Figure 7.3.
Proof. Since θ(p) > 0, there exists a.s. an infinite open cluster, whence
We pick m such that
� �
Pp B(m) ↔ ∞ → 1 as m → ∞.
� �
1
(7.11) Pp B(m) ↔ ∞ > 1 − ( 3η)24 ,
for a reason which will become clear later.