PERCOLATION AND DISORDERED SYSTEMS Geoffrey GRIMMETT

206 Geoffrey Grimmett
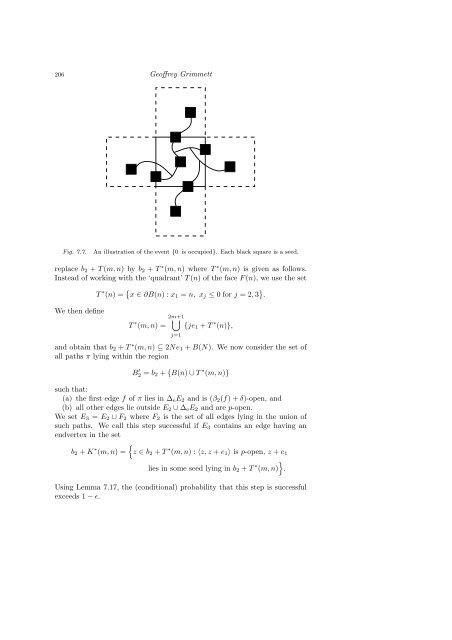
Fig. 7.7. An illustration of the event {0 is occupied}. Each black square is a seed.
replace b2 + T(m, n) by b2 + T ∗ (m, n) where T ∗ (m, n) is given as follows.
Instead of working with the ‘quadrant’ T(n) of the face F(n), we use the set
We then define
T ∗ (n) = � x ∈ ∂B(n) : x1 = n, xj ≤ 0 for j = 2, 3 � .
T ∗ (m, n) =
�
2m+1
j=1
{je1 + T ∗ (n)},
and obtain that b2 + T ∗ (m, n) ⊆ 2Ne1 + B(N). We now consider the set of
all paths π lying within the region
B ′ 2 = b2 + {B(n) ∪ T ∗ (m, n)}
such that:
(a) the first edge f of π lies in ∆eE2 and is (β2(f) + δ)-open, and
(b) all other edges lie outside E2 ∪ ∆eE2 and are p-open.
We set E3 = E2 ∪ F2 where F2 is the set of all edges lying in the union of
such paths. We call this step successful if E3 contains an edge having an
endvertex in the set
b2 + K ∗ �
(m, n) = z ∈ b2 + T ∗ (m, n) : 〈z, z + e1〉 is p-open, z + e1
lies in some seed lying in b2 + T ∗ �
(m, n) .
Using Lemma 7.17, the (conditional) probability that this step is successful
exceeds 1 − ǫ.