PERCOLATION AND DISORDERED SYSTEMS Geoffrey GRIMMETT

252 Geoffrey Grimmett
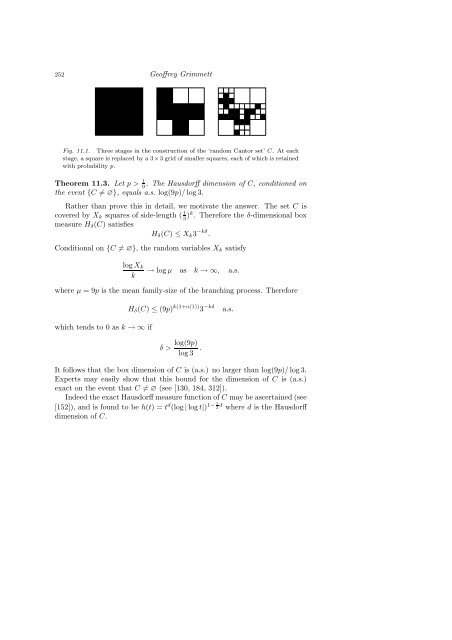
Fig. 11.1. Three stages in the construction of the ‘random Cantor set’ C. At each
stage, a square is replaced by a 3×3 grid of smaller squares, each of which is retained
with probability p.
Theorem 11.3. Let p > 1
9 . The Hausdorff dimension of C, conditioned on
the event {C �= ∅}, equals a.s. log(9p)/ log 3.
Rather than prove this in detail, we motivate the answer. The set C is
covered by Xk squares of side-length ( 1
3 )k . Therefore the δ-dimensional box
measure Hδ(C) satisfies
Hδ(C) ≤ Xk3 −kδ .
Conditional on {C �= ∅}, the random variables Xk satisfy
log Xk
k
→ log µ as k → ∞, a.s.
where µ = 9p is the mean family-size of the branching process. Therefore
which tends to 0 as k → ∞ if
Hδ(C) ≤ (9p) k(1+o(1)) 3 −kδ
δ > log(9p)
log 3 .
It follows that the box dimension of C is (a.s.) no larger than log(9p)/ log3.
Experts may easily show that this bound for the dimension of C is (a.s.)
exact on the event that C �= ∅ (see [130, 184, 312]).
Indeed the exact Hausdorff measure function of C may be ascertained (see
[152]), and is found to be h(t) = td 1 1− (log | log t|) 2 d where d is the Hausdorff
dimension of C.
a.s.