PERCOLATION AND DISORDERED SYSTEMS Geoffrey GRIMMETT

Percolation and Disordered Systems 233
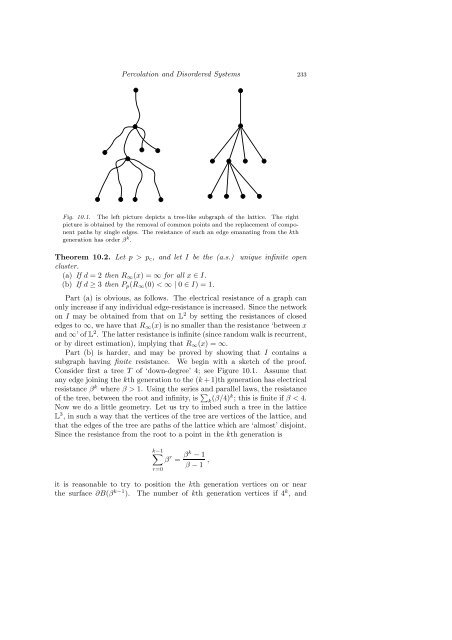
Fig. 10.1. The left picture depicts a tree-like subgraph of the lattice. The right
picture is obtained by the removal of common points and the replacement of component
paths by single edges. The resistance of such an edge emanating from the kth
generation has order β k .
Theorem 10.2. Let p > pc, and let I be the (a.s.) unique infinite open
cluster.
(a) If d = 2 then R∞(x) = ∞ for all x ∈ I.
(b) If d ≥ 3 then Pp(R∞(0) < ∞ | 0 ∈ I) = 1.
Part (a) is obvious, as follows. The electrical resistance of a graph can
only increase if any individual edge-resistance is increased. Since the network
on I may be obtained from that on L2 by setting the resistances of closed
edges to ∞, we have that R∞(x) is no smaller than the resistance ‘between x
and ∞’ of L2 . The latter resistance is infinite (since random walk is recurrent,
or by direct estimation), implying that R∞(x) = ∞.
Part (b) is harder, and may be proved by showing that I contains a
subgraph having finite resistance. We begin with a sketch of the proof.
Consider first a tree T of ‘down-degree’ 4; see Figure 10.1. Assume that
any edge joining the kth generation to the (k +1)th generation has electrical
resistance βk where β > 1. Using the series and parallel laws, the resistance
of the tree, between the root and infinity, is �
k (β/4)k ; this is finite if β < 4.
Now we do a little geometry. Let us try to imbed such a tree in the lattice
L3 , in such a way that the vertices of the tree are vertices of the lattice, and
that the edges of the tree are paths of the lattice which are ‘almost’ disjoint.
Since the resistance from the root to a point in the kth generation is
k−1 �
β r = βk − 1
β − 1 ,
r=0
it is reasonable to try to position the kth generation vertices on or near
the surface ∂B(β k−1 ). The number of kth generation vertices if 4 k , and