PERCOLATION AND DISORDERED SYSTEMS Geoffrey GRIMMETT

208 Geoffrey Grimmett
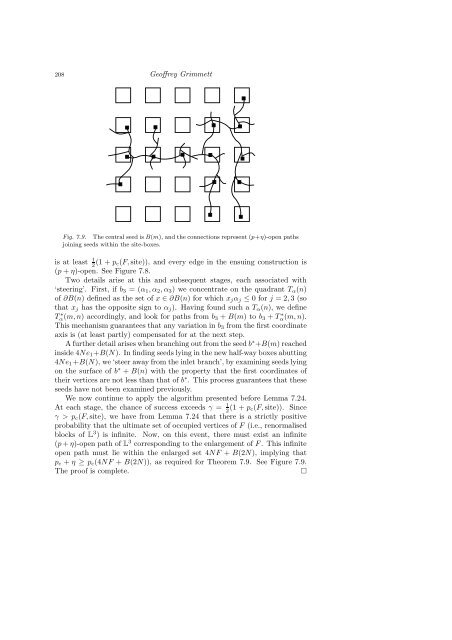
Fig. 7.9. The central seed is B(m), and the connections represent (p+η)-open paths
joining seeds within the site-boxes.
is at least 1
2 (1 + pc(F, site)), and every edge in the ensuing construction is
(p + η)-open. See Figure 7.8.
Two details arise at this and subsequent stages, each associated with
‘steering’. First, if b3 = (α1, α2, α3) we concentrate on the quadrant Tα(n)
of ∂B(n) defined as the set of x ∈ ∂B(n) for which xjαj ≤ 0 for j = 2, 3 (so
that xj has the opposite sign to αj). Having found such a Tα(n), we define
T ∗ α(m, n) accordingly, and look for paths from b3 + B(m) to b3 + T ∗ α(m, n).
This mechanism guarantees that any variation in b3 from the first coordinate
axis is (at least partly) compensated for at the next step.
A further detail arises when branching out from the seed b ∗ +B(m) reached
inside 4Ne1+B(N). In finding seeds lying in the new half-way boxes abutting
4Ne1+B(N), we ‘steer away from the inlet branch’, by examining seeds lying
on the surface of b ∗ + B(n) with the property that the first coordinates of
their vertices are not less than that of b ∗ . This process guarantees that these
seeds have not been examined previously.
We now continue to apply the algorithm presented before Lemma 7.24.
At each stage, the chance of success exceeds γ = 1
2 (1 + pc(F, site)). Since
γ > pc(F, site), we have from Lemma 7.24 that there is a strictly positive
probability that the ultimate set of occupied vertices of F (i.e., renormalised
blocks of L 3 ) is infinite. Now, on this event, there must exist an infinite
(p + η)-open path of L 3 corresponding to the enlargement of F. This infinite
open path must lie within the enlarged set 4NF + B(2N), implying that
pc + η ≥ pc(4NF + B(2N)), as required for Theorem 7.9. See Figure 7.9.
The proof is complete. �