PERCOLATION AND DISORDERED SYSTEMS Geoffrey GRIMMETT

More documents

Recommendations

Info

276 <strong>Geoffrey</strong> Grimmett Each circuit Γ of B d partitions the set EB of edges of B into three sets, being E = {edges of B exterior to Γ}, I = {edges of B interior to Γ}, Γ ′ = {edges of B crossing Γ}. The edges I form a connected subgraph of B. Our target is to obtain an upper bound for the probability that a given Γ is an outer circuit. This we shall do by examining certain partition functions. Since no open component of ω contains points lying in both the exterior and interior of an outer circuit, the event OC(Γ) = {Γ is an outer circuit} satisfies, for any dual circuit Γ having 0 in its interior, (13.41) φ 1 � � 1 B,p,q OC(Γ) = Z1 B,p,q � 1OC(Γ)(ω)πp(ω) ω = 1 Z1 (1 − p) B,p,q |Γ| Z 1 E (Γ)ZI where πp(ω) = p N(ω) (1 − p) |E|−N(ω) q k(ω) , Z 1 E (Γ) is the sum of πp(ω ′ ) over all ω ′ ∈ {0, 1} E with ‘1’ boundary conditions on ∂B and consistent with Γ being an outer circuit (i.e., property (c) above), and ZI is the sum of πp(ω ′′ ) over all ω ′′ ∈ {0, 1} I . Next we use duality. Let I d be the set of dual edges which cross the primal edges I, and let m be the number of vertices of B inside Γ. By (13.34), (13.42) ZI = q m−1 � � |I| 1 − p where p d satisfies (13.33), and where Z 1 I d ,p d ,q p d Z 1 I d ,p d ,q is the partition function for dual configurations, having wired boundary conditions, on the set V d of vertices incident to I d (i.e., all vertices of V d on its boundary are identified, as indicated in Figure 13.3). We note two general facts about partition functions. First, for any graph G, ZG,p,q ≥ 1 if q ≥ 1. Secondly, Z·,p,q has a property of supermultiplicativity when q ≥ 1, which implies in particular that Z 1 B,p,q ≥ Z 1 E(Γ)Z 1 I∪Γ ′ ,p,q for any circuit Γ of Bd . (This is where we use property (c) above.) Let I∗ = Id + ( 1 1 2 , 2 ), where Id is thought of as a subset of R2 . Note from Figure 13.3 that I ∗ ⊆ I ∪Γ ′ . Using the two general facts above, we have that (13.43) Z 1 B,p,q ≥ Z 1 E(Γ)Z 1 I ∗ ,p,q = Z 1 E(Γ)Z 1 I d ,p,q .
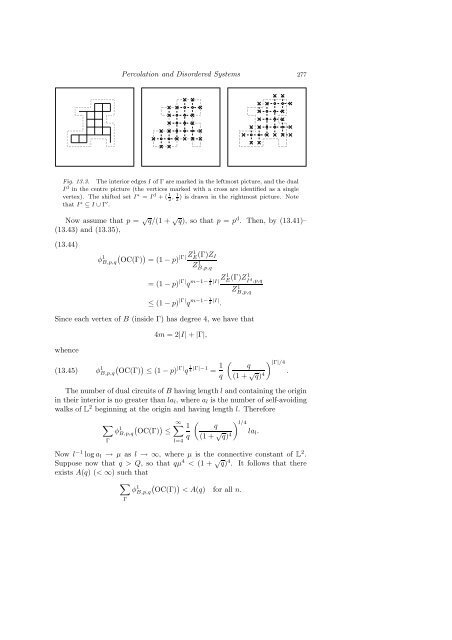
Percolation and Disordered Systems 277 Fig. 13.3. The interior edges I of Γ are marked in the leftmost picture, and the dual Id in the centre picture (the vertices marked with a cross are identified as a single vertex). The shifted set I∗ = Id + ( 1 1 , ) is drawn in the rightmost picture. Note 2 2 that I∗ ⊆ I ∪ Γ ′ . Now assume that p = √ q/(1 + √ q), so that p = p d . Then, by (13.41)– (13.43) and (13.35), (13.44) φ 1 � � |Γ| B,p,q OC(Γ) = (1 − p) Z1 E (Γ)ZI Z1 B,p,q = (1 − p) |Γ| q m−1−1 2 |I|Z1 E (Γ)Z1 Id ,p,q Z1 B,p,q ≤ (1 − p) |Γ| q m−1−1 2 |I| . Since each vertex of B (inside Γ) has degree 4, we have that whence 4m = 2|I| + |Γ|, (13.45) φ 1 � � 1 |Γ| B,p,q OC(Γ) ≤ (1 − p) q 4 |Γ|−1 = 1 q � q (1 + √ q) 4 � |Γ|/4 . The number of dual circuits of B having length l and containing the origin in their interior is no greater than lal, where al is the number of self-avoiding walks of L 2 beginning at the origin and having length l. Therefore � Γ φ 1 � � B,p,q OC(Γ) ≤ ∞� l=4 1 q � q (1 + √ q) 4 �l/4 lal. Now l −1 log al → µ as l → ∞, where µ is the connective constant of L 2 . Suppose now that q > Q, so that qµ 4 < (1 + √ q) 4 . It follows that there exists A(q) (< ∞) such that � Γ φ 1 � � B,p,q OC(Γ) < A(q) for all n.
Page 1:
PERCOLATION AND DISORDERED SYSTEMS
Page 4 and 5:
144 Geoffrey Grimmett ÈÊÇ��
Page 6 and 7:
146 Geoffrey Grimmett 8. Critical P
Page 8 and 9:
148 Geoffrey Grimmett θ(p) 1 pc 1
Page 10 and 11:
150 Geoffrey Grimmett physical imag
Page 12 and 13:
152 Geoffrey Grimmett Fig. 2.1. Par
Page 14 and 15:
154 Geoffrey Grimmett Fig. 2.2. An
Page 16 and 17:
156 Geoffrey Grimmett 3.1 Percolati
Page 18 and 19:
158 Geoffrey Grimmett Kesten [201]
Page 20 and 21:
160 Geoffrey Grimmett whence d dp P
Page 22 and 23:
Page 24 and 25:
164 Geoffrey Grimmett s 1 θ = 0 pc
Page 26 and 27:
166 Geoffrey Grimmett ∂B(n) 0 e f
Page 28 and 29:
168 Geoffrey Grimmett Fig. 4.5. A s
Page 30 and 31:
170 Geoffrey Grimmett Theorem 5.5 (
Page 32 and 33:
172 Geoffrey Grimmett Proof of Theo
Page 34 and 35:
174 Geoffrey Grimmett 5.3 Site and
Page 36 and 37:
176 Geoffrey Grimmett 6.1 Using Sub
Page 38 and 39:
178 Geoffrey Grimmett Now φ(p) = 0
Page 40 and 41:
180 Geoffrey Grimmett so that (3.10
Page 42 and 43:
182 Geoffrey Grimmett x 2 y 2 x 4 x
Page 44 and 45:
184 Geoffrey Grimmett Similarly, Pp
Page 46 and 47:
186 Geoffrey Grimmett It remains to
Page 48 and 49:
188 Geoffrey Grimmett Fix β < pc a
Page 50 and 51:
190 Geoffrey Grimmett where δ 2 =
Page 52 and 53:
192 Geoffrey Grimmett Fig. 7.1. Tak
Page 54 and 55:
Page 56 and 57:
196 Geoffrey Grimmett 4. The open c
Page 58 and 59:
198 Geoffrey Grimmett For n > m, le
Page 60 and 61:
200 Geoffrey Grimmett Lemma 7.17. I
Page 62 and 63:
202 Geoffrey Grimmett Lemma 7.24. S
Page 64 and 65:
204 Geoffrey Grimmett 000 111 000 1
Page 66 and 67:
Page 68 and 69:
208 Geoffrey Grimmett Fig. 7.9. The
Page 70 and 71:
210 Geoffrey Grimmett Fig. 7.10. Il
Page 72 and 73:
Page 74 and 75:
214 Geoffrey Grimmett may be shown
Page 76 and 77:
216 Geoffrey Grimmett Lemma 8.11. L
Page 78 and 79:
218 Geoffrey Grimmett 0 u noting th
Page 80 and 81:
220 Geoffrey Grimmett where α ′
Page 82 and 83:
222 Geoffrey Grimmett The infra-red
Page 84 and 85:
224 Geoffrey Grimmett 9. PERCOLATIO
Page 86 and 87: 226 Geoffrey Grimmett Fig. 9.2. If
Page 88 and 89: 228 Geoffrey Grimmett x Fig. 9.3. I
Page 90 and 91: 230 Geoffrey Grimmett for crossing
Page 92 and 93: 232 Geoffrey Grimmett 10. RANDOM WA
Page 94 and 95: 234 Geoffrey Grimmett the volume of
Page 96 and 97: 236 Geoffrey Grimmett We define sim
Page 98 and 99: 238 Geoffrey Grimmett We now use th
Page 100 and 101: 240 Geoffrey Grimmett p+ 1 pc(site)
Page 102 and 103: 242 Geoffrey Grimmett Fig. 10.6. Th
Page 106 and 107: 246 Geoffrey Grimmett A L d -path i
Page 108 and 109: 248 Geoffrey Grimmett where pc(site
Page 110 and 111: 250 Geoffrey Grimmett now make two
Page 116 and 117: 256 Geoffrey Grimmett II. P(C �=
Page 118 and 119: 258 Geoffrey Grimmett Theorem 11.13
Page 120 and 121: 260 Geoffrey Grimmett (b) Under wha
Page 122 and 123: 262 Geoffrey Grimmett is obtained b
Page 124 and 125: 264 Geoffrey Grimmett 13.2 Weak Lim
Page 126 and 127: 266 Geoffrey Grimmett where p = 1
Page 128 and 129: 268 Geoffrey Grimmett Evidently pg(
Page 130 and 131: 270 Geoffrey Grimmett That is to sa
Page 132 and 133: 272 Geoffrey Grimmett which, via Le
Page 134 and 135: 274 Geoffrey Grimmett Turning to th
Page 138 and 139: 278 Geoffrey Grimmett If A(q) < 1 (
Page 140 and 141: 280 Geoffrey Grimmett REFERENCES 1.
Page 142 and 143: 282 Geoffrey Grimmett 30. Alexander
Page 144 and 145: 284 Geoffrey Grimmett 63. Berg, J.
Page 146 and 147: 286 Geoffrey Grimmett 93. Chayes, J
Page 148 and 149: 288 Geoffrey Grimmett 123. Durrett,
Page 150 and 151: 290 Geoffrey Grimmett 158. Grimmett
Page 152 and 153: 292 Geoffrey Grimmett 191. Higuchi,
Page 154 and 155: 294 Geoffrey Grimmett 224. Koteck´
Page 156 and 157: 296 Geoffrey Grimmett 259. Meester,
Page 158 and 159: 298 Geoffrey Grimmett 290. Newman,
Page 160 and 161: 300 Geoffrey Grimmett 323. Roy, R.
Page 162 and 163: 302 Geoffrey Grimmett 355. Wierman,
show all

PERCOLATION AND DISORDERED SYSTEMS Geoffrey GRIMMETT

Create successful ePaper yourself

Delete template?

Save as template?