PERCOLATION AND DISORDERED SYSTEMS Geoffrey GRIMMETT

Percolation and Disordered Systems 225
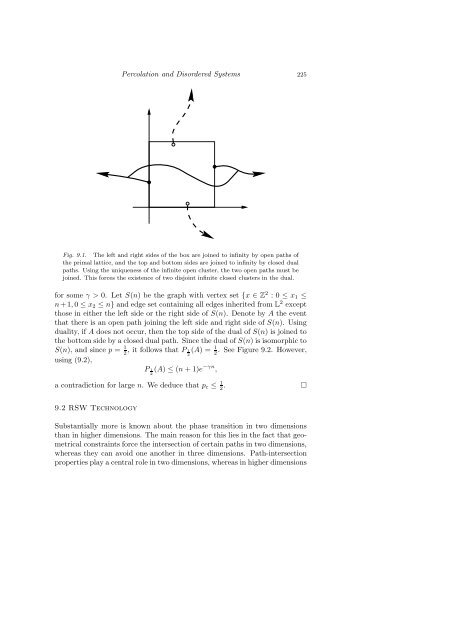
Fig. 9.1. The left and right sides of the box are joined to infinity by open paths of
the primal lattice, and the top and bottom sides are joined to infinity by closed dual
paths. Using the uniqueness of the infinite open cluster, the two open paths must be
joined. This forces the existence of two disjoint infinite closed clusters in the dual.
for some γ > 0. Let S(n) be the graph with vertex set {x ∈ Z 2 : 0 ≤ x1 ≤
n+1, 0 ≤ x2 ≤ n} and edge set containing all edges inherited from L 2 except
those in either the left side or the right side of S(n). Denote by A the event
that there is an open path joining the left side and right side of S(n). Using
duality, if A does not occur, then the top side of the dual of S(n) is joined to
the bottom side by a closed dual path. Since the dual of S(n) is isomorphic to
S(n), and since p = 1
1
2 , it follows that P1 (A) =
2 2 . See Figure 9.2. However,
using (9.2),
P1
2 (A) ≤ (n + 1)e−γn ,
a contradiction for large n. We deduce that pc ≤ 1
2 . �
9.2 RSW Technology
Substantially more is known about the phase transition in two dimensions
than in higher dimensions. The main reason for this lies in the fact that geometrical
constraints force the intersection of certain paths in two dimensions,
whereas they can avoid one another in three dimensions. Path-intersection
properties play a central role in two dimensions, whereas in higher dimensions