PERCOLATION AND DISORDERED SYSTEMS Geoffrey GRIMMETT

192 Geoffrey Grimmett
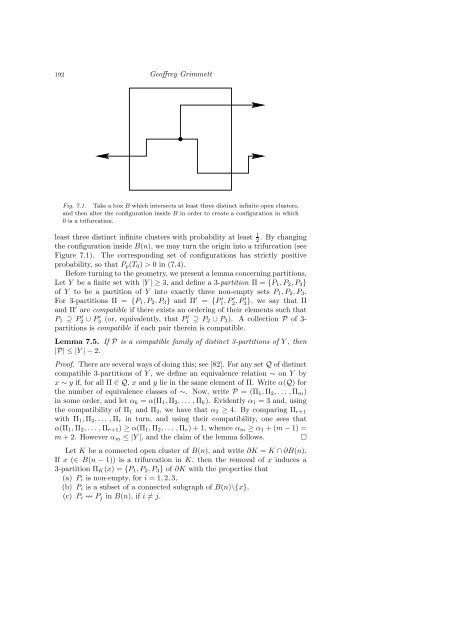
Fig. 7.1. Take a box B which intersects at least three distinct infinite open clusters,
and then alter the configuration inside B in order to create a configuration in which
0 is a trifurcation.
least three distinct infinite clusters with probability at least 1
2 . By changing
the configuration inside B(n), we may turn the origin into a trifurcation (see
Figure 7.1). The corresponding set of configurations has strictly positive
probability, so that Pp(T0) > 0 in (7.4).
Before turning to the geometry, we present a lemma concerning partitions.
Let Y be a finite set with |Y | ≥ 3, and define a 3-partition Π = {P1, P2, P3}
of Y to be a partition of Y into exactly three non-empty sets P1, P2, P3.
}, we say that Π
For 3-partitions Π = {P1, P2, P3} and Π ′ = {P ′ 1 , P ′ 2 , P ′ 3
and Π ′ are compatible if there exists an ordering of their elements such that
P1 ⊇ P ′ 2 ∪ P ′ 3 (or, equivalently, that P ′ 1 ⊇ P2 ∪ P3). A collection P of 3partitions
is compatible if each pair therein is compatible.
Lemma 7.5. If P is a compatible family of distinct 3-partitions of Y , then
|P| ≤ |Y | − 2.
Proof. There are several ways of doing this; see [82]. For any set Q of distinct
compatible 3-partitions of Y , we define an equivalence relation ∼ on Y by
x ∼ y if, for all Π ∈ Q, x and y lie in the same element of Π. Write α(Q) for
the number of equivalence classes of ∼. Now, write P = (Π1, Π2, . . . , Πm)
in some order, and let αk = α(Π1, Π2, . . . , Πk). Evidently α1 = 3 and, using
the compatibility of Π1 and Π2, we have that α2 ≥ 4. By comparing Πr+1
with Π1, Π2, . . . , Πr in turn, and using their compatibility, one sees that
α(Π1, Π2, . . . , Πr+1) ≥ α(Π1, Π2, . . . , Πr) + 1, whence αm ≥ α1 + (m − 1) =
m + 2. However αm ≤ |Y |, and the claim of the lemma follows. �
Let K be a connected open cluster of B(n), and write ∂K = K ∩ ∂B(n).
If x (∈ B(n − 1)) is a trifurcation in K, then the removal of x induces a
3-partition ΠK(x) = {P1, P2, P3} of ∂K with the properties that
(a) Pi is non-empty, for i = 1, 2, 3,
(b) Pi is a subset of a connected subgraph of B(n)\{x},
(c) Pi � Pj in B(n), if i �= j.