PERCOLATION AND DISORDERED SYSTEMS Geoffrey GRIMMETT

182 Geoffrey Grimmett
x 2
y 2
x 4
x 3
y 3
y 4
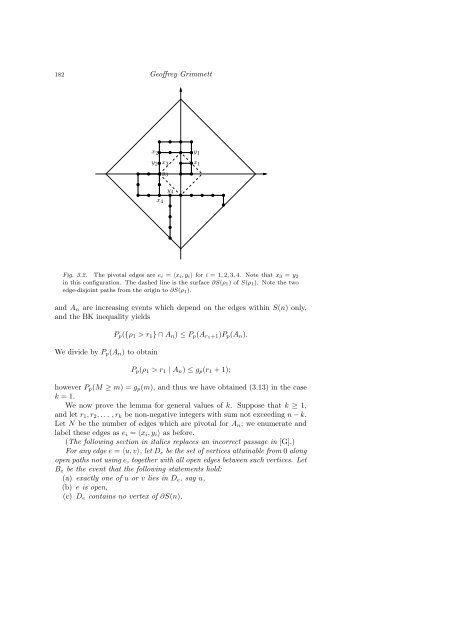
Fig. 3.2. The pivotal edges are ei = 〈xi, yi〉 for i = 1, 2,3, 4. Note that x3 = y2
in this configuration. The dashed line is the surface ∂S(ρ1) of S(ρ1). Note the two
edge-disjoint paths from the origin to ∂S(ρ1).
and An are increasing events which depend on the edges within S(n) only,
and the BK inequality yields
We divide by Pp(An) to obtain
Pp({ρ1 > r1} ∩ An) ≤ Pp(Ar1+1)Pp(An).
y 1
x 1
Pp(ρ1 > r1 | An) ≤ gp(r1 + 1);
however Pp(M ≥ m) = gp(m), and thus we have obtained (3.13) in the case
k = 1.
We now prove the lemma for general values of k. Suppose that k ≥ 1,
and let r1, r2, . . . , rk be non-negative integers with sum not exceeding n − k.
Let N be the number of edges which are pivotal for An; we enumerate and
label these edges as ei = 〈xi, yi〉 as before.
(The following section in italics replaces an incorrect passage in [G].)
For any edge e = 〈u, v〉, let De be the set of vertices attainable from 0 along
open paths not using e, together with all open edges between such vertices. Let
Be be the event that the following statements hold:
(a) exactly one of u or v lies in De, say u,
(b) e is open,
(c) De contains no vertex of ∂S(n),