derived categories of twisted sheaves on calabi-yau manifolds
derived categories of twisted sheaves on calabi-yau manifolds
derived categories of twisted sheaves on calabi-yau manifolds
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
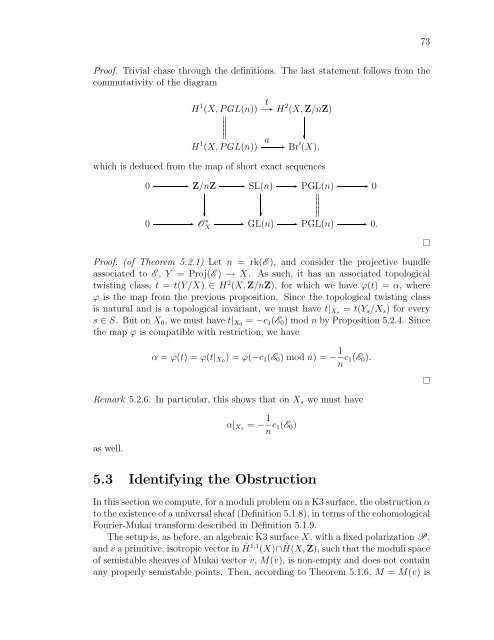
Pro<str<strong>on</strong>g>of</str<strong>on</strong>g>. Trivial chase through the definiti<strong>on</strong>s. The last statement follows from the<br />
commutativity <str<strong>on</strong>g>of</str<strong>on</strong>g> the diagram<br />
H 1 (X, P GL(n))<br />
<br />
<br />
<br />
<br />
<br />
H 1 (X, P GL(n))<br />
t<br />
✲ H 2 (X, Z/nZ)<br />
a<br />
❄<br />
✲ ′<br />
Br (X),<br />
which is deduced from the map <str<strong>on</strong>g>of</str<strong>on</strong>g> short exact sequences<br />
0 ✲ Z/nZ ✲ SL(n) ✲ PGL(n) ✲ 0<br />
<br />
<br />
<br />
<br />
<br />
❄<br />
❄<br />
0 ✲ ∗<br />
O ✲<br />
X GL(n) ✲ PGL(n) ✲ 0.<br />
Pro<str<strong>on</strong>g>of</str<strong>on</strong>g>. (<str<strong>on</strong>g>of</str<strong>on</strong>g> Theorem 5.2.1) Let n = rk(E ), and c<strong>on</strong>sider the projective bundle<br />
associated to E , Y = Proj(E ) → X. As such, it has an associated topological<br />
twisting class, t = t(Y/X) ∈ H 2 (X, Z/nZ), for which we have ϕ(t) = α, where<br />
ϕ is the map from the previous propositi<strong>on</strong>. Since the topological twisting class<br />
is natural and is a topological invariant, we must have t|Xs = t(Ys/Xs) for every<br />
s ∈ S. But <strong>on</strong> X0, we must have t|X0 = −c1(E0) mod n by Propositi<strong>on</strong> 5.2.4. Since<br />
the map ϕ is compatible with restricti<strong>on</strong>, we have<br />
α = ϕ(t) = ϕ(t|X0) = ϕ(−c1(E0) mod n) = − 1<br />
n c1(E0).<br />
Remark 5.2.6. In particular, this shows that <strong>on</strong> Xs we must have<br />
as well.<br />
α|Xs = − 1<br />
n c1(E0)<br />
5.3 Identifying the Obstructi<strong>on</strong><br />
In this secti<strong>on</strong> we compute, for a moduli problem <strong>on</strong> a K3 surface, the obstructi<strong>on</strong> α<br />
to the existence <str<strong>on</strong>g>of</str<strong>on</strong>g> a universal sheaf (Definiti<strong>on</strong> 5.1.8), in terms <str<strong>on</strong>g>of</str<strong>on</strong>g> the cohomological<br />
Fourier-Mukai transform described in Definiti<strong>on</strong> 5.1.9.<br />
The setup is, as before, an algebraic K3 surface X, with a fixed polarizati<strong>on</strong> P,<br />
and v a primitive, isotropic vector in ˜ H 1,1 (X)∩ ˜ H(X, Z), such that the moduli space<br />
<str<strong>on</strong>g>of</str<strong>on</strong>g> semistable <str<strong>on</strong>g>sheaves</str<strong>on</strong>g> <str<strong>on</strong>g>of</str<strong>on</strong>g> Mukai vector v, M(v), is n<strong>on</strong>-empty and does not c<strong>on</strong>tain<br />
any properly semistable points. Then, according to Theorem 5.1.6, M = M(v) is<br />
73