Aluminium Design and Construction John Dwight

We now turn to monosymmetric sections (Figure 10.2(c)). The above
treatment holds valid for these in principle, but the calculation is longer,
because we need two parameters (w, z) to specify the neutral axis nn. The
whole section is split up into convenient elements, none of which must
straddle nn. A convenient pair of axes OX and OY is selected, enabling
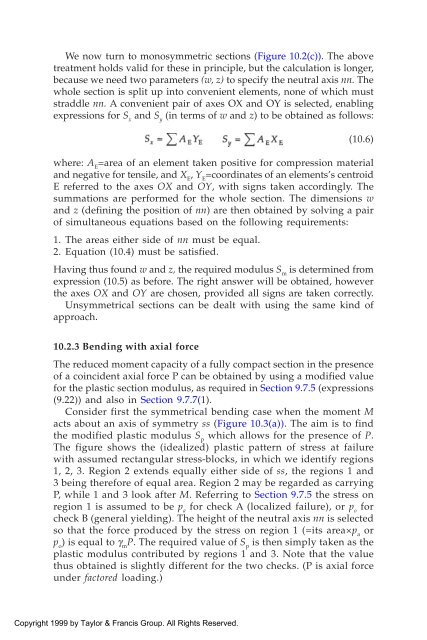
expressions for S x and S y (in terms of w and z) to be obtained as follows:
(10.6)
where: AE =area of an element taken positive for compression material
and negative for tensile, and XE , YE =coordinates of an elements’s centroid
E referred to the axes OX and OY, with signs taken accordingly. The
summations are performed for the whole section. The dimensions w
and z (defining the position of nn) are then obtained by solving a pair
of simultaneous equations based on the following requirements:
1. The areas either side of nn must be equal.
2. Equation (10.4) must be satisfied.
Having thus found w and z, the required modulus Sm is determined from
expression (10.5) as before. The right answer will be obtained, however
the axes OX and OY are chosen, provided all signs are taken correctly.
Unsymmetrical sections can be dealt with using the same kind of
approach.
10.2.3 Bending with axial force
The reduced moment capacity of a fully compact section in the presence
of a coincident axial force P can be obtained by using a modified value
for the plastic section modulus, as required in Section 9.7.5 (expressions
(9.22)) and also in Section 9.7.7(1).
Consider first the symmetrical bending case when the moment M
acts about an axis of symmetry ss (Figure 10.3(a)). The aim is to find
the modified plastic modulus S p which allows for the presence of P.
The figure shows the (idealized) plastic pattern of stress at failure
with assumed rectangular stress-blocks, in which we identify regions
1, 2, 3. Region 2 extends equally either side of ss, the regions 1 and
3 being therefore of equal area. Region 2 may be regarded as carrying
P, while 1 and 3 look after M. Referring to Section 9.7.5 the stress on
region 1 is assumed to be p a for check A (localized failure), or p o for
check B (general yielding). The height of the neutral axis nn is selected
so that the force produced by the stress on region 1 (=its area×p a or
p o ) is equal to � m P. The required value of S p is then simply taken as the
plastic modulus contributed by regions 1 and 3. Note that the value
thus obtained is slightly different for the two checks. (P is axial force
under factored loading.)
Copyright 1999 by Taylor & Francis Group. All Rights Reserved.