Contents Telektronikk - Telenor

Appendix B
Evaluation of two
logarithmic sums
To derive (2.12) and (2.13) we denote
n�
S = log(z − rk)
k=2
(where we use theprincipal value of the
logarithm). We also denote
g(ζ) =1− A(ζ)
.
ζn The contour-integral
�
1
log(ζ − z)
2πi Γ
g′ (ζ)
g(ζ) dζ
n�
= log(z − rk) − n log z
k=1
(B.1)
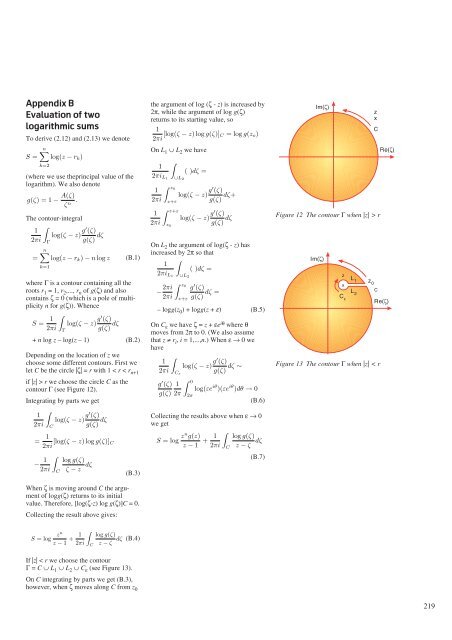
where Γ is a contour containing all the
roots r1 = 1, r2 ,..., rn of g(ζ) and also
contains ζ = 0 (which is a pole of multiplicity
n for g(ζ)). Whence
S = 1
2πi
�
Γ
log(ζ − z) g′ (ζ)
g(ζ) dζ
+ n log z – log(z – 1) (B.2)
Depending on the location of z we
choose some different contours. First we
let C be the circle |ζ| = r with 1 < r < r n+1
if |z| > r we choose the circle C as the
contour Γ (see Figure 12).
Integrating by parts we get
�
1
log(ζ − z)
2πi C
g′ (ζ)
g(ζ) dζ
= 1
[log(ζ − z)logg(ζ)]C
2πi
− 1
�
log g(ζ)
2πi ζ − z dζ
C
(B.3)
When ζ is moving around C the argument
of logg(ζ) returns to its initial
value. Therefore, [log(ζ-z) log g(ζ)]C = 0.
Collecting the result above gives:
S =log zn
�
1 log g(ζ)
+
z − 1 2πi C z − ζ dζ
(B.4)
If |z| < r we choose the contour
Γ = C ∪ L1 ∪ L2 ∪ Cε (see Figure 13).
On C integrating by parts we get (B.3),
however, when ζ moves along C from z0 the argument of log (ζ - z) is increased by
2π, while the argument of log g(ζ)
returns to its starting value, so
1
2πi [log(ζ − z)logg(ζ)]C =logg(zo)
On L 1 ∪ L 2 we have
�
1
()dζ =
2πiL1 ∪L2
� z0
1
log(ζ − z)
2πi z+ε
g′ (ζ)
g(ζ) dζ+
� z+ε
1
log(ζ − z)
2πi
g′ (ζ)
g(ζ) dζ
z0
On L2 the argument of log(ζ - z) has
increased by 2π so that
�
1
()dζ =
2πiL1 ∪L2
− 2πi
� z0
g
2πi
′ (ζ)
dζ =
g(ζ)
z+ε
– logg(z 0 ) + logg(z + ε) (B.5)
On Cε we have ζ = z + εeiθ where θ
moves from 2π to 0. (We also assume
that z ≠ ri , i = 1,...,n.) When ε→0 we
have
�
1
log(ζ − z)
2πi Cε
(B.6)
g′ (ζ)
dζ ∼
g(ζ)
g ′ � 0
(ζ) 1
log(εe
g(ζ) 2π 2π
iθ )(εe iθ )dθ → 0
Collecting the results above when ε→0 we get
S =log zn �
g(z) 1 log g(ζ)
+
z − 1 2πi C z − ζ dζ
(B.7)
Im(ζ)
z
x
Figure 12 The contour Γ when |z| > r
Im(ζ)
z
x
C ε
L 1
L 2
Figure 13 The contour Γ when |z| < r
C
z 0
C
Re(ζ)
Re(ζ)
219