Contents Telektronikk - Telenor

Point-to-point losses in hierarchical alternative routing
BY BENGT WALLSTRÖM
Abstract
In a previous paper [9] the joint state
distributions of carried and overflowing
partial traffics were considered,
suggesting possibilities of point-topoint
blocking calculations in alternative
routing networks. The present
paper will show how a simple extension
of Wilkinson’s Equivalent Random
Theory (ERT) can be used for
that purpose in a hierarchical network.
1 Introduction
To recall some basic elements of overflow
traffic theory, let us consider the
scheme (Figure 1) studied by Wilkinson
in his Theories for toll traffic engineering
in the USA [10].
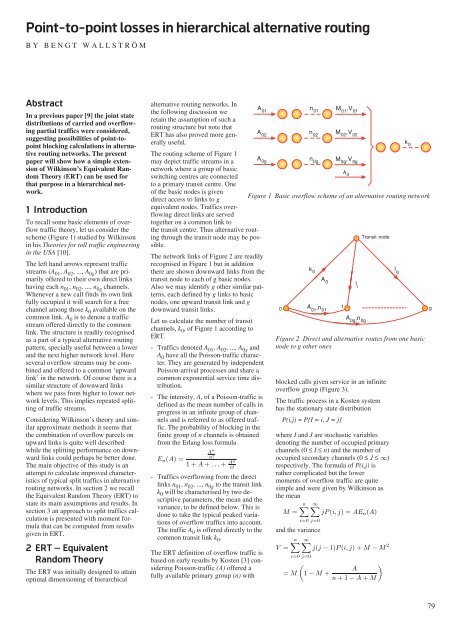
The left hand arrows represent traffic
streams (A01 , A02 , ..., A0g ) that are primarily
offered to their own direct links
having each n01 , n02 , ..., n0g channels.
Whenever a new call finds its own link
fully occupied it will search for a free
channel among those k0 available on the
common link. A0 is to denote a traffic
stream offered directly to the common
link. The structure is readily recognised
as a part of a typical alternative routing
pattern, specially useful between a lower
and the next higher network level. Here
several overflow streams may be combined
and offered to a common ‘upward
link’ in the network. Of course there is a
similar structure of downward links
where we pass from higher to lower network
levels. This implies repeated splitting
of traffic streams.
Considering Wilkinson’s theory and similar
approximate methods it seems that
the combination of overflow parcels on
upward links is quite well described
while the splitting performance on downward
links could perhaps be better done.
The main objective of this study is an
attempt to calculate improved characteristics
of typical split traffics in alternative
routing networks. In section 2 we recall
the Equivalent Random Theory (ERT) to
state its main assumptions and results. In
section 3 an approach to split traffics calculation
is presented with moment formula
that can be computed from results
given in ERT.
2 ERT – Equivalent
Random Theory
The ERT was initially designed to attain
optimal dimensioning of hierarchical
alternative routing networks. In
A
the following discussion we
01
retain the assumption of such a
routing structure but note that
ERT has also proved more gen- A02 erally useful.
The routing scheme of Figure 1
may depict traffic streams in a
A0g network where a group of basic
switching centres are connected
to a primary transit centre. One
of the basic nodes is given
direct access to links to g
equivalent nodes. Traffics overflowing
direct links are served
together on a common link to
the transit centre. Thus alternative routing
through the transit node may be possible.
The network links of Figure 2 are readily
recognised in Figure 1 but in addition
there are shown downward links from the
transit node to each of g basic nodes.
Also we may identify g other similar patterns,
each defined by g links to basic
nodes, one upward transit link and g
downward transit links.
Let us calculate the number of transit
channels, k0 , of Figure 1 according to
ERT.
- Traffics denoted A01 , A02 , ..., A0g and
A0 have all the Poisson-traffic character.
They are generated by independent
Poisson-arrival processes and share a
common exponential service time distribution.
- The intensity, A, of a Poisson-traffic is
defined as the mean number of calls in
progress in an infinite group of channels
and is referred to as offered traffic.
The probability of blocking in the
finite group of n channels is obtained
from the Erlang loss formula
En(A) =
A n
n!
1+A + ...+ An
n!
- Traffics overflowing from the direct
links n 01 , n 02 , ..., n 0g to the transit link
k 0 will be characterised by two descriptive
parameters, the mean and the
variance, to be defined below. This is
done to take the typical peaked variations
of overflow traffics into account.
The traffic A 0 is offered directly to the
common transit link k 0 .
The ERT definition of overflow traffic is
based on early results by Kosten [3] considering
Poisson-traffic (A) offered a
fully available primary group (n) with
0
n 01
n 02
n 0g
k 0
A 0
A 01 ,n 01
M 01 ,V 01
M 02 ,V 02
M 0g ,V 0g
Figure 1 Basic overflow scheme of an alternative routing network
blocked calls given service in an infinite
overflow group (Figure 3).
The traffic process in a Kosten system
has the stationary state distribution
P(i,j) = P{I = i, J = j}
where I and J are stochastic variables
denoting the number of occupied primary
channels (0 ≤ I ≤ n) and the number of
occupied secondary channels (0 ≤ J ≤ ∞)
respectively. The formula of P(i,j) is
rather complicated but the lower
moments of overflow traffic are quite
simple and were given by Wilkinson as
the mean
n� ∞�
M = jP(i, j) =AEn(A)
A 0
and the variance
n� ∞�
V = j(j − 1)P (i, j)+M − M 2
l ı
1 g
A 0g ,n 0g
Transit node
Figure 2 Direct and alternative routes from one basic
node to g other ones
i=0 j=0
i=0 j=0
= M
�
�
A
1 − M +
n +1− A + M
l g
k 0
79