Weak Convergence Methods for Nonlinear Partial Differential ...

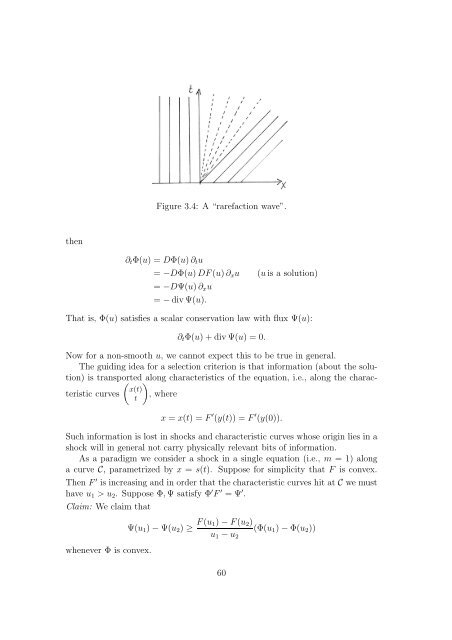
Figure 3.4: A “rarefaction wave”.
then
∂ t Φ(u) = DΦ(u) ∂ t u
= −DΦ(u) DF(u) ∂ x u (u is a solution)
= −DΨ(u) ∂ x u
= − div Ψ(u).
That is, Φ(u) satisfies a scalar conservation law with flux Ψ(u):
∂ t Φ(u) + div Ψ(u) = 0.
Now for a non-smooth u, we cannot expect this to be true in general.
The guiding idea for a selection criterion is that information (about the solution)
is transported ( ) along characteristics of the equation, i.e., along the characteristic
curves , where
x(t)
t
x = x(t) = F ′ (y(t)) = F ′ (y(0)).
Such information is lost in shocks and characteristic curves whose origin lies in a
shock will in general not carry physically relevant bits of information.
As a paradigm we consider a shock in a single equation (i.e., m = 1) along
a curve C, parametrized by x = s(t). Suppose for simplicity that F is convex.
Then F ′ is increasing and in order that the characteristic curves hit at C we must
have u 1 > u 2 . Suppose Φ, Ψ satisfy Φ ′ F ′ = Ψ ′ .
Claim: We claim that
whenever Φ is convex.
Ψ(u 1 ) − Ψ(u 2 ) ≥ F(u 1) − F(u 2 )
u 1 − u 2
(Φ(u 1 ) − Φ(u 2 ))
60