GUIDE WAVE ANALYSIS AND FORECASTING - WMO

106
1
( )= 1
0 9999726
50 × 365 25× 2 ≈
–
. .
.
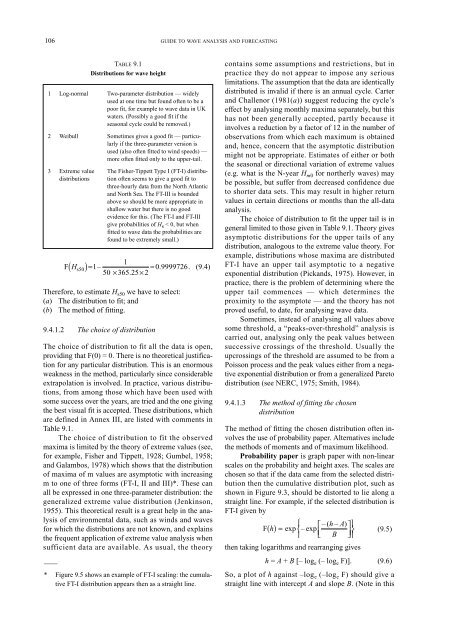
F Hs50 TABLE 9.1
Distributions for wave height
1 Log-normal Two-parameter distribution — widely
used at one time but found often to be a
poor fit, for example to wave data in UK
waters. (Possibly a good fit if the
seasonal cycle could be removed.)
2 Weibull Sometimes gives a good fit — particularly
if the three-parameter version is
used (also often fitted to wind speeds) —
more often fitted only to the upper-tail.
3 Extreme value The Fisher-Tippett Type I (FT-I) distribudistributions
tion often seems to give a good fit to
three-hourly data from the North Atlantic
and North Sea. The FT-III is bounded
above so should be more appropriate in
shallow water but there is no good
evidence for this. (The FT-I and FT-III
give probabilities of H s < 0, but when
fitted to wave data the probabilities are
found to be extremely small.)
Therefore, to estimate H s50 we have to select:
(a) The distribution to fit; and
(b) The method of fitting.
9.4.1.2 The choice of distribution
(9.4)
The choice of distribution to fit all the data is open,
providing that F(0) = 0. There is no theoretical justification
for any particular distribution. This is an enormous
weakness in the method, particularly since considerable
extrapolation is involved. In practice, various distributions,
from among those which have been used with
some success over the years, are tried and the one giving
the best visual fit is accepted. These distributions, which
are defined in Annex III, are listed with comments in
Table 9.1.
The choice of distribution to fit the observed
maxima is limited by the theory of extreme values (see,
for example, Fisher and Tippett, 1928; Gumbel, 1958;
and Galambos, 1978) which shows that the distribution
of maxima of m values are asymptotic with increasing
m to one of three forms (FT-I, II and III)*. These can
all be expressed in one three-parameter distribution: the
generalized extreme value distribution (Jenkinson,
1955). This theoretical result is a great help in the analysis
of environmental data, such as winds and waves
for which the distributions are not known, and explains
the frequent application of extreme value analysis when
sufficient data are available. As usual, the theory
____
* Figure 9.5 shows an example of FT-I scaling: the cumulative
FT-I distribution appears then as a straight line.
GUIDE TO WAVE ANALYSIS AND FORECASTING
contains some assumptions and restrictions, but in
practice they do not appear to impose any serious
limitations. The assumption that the data are identically
distributed is invalid if there is an annual cycle. Carter
and Challenor (1981(a)) suggest reducing the cycle’s
effect by analysing monthly maxima separately, but this
has not been generally accepted, partly because it
involves a reduction by a factor of 12 in the number of
observations from which each maximum is obtained
and, hence, concern that the asymptotic distribution
might not be appropriate. Estimates of either or both
the seasonal or directional variation of extreme values
(e.g. what is the N-year H m0 for northerly waves) may
be possible, but suffer from decreased confidence due
to shorter data sets. This may result in higher return
values in certain directions or months than the all-data
analysis.
The choice of distribution to fit the upper tail is in
general limited to those given in Table 9.1. Theory gives
asymptotic distributions for the upper tails of any
distribution, analogous to the extreme value theory. For
example, distributions whose maxima are distributed
FT-I have an upper tail asymptotic to a negative
exponential distribution (Pickands, 1975). However, in
practice, there is the problem of determining where the
upper tail commences — which determines the
proximity to the asymptote — and the theory has not
proved useful, to date, for analysing wave data.
Sometimes, instead of analysing all values above
some threshold, a “peaks-over-threshold” analysis is
carried out, analysing only the peak values between
successive crossings of the threshold. Usually the
upcrossings of the threshold are assumed to be from a
Poisson process and the peak values either from a negative
exponential distribution or from a generalized Pareto
distribution (see NERC, 1975; Smith, 1984).
9.4.1.3 The method of fitting the chosen
distribution
The method of fitting the chosen distribution often involves
the use of probability paper. Alternatives include
the methods of moments and of maximum likelihood.
Probability paper is graph paper with non-linear
scales on the probability and height axes. The scales are
chosen so that if the data came from the selected distribution
then the cumulative distribution plot, such as
shown in Figure 9.3, should be distorted to lie along a
straight line. For example, if the selected distribution is
FT-I given by
⎧ h A
F( h)
=
⎡ – ( – ) ⎤⎫
exp ⎨–
exp ⎬ (9.5)
⎩ ⎣⎢ B ⎦⎥
⎭
then taking logarithms and rearranging gives
h = A + B [– loge (– loge F)]. (9.6)
So, a plot of h against –loge (–loge F) should give a
straight line with intercept A and slope B. (Note in this