GUIDE WAVE ANALYSIS AND FORECASTING - WMO

4
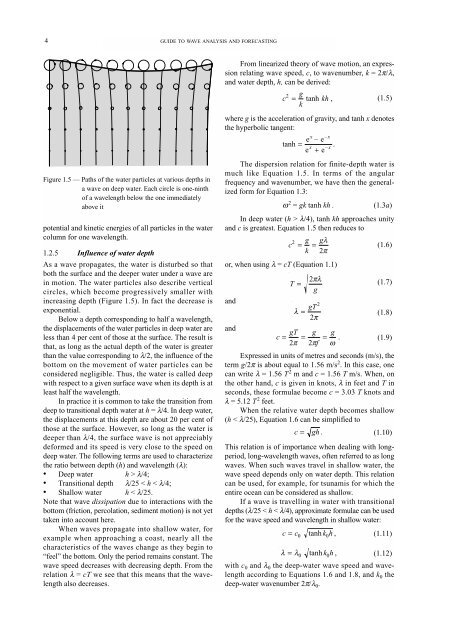
Figure 1.5 — Paths of the water particles at various depths in
a wave on deep water. Each circle is one-ninth
of a wavelength below the one immediately
above it
potential and kinetic energies of all particles in the water
column for one wavelength.
1.2.5 Influence of water depth
As a wave propagates, the water is disturbed so that
both the surface and the deeper water under a wave are
in motion. The water particles also describe vertical
circles, which become progressively smaller with
increasing depth (Figure 1.5). In fact the decrease is
exponential.
Below a depth corresponding to half a wavelength,
the displacements of the water particles in deep water are
less than 4 per cent of those at the surface. The result is
that, as long as the actual depth of the water is greater
than the value corresponding to λ/2, the influence of the
bottom on the movement of water particles can be
considered negligible. Thus, the water is called deep
with respect to a given surface wave when its depth is at
least half the wavelength.
In practice it is common to take the transition from
deep to transitional depth water at h = λ/4. In deep water,
the displacements at this depth are about 20 per cent of
those at the surface. However, so long as the water is
deeper than λ/4, the surface wave is not appreciably
deformed and its speed is very close to the speed on
deep water. The following terms are used to characterize
the ratio between depth (h) and wavelength (λ):
• Deep water h > λ/4;
• Transitional depth λ/25 < h < λ/4;
• Shallow water h < λ/25.
Note that wave dissipation due to interactions with the
bottom (friction, percolation, sediment motion) is not yet
taken into account here.
When waves propagate into shallow water, for
example when approaching a coast, nearly all the
characteristics of the waves change as they begin to
“feel” the bottom. Only the period remains constant. The
wave speed decreases with decreasing depth. From the
relation λ = cT we see that this means that the wavelength
also decreases.
GUIDE TO WAVE ANALYSIS AND FORECASTING
From linearized theory of wave motion, an expression
relating wave speed, c, to wavenumber, k = 2π/λ,
and water depth, h, can be derived:
2 g
c = tanh kh ,
(1.5)
k
where g is the acceleration of gravity, and tanh x denotes
the hyperbolic tangent:
The dispersion relation for finite-depth water is
much like Equation 1.5. In terms of the angular
frequency and wavenumber, we have then the generalized
form for Equation 1.3:
ω 2 = gk tanh kh . (1.3a)
In deep water (h > λ/4), tanh kh approaches unity
and c is greatest. Equation 1.5 then reduces to
2 g g
c = = (1.6)
k 2
or, when using λ = cT (Equation 1.1)
λ
π
and
and
e – e
tanh =
e + e
T
x – x
x – x
=
g
2πλ
λ =
π
gT 2
2
gT g g
c = = =
2π 2πfω
.
(1.7)
(1.8)
(1.9)
Expressed in units of metres and seconds (m/s), the
term g/2π is about equal to 1.56 m/s 2 . In this case, one
can write λ = 1.56 T 2 m and c = 1.56 T m/s. When, on
the other hand, c is given in knots, λ in feet and T in
seconds, these formulae become c = 3.03 T knots and
λ = 5.12 T 2 feet.
When the relative water depth becomes shallow
(h < λ/25), Equation 1.6 can be simplified to
c = gh.
(1.10)
This relation is of importance when dealing with longperiod,
long-wavelength waves, often referred to as long
waves. When such waves travel in shallow water, the
wave speed depends only on water depth. This relation
can be used, for example, for tsunamis for which the
entire ocean can be considered as shallow.
If a wave is travelling in water with transitional
depths (λ/25 < h < λ/4), approximate formulae can be used
for the wave speed and wavelength in shallow water:
c = c0 tanh k0h, (1.11)
λ = λ0
tanh kh 0 , (1.12)
with c0 and λ0 the deep-water wave speed and wavelength
according to Equations 1.6 and 1.8, and k0 the
deep-water wavenumber 2π/λ0. .