GUIDE WAVE ANALYSIS AND FORECASTING - WMO

eflected waves, although the latter are usually negligible.
In that case the technique can be readily
illustrated with Huygens’ principle. Each point on a crest
is the source of a new wave on the down-wave side. If
the waves are undisturbed, the next down-wave crest can
be constructed from the point sources on the seaward
crest. When the wave propagation is interrupted by an
obstacle, the down-wave crests are constructed only
from those seaward point sources that can radiate
beyond the obstacle (along straight lines). This technique
of reconstructing diffraction has been transferred into a
graphical technique (the Cornu spiral, Lacombe, 1965).
Graphs for standard situations are available to use for
rough estimates (e.g. CERC, 1973; Wiegel, 1964).
A joint theory for refraction and diffraction (for
cases with an uneven sea-bed) is available in the mildslope
equation of Berkhoff (1972). The key assumption
for mild slope is that depth variations are gradual but
horizontal variations in wave characteristics may be
rapid, as in refraction (see for example, Section 3.5 of
Mei, 1989). The Berkhoff equation is a generalization of
the Helmholtz equation which is the basis of the above
Sommerfeld solution. However, the computational
demands are rather severe in terms of computer capacity
and an approach based on the full mild-slope equation is
of practical use only for small areas with dimensions of a
few wavelengths (Booij et al., 1992).
The mild-slope equation includes the effects of
wave reflections (e.g. from steep bottom slopes and
obstacles). For waves that approach a coast with mild
slopes, the reflection may often be neglected, usually
implying that only the wave height variations along the
wave crest are relevant (and not the variations in the wave
direction). This provides a computational option (the
parabolic approach) in which the solution is obtained
simultaneously for all points along a line more or less
aligned with the wave crests. The solution then moves
WAVES IN SHALLOW WATER 85
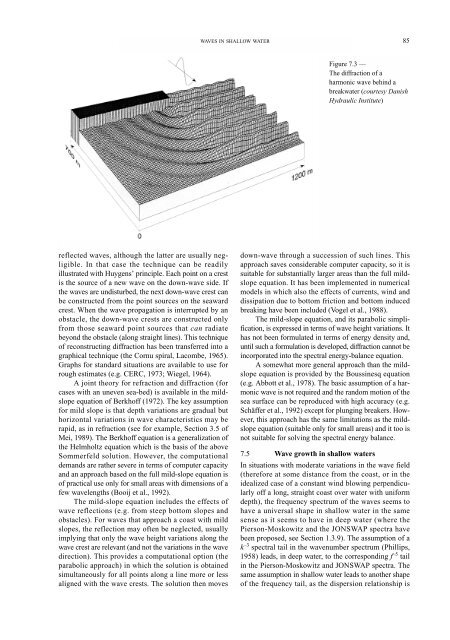
Figure 7.3 —
The diffraction of a
harmonic wave behind a
breakwater (courtesy Danish
Hydraulic Institute)
down-wave through a succession of such lines. This
approach saves considerable computer capacity, so it is
suitable for substantially larger areas than the full mildslope
equation. It has been implemented in numerical
models in which also the effects of currents, wind and
dissipation due to bottom friction and bottom induced
breaking have been included (Vogel et al., 1988).
The mild-slope equation, and its parabolic simplification,
is expressed in terms of wave height variations. It
has not been formulated in terms of energy density and,
until such a formulation is developed, diffraction cannot be
incorporated into the spectral energy-balance equation.
A somewhat more general approach than the mildslope
equation is provided by the Boussinesq equation
(e.g. Abbott et al., 1978). The basic assumption of a harmonic
wave is not required and the random motion of the
sea surface can be reproduced with high accuracy (e.g.
Schäffer et al., 1992) except for plunging breakers. However,
this approach has the same limitations as the mildslope
equation (suitable only for small areas) and it too is
not suitable for solving the spectral energy balance.
7.5 Wave growth in shallow waters
In situations with moderate variations in the wave field
(therefore at some distance from the coast, or in the
idealized case of a constant wind blowing perpendicularly
off a long, straight coast over water with uniform
depth), the frequency spectrum of the waves seems to
have a universal shape in shallow water in the same
sense as it seems to have in deep water (where the
Pierson-Moskowitz and the JONSWAP spectra have
been proposed, see Section 1.3.9). The assumption of a
k –3 spectral tail in the wavenumber spectrum (Phillips,
1958) leads, in deep water, to the corresponding f –5 tail
in the Pierson-Moskowitz and JONSWAP spectra. The
same assumption in shallow water leads to another shape
of the frequency tail, as the dispersion relationship is