GUIDE WAVE ANALYSIS AND FORECASTING - WMO

82
in the direction of wave propagation. Any decrease in cg is therefore accompanied by a corresponding increase in
wave height (and vice versa, any increase in cg by a
decrease in wave height). This is readily illustrated with
a wave perpendicular to a straight coast. Its wave height
can be determined from this conservation of energy
transport (i.e. assuming no dissipation), with:
H 2 =
(7.3)
where H is the wave height and the subscripts 1 and 2
refer to any two different locations along the (straight)
path of the wave.
The inclusion of shoaling in an Eulerian discrete
spectral wave model as described in Section 5.3 is relatively
simple. Only the determination of c g,x and c g,y in
Equation 5.4 needs to be adapted as described above by
c g = βc phase.
7.3 Refraction
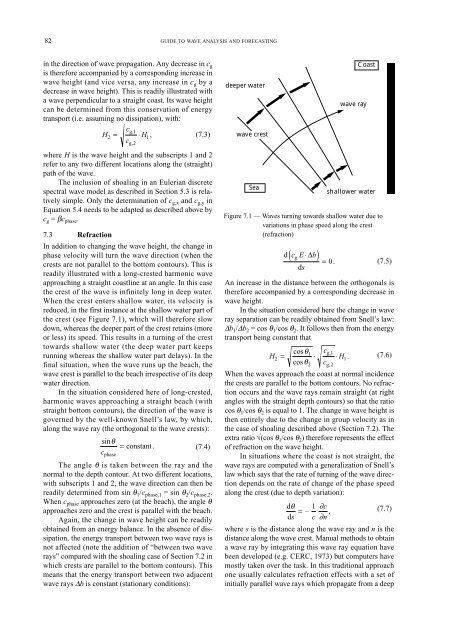
In addition to changing the wave height, the change in
phase velocity will turn the wave direction (when the
crests are not parallel to the bottom contours). This is
readily illustrated with a long-crested harmonic wave
approaching a straight coastline at an angle. In this case
the crest of the wave is infinitely long in deep water.
When the crest enters shallow water, its velocity is
reduced, in the first instance at the shallow water part of
the crest (see Figure 7.1), which will therefore slow
down, whereas the deeper part of the crest retains (more
or less) its speed. This results in a turning of the crest
towards shallow water (the deep water part keeps
running whereas the shallow water part delays). In the
final situation, when the wave runs up the beach, the
wave crest is parallel to the beach irrespective of its deep
water direction.
In the situation considered here of long-crested,
harmonic waves approaching a straight beach (with
straight bottom contours), the direction of the wave is
governed by the well-known Snell’s law, by which,
along the wave ray (the orthogonal to the wave crests):
sinθ
c g,1
c g,2
⋅H 1 ,
= constant.
(7.4)
cphase The angle θ is taken between the ray and the
normal to the depth contour. At two different locations,
with subscripts 1 and 2, the wave direction can then be
readily determined from sin θ1/cphase,1 = sin θ2/cphase,2. When cphase approaches zero (at the beach), the angle θ
approaches zero and the crest is parallel with the beach.
Again, the change in wave height can be readily
obtained from an energy balance. In the absence of dissipation,
the energy transport between two wave rays is
not affected (note the addition of “between two wave
rays” compared with the shoaling case of Section 7.2 in
which crests are parallel to the bottom contours). This
means that the energy transport between two adjacent
wave rays ∆b is constant (stationary conditions):
GUIDE TO WAVE ANALYSIS AND FORECASTING
deeper water
wave crest
Sea
d( cg E ⋅∆b)
= 0.
ds
(7.5)
An increase in the distance between the orthogonals is
therefore accompanied by a corresponding decrease in
wave height.
In the situation considered here the change in wave
ray separation can be readily obtained from Snell’s law:
∆b1/∆b2 = cos θ1/cos θ2. It follows then from the energy
transport being constant that
cosθ c 1 g,1
H (7.6)
2 = ⋅ ⋅H1 .
cosθ2 cg,2 When the waves approach the coast at normal incidence
the crests are parallel to the bottom contours. No refraction
occurs and the wave rays remain straight (at right
angles with the straight depth contours) so that the ratio
cos θ1/cos θ2 is equal to 1. The change in wave height is
then entirely due to the change in group velocity as in
the case of shoaling described above (Section 7.2). The
extra ratio √(cos θ1/cos θ2) therefore represents the effect
of refraction on the wave height.
In situations where the coast is not straight, the
wave rays are computed with a generalization of Snell’s
law which says that the rate of turning of the wave direction
depends on the rate of change of the phase speed
along the crest (due to depth variation):
dθ 1
= –
ds c
∂c
∂n ,
Coast
wave ray
shallower water
Figure 7.1 — Waves turning towards shallow water due to
variations in phase speed along the crest
(refraction)
(7.7)
where s is the distance along the wave ray and n is the
distance along the wave crest. Manual methods to obtain
a wave ray by integrating this wave ray equation have
been developed (e.g. CERC, 1973) but computers have
mostly taken over the task. In this traditional approach
one usually calculates refraction effects with a set of
initially parallel wave rays which propagate from a deep