A Semi-Implicit, Three-Dimensional Model for Estuarine ... - USGS
A Semi-Implicit, Three-Dimensional Model for Estuarine ... - USGS
A Semi-Implicit, Three-Dimensional Model for Estuarine ... - USGS
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
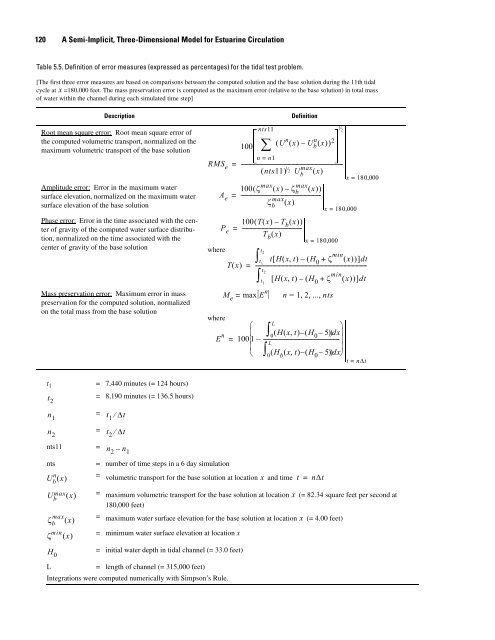
120 A <strong>Semi</strong>-<strong>Implicit</strong>, <strong>Three</strong>-<strong>Dimensional</strong> <strong>Model</strong> <strong>for</strong> <strong>Estuarine</strong> Circulation<br />
Table 5.5. Definition of error measures (expressed as percentages) <strong>for</strong> the tidal test problem.<br />
[The first three error measures are based on comparisons between the computed solution and the base solution during the 11th tidal<br />
cycle at x =180,000 feet. The mass preservation error is computed as the maximum error (relative to the base solution) in total mass<br />
of water within the channel during each simulated time step]<br />
Description Definition<br />
Root mean square error: Root mean square error of<br />
the computed volumetric transport, normalized on the<br />
maximum volumetric transport of the base solution<br />
Amplitude error: Error in the maximum water<br />
surface elevation, normalized on the maximum water<br />
surface elevation of the base solution<br />
Phase error: Error in the time associated with the center<br />
of gravity of the computed water surface distribution,<br />
normalized on the time associated with the<br />
center of gravity of the base solution<br />
Mass preservation error: Maximum error in mass<br />
preservation <strong>for</strong> the computed solution, normalized<br />
on the total mass from the base solution<br />
t 1 = 7,440 minutes (= 124 hours)<br />
t 2<br />
n 1<br />
n 2<br />
= 8,190 minutes (= 136.5 hours)<br />
= t1 ⁄ Δt<br />
= t2 ⁄ Δt<br />
RMS e<br />
A e<br />
P e<br />
where<br />
nts11 = n2 – n1 nts = number of time steps in a 6 day simulation<br />
n<br />
Ub ( x)<br />
nts11<br />
∑<br />
100<br />
U n n<br />
( ( x)<br />
– Ub ( x)<br />
) 2<br />
n = n1<br />
( nts11)<br />
1 = ---------------------------------------------------------------------------<br />
⁄ max 2 Ub ( x)<br />
100 ζ max max<br />
( ( x)<br />
– ζb ( x)<br />
)<br />
= ---------------------------------------------------------max<br />
ζb ( x)<br />
100( Tx ( ) – Tb( x)<br />
)<br />
= --------------------------------------------<br />
Tb( x)<br />
Tx ( )<br />
M e<br />
t2 ∫t1 t2 ∫t1 x = 180,000<br />
1⁄ 2<br />
x = 180,000<br />
x = 180,000<br />
tHxt ( , ) H0 ζ min [ – ( + ( x)<br />
) ]dt<br />
Hxt ( , ) H0 ζ min = ----------------------------------------------------------------------------------<br />
[ – ( + ( x)<br />
) ]dt<br />
max E n<br />
= n = 1, 2 , ... , nts<br />
= volumetric transport <strong>for</strong> the base solution at location x and time t = nΔt<br />
Umax b ( x)<br />
= maximum volumetric transport <strong>for</strong> the base solution at location x (= 82.34 square feet per second at<br />
180,000 feet)<br />
max<br />
ζb ( x)<br />
= maximum water surface elevation <strong>for</strong> the base solution at location x (= 4.00 feet)<br />
ζ min ( x)<br />
= minimum water surface elevation at location x<br />
H0 = initial water depth in tidal channel (= 33.0 feet)<br />
L = length of channel (= 315,000 feet)<br />
Integrations were computed numerically with Simpson’s Rule.<br />
where<br />
E n<br />
=<br />
⎛ L<br />
⎞<br />
⎜ ∫0( Hxt ( , ) – ( H0 – 5)<br />
)dx⎟<br />
100⎜1– -------------------------------------------------------- ⎟<br />
L<br />
⎜ ⎟<br />
⎝ ( Hb( x, t)<br />
– ( H0 – 5)<br />
)dx⎠<br />
∫ 0<br />
t = nΔt