Contents - Max-Planck-Institut für Physik komplexer Systeme

classical case, where the Boolean variables and clauses
take on discrete values – true or false – their quantum
generalizations are continuous: the states of a qubit
live in Hilbert space, which allows for linear combinations
of |0〉 (“false”) and |1〉 (“true”). Thinking of a
Boolean clause as forbidding one out of 2k configurations
leads to its quantum generalization as a projector
ΠI φ ≡ |φ〉〈φ|, which penalizes any overlap of a state |ψ〉
of the k qubits in set I with a state |φ〉 in their 2k dimensional
Hilbert space. In order to generate an instance of
k-QSAT the states |φ〉 (of unit norm) is picked randomly
in this Hilbert space.
Figure 3: Examples of random (hyper-) graphs for 2-SAT (left) and
3-SAT. Left: The clusters, clockwise from bottom left, are chain, tree,
clusters with one and two closed loops (“figure eight”). By our geometrisation
theorem the shaded objects play a role in determining
existence, and nature, of satisfying states. Right: Circles denote
qubits, and squares denote clauses.
The sum of these projectors defines a positive semidefinite
Hamiltonian
H =
M
m=1
Π Im
φm
and the decision problem for a given instance is, essentially,
to ask if there exists a state that simultaneously
satisfies all of the projectors, i.e. to determine whether
H has ground state energy, E0, exactly zero.
One of our most remarkable results is presented in the
following. The ensemble of problems we study is random
in two ways. Firstly, the interactions are represented
by random graphs. Secondly, the projectors are
drawn at random. It turns out that different realisations
of the latter does not exhibit a variation in satisfiability.
Specifically:
Geometrisation Theorem: Given an instance H of random
k-QSAT over a hypergraph G, the degeneracy of zero
energy states dim(ker(H)) takes a particular value D
with probability 1 with respect to the choice of projectors
on the edges of the hypergraph G.
This means that dim(ker(H)), which can be obtained
from solving a “hard” quantum problem, appears as a
property of a classical graph which, for k ≥ 3, has as
(1)
yet not been identified. For k = 2, an L-site tree has
D = L + 1, whereas a cluster with one closed loop has
D = 2, and D = 0 holds in the presence of more than
one closed loop (Fig. 3).
Besides the question about existence, and the number
D, of satisfying assignments, it is interesting to ask
about their nature. We have found that, for α < αPS,
there exists with probability 1 a satisfying assignment
that is a product state of qubits: |ΨPS〉 = ⊗ N i=1 |ψi〉. In
general, this holds when the interaction graph G permits
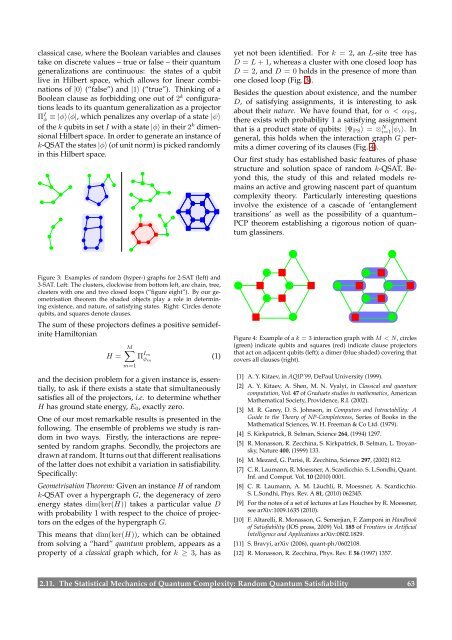
a dimer covering of its clauses (Fig. 4).
Our first study has established basic features of phase
structure and solution space of random k-QSAT. Beyond
this, the study of this and related models remains
an active and growing nascent part of quantum
complexity theory. Particularly interesting questions
involve the existence of a cascade of ‘entanglement
transitions’ as well as the possibility of a quantum–
PCP theorem establishing a rigorous notion of quantum
glassiners.
Figure 4: Example of a k = 3 interaction graph with M < N, circles
(green) indicate qubits and squares (red) indicate clause projectors
that act on adjacent qubits (left); a dimer (blue shaded) covering that
covers all clauses (right).
[1] A. Y. Kitaev, in AQIP’99, DePaul University (1999).
[2] A. Y. Kitaev, A. Shen, M. N. Vyalyi, in Classical and quantum
computation, Vol. 47 of Graduate studies in mathematics, American
Mathematical Society, Providence, R.I. (2002).
[3] M. R. Garey, D. S. Johnson, in Computers and Intractability: A
Guide to the Theory of NP-Completeness, Series of Books in the
Mathematical Sciences, W. H. Freeman & Co Ltd. (1979).
[4] S. Kirkpatrick, B. Selman, Science 264, (1994) 1297.
[5] R. Monasson, R. Zecchina, S. Kirkpatrick, B. Selman, L. Troyansky,
Nature 400, (1999) 133.
[6] M. Mezard, G. Parisi, R. Zecchina, Science 297, (2002) 812.
[7] C. R. Laumann, R. Moessner, A. Scardicchio. S. L.Sondhi, Quant.
Inf. and Comput. Vol. 10 (2010) 0001.
[8] C. R. Laumann, A. M. Läuchli, R. Moessner, A. Scardicchio.
S. L.Sondhi, Phys. Rev. A 81, (2010) 062345.
[9] For the notes of a set of lectures at Les Houches by R. Moessner,
see arXiv:1009.1635 (2010).
[10] F. Altarelli, R. Monasson, G. Semerjian, F. Zamponi in Handbook
of Satisfiability (IOS press, 2009) Vol. 185 of Frontiers in Artificial
Intelligence and Applications arXiv:0802.1829.
[11] S. Bravyi, arXiv (2006), quant-ph/0602108.
[12] R. Monasson, R. Zecchina, Phys. Rev. E 56 (1997) 1357.
2.11. The Statistical Mechanics of Quantum Complexity: Random Quantum Satisfiability 63