Contents - Max-Planck-Institut für Physik komplexer Systeme

ξ(N A =5)
ξ(N A =6)
20
10
0
20
10
BB
3 2 2 3
2 1 2
1 1
1
-3 -2 -1 0 1 2 3
∆K
0
-18 -12 -6 0
K
A
6 12 18
3
2
1
0
AB BA
AA
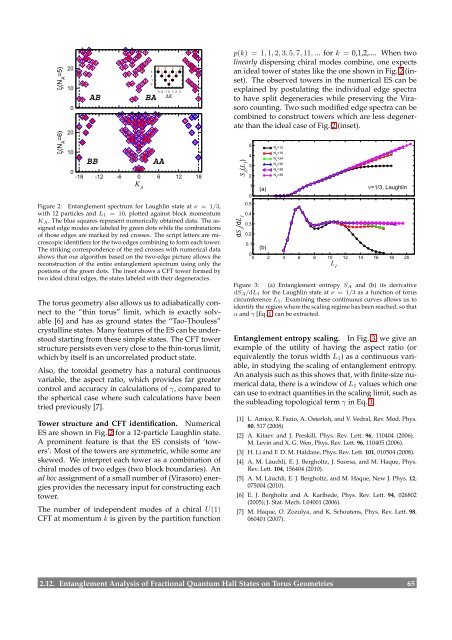
Figure 2: Entanglement spectrum for Laughlin state at ν = 1/3,
with 12 particles and L1 = 10, plotted against block momentum
KA. The blue squares represent numerically obtained data. The assigned
edge modes are labeled by green dots while the combinations
of those edges are marked by red crosses. The script letters are microscopic
identifiers for the two edges combining to form each tower.
The striking correspondence of the red crosses with numerical data
shows that our algorithm based on the two-edge picture allows the
reconstruction of the entire entanglement spectrum using only the
postions of the green dots. The inset shows a CFT tower formed by
two ideal chiral edges, the states labeled with their degeneracies.
The torus geometry also allows us to adiabatically connect
to the “thin torus” limit, which is exactly solvable
[6] and has as ground states the “Tao-Thouless”
crystalline states. Many features of the ES can be understood
starting from these simple states. The CFT tower
structure persists even very close to the thin-torus limit,
which by itself is an uncorrelated product state.
Also, the toroidal geometry has a natural continuous
variable, the aspect ratio, which provides far greater
control and accuracy in calculations of γ, compared to
the spherical case where such calculations have been
tried previously [7].
Tower structure and CFT identification. Numerical
ES are shown in Fig. 2 for a 12-particle Laughlin state.
A prominent feature is that the ES consists of ‘towers’.
Most of the towers are symmetric, while some are
skewed. We interpret each tower as a combination of
chiral modes of two edges (two block boundaries). An
ad hoc assignment of a small number of (Virasoro) energies
provides the necessary input for constructing each
tower.
The number of independent modes of a chiral U(1)
CFT at momentum k is given by the partition function
p(k) = 1,1,2,3,5,7,11,... for k = 0,1,2,.... When two
linearly dispersing chiral modes combine, one expects
an ideal tower of states like the one shown in Fig. 2 (inset).
The observed towers in the numerical ES can be
explained by postulating the individual edge spectra
to have split degeneracies while preserving the Virasoro
counting. Two such modified edge spectra can be
combined to construct towers which are less degenerate
than the ideal case of Fig. 2 (inset).
S A (L 1 )
dS A /dL 1
5
4
3
2
1
0
0.5
0.4
0.3
xxxxx xxxxxx xxxxx
xxxxxxx xxxxx
xx xx
xxxx x xxx
xxxxx xxxxx
xxxxxx xx
x x xxxxxx
N =12 s xxxxx N =18
xxxxxxxx s
N =24
xx
s
N =30
s
xxxN
=36
s
xxxN
=39
s
ν=1/3, Laughlin
(a)
xx
xxxxx
xx
x
xx xxxxxxxxxxxxxxxxxxxxxxx
xx xx
xxxx
xx xx xx xx
0.2
xx
0.1
0
0
(b)
2
xx
xx
4 6 8 10
L
1
12 14 16 18 20
Figure 3: (a) Entanglement entropy SA and (b) its derivative
dSA/dL1 for the Laughlin state at ν = 1/3 as a function of torus
circumference L1. Examining these continuous curves allows us to
identify the region where the scaling regime has been reached, so that
α and γ [Eq. 1] can be extracted.
Entanglement entropy scaling. In Fig. 3, we give an
example of the utility of having the aspect ratio (or
equivalently the torus width L1) as a continuous variable,
in studying the scaling of entanglement entropy.
An analysis such as this shows that, with finite-size numerical
data, there is a window of L1 values which one
can use to extract quantities in the scaling limit, such as
the subleading topological term γ in Eq. 1.
[1] L. Amico, R. Fazio, A. Osterloh, and V. Vedral, Rev. Mod. Phys.
80, 517 (2008)
[2] A. Kitaev and J. Preskill, Phys. Rev. Lett. 96, 110404 (2006).
M. Levin and X. G. Wen, Phys. Rev. Lett. 96, 110405 (2006).
[3] H. Li and F. D. M. Haldane, Phys. Rev. Lett. 101, 010504 (2008).
[4] A. M. Läuchli, E. J. Bergholtz, J. Suorsa, and M. Haque, Phys.
Rev. Lett. 104, 156404 (2010).
[5] A. M. Läuchli, E. J. Bergholtz, and M. Haque, New J. Phys. 12,
075004 (2010).
[6] E. J. Bergholtz and A. Karlhede, Phys. Rev. Lett. 94, 026802
(2005); J. Stat. Mech. L04001 (2006).
[7] M. Haque, O. Zozulya, and K. Schoutens, Phys. Rev. Lett. 98,
060401 (2007).
2.12. Entanglement Analysis of Fractional Quantum Hall States on Torus Geometries 65