history of mathematics - National STEM Centre

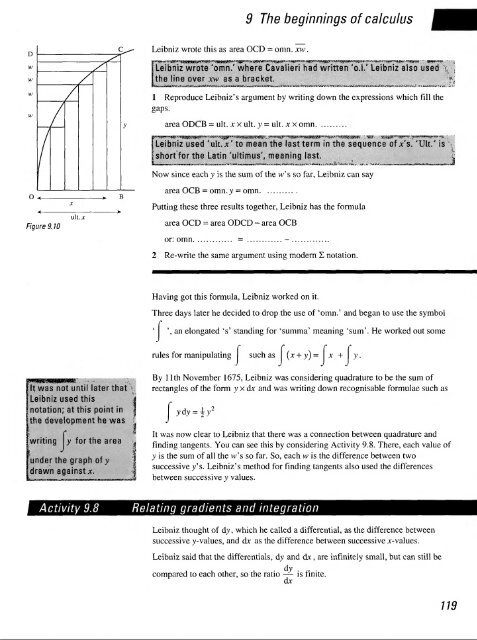
Figure 9.10
u\t.x
It was not until later that
Leibniz used this
notation; at this point in
the development he was
f
writing \y for the area
under the graph of y ;i
drawn against x. ;
Leibniz wrote this as area OCD = omn. xw.
9 The beginnings of calculus
Leibniz wrote 'omn.' where Cavalieri had written '0.1,' Leibniz also used
the line over xw as a bracket.
1 Reproduce Leibniz's argument by writing down the expressions which fill the
gaps.
area ODCB = ult. x x ult. y = ult. x x omn. .........
Leibniz used "ult. x' to mean the last term in the sequence of x's. 'Ult.' is
short for the Latin 'ultimus', meaning last.
Now since each y is the sum of the w's so far, Leibniz can say
area OCB = omn. y = omn. ..........
Putting these three results together, Leibniz has the formula
area OCD = area ODCD - area OCB
or: omn. ............ = ..........................
2 Re-write the same argument using modern Z notation.
Having got this formula, Leibniz worked on it.
Three days later he decided to drop the use of 'omn.' and began to use the symbol
', an elongated 's' standing for 'summa' meaning 'sum'. He worked out some
f f f f
rules for manipulating such as \ (x + y) = \ x + \ y .
-/ J J m/
By 1 1th November 1675, Leibniz was considering quadrature to be the sum of
rectangles of the form y x dx and was writing down recognisable formulae such as
It was now clear to Leibniz that there was a connection between quadrature and
finding tangents. You can see this by considering Activity 9.8. There, each value of
y is the sum of all the w's so far. So, each w is the difference between two
successive /s. Leibniz's method for finding tangents also used the differences
between successive y values.
Activity 9.8 Relating gradients and integration
Leibniz thought of Ay, which he called a differential, as the difference between
successive y-values, and Ax as the difference between successive x-values.
Leibniz said that the differentials, dy and dx, are infinitely small, but can still be
dy
compared to each other, so the ratio — is finite.
dx
119