history of mathematics - National STEM Centre
history of mathematics - National STEM Centre
history of mathematics - National STEM Centre
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
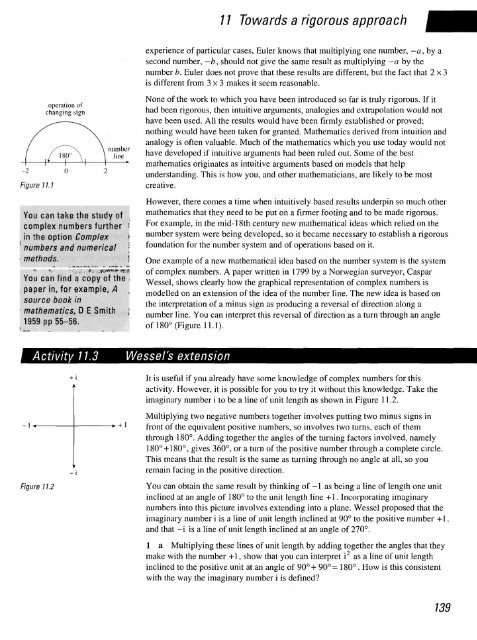
operation <strong>of</strong><br />
changing sign<br />
number<br />
line<br />
You can take the study <strong>of</strong><br />
complex numbers further<br />
in the option Complex<br />
numbers and numerical<br />
methods.<br />
You can find a copy <strong>of</strong> the<br />
paper in, for example, A<br />
source book in<br />
<strong>mathematics</strong>, D E Smith<br />
1959 pp 55-56. ,,<br />
Activity 11.3 Wessel's extension<br />
-1 .+1<br />
Figure 11.2<br />
7 7 Towards a rigorous approach<br />
experience <strong>of</strong> particular cases, Euler knows that multiplying one number, -a, by a<br />
second number, —b, should not give the same result as multiplying -a by the<br />
number b. Euler does not prove that these results are different, but the fact that 2x3<br />
is different from 3x3 makes it seem reasonable.<br />
None <strong>of</strong> the work to which you have been introduced so far is truly rigorous. If it<br />
had been rigorous, then intuitive arguments, analogies and extrapolation would not<br />
have been used. All the results would have been firmly established or proved;<br />
nothing would have been taken for granted. Mathematics derived from intuition and<br />
analogy is <strong>of</strong>ten valuable. Much <strong>of</strong> the <strong>mathematics</strong> which you use today would not<br />
have developed if intuitive arguments had been ruled out. Some <strong>of</strong> the best<br />
<strong>mathematics</strong> originates as intuitive arguments based on models that help<br />
understanding. This is how you, and other mathematicians, are likely to be most<br />
creative.<br />
However, there comes a time when intuitively based results underpin so much other<br />
<strong>mathematics</strong> that they need to be put on a firmer footing and to be made rigorous.<br />
For example, in the mid-18th century new mathematical ideas which relied on the<br />
number system were being developed, so it became necessary to establish a rigorous<br />
foundation for the number system and <strong>of</strong> operations based on it.<br />
One example <strong>of</strong> a new mathematical idea based on the number system is the system<br />
<strong>of</strong> complex numbers. A paper written in 1799 by a Norwegian surveyor, Caspar<br />
Wessel, shows clearly how the graphical representation <strong>of</strong> complex numbers is<br />
modelled on an extension <strong>of</strong> the idea <strong>of</strong> the number line. The new idea is based on<br />
the interpretation <strong>of</strong> a minus sign as producing a reversal <strong>of</strong> direction along a<br />
number line. You can interpret this reversal <strong>of</strong> direction as a turn through an angle<br />
<strong>of</strong> 180° (Figure 11.1).<br />
It is useful if you already have some knowledge <strong>of</strong> complex numbers for this<br />
activity. However, it is possible for you to try it without this knowledge. Take the<br />
imaginary number i to be a line <strong>of</strong> unit length as shown in Figure 11.2.<br />
Multiplying two negative numbers together involves putting two minus signs in<br />
front <strong>of</strong> the equivalent positive numbers, so involves two turns, each <strong>of</strong> them<br />
through 180°. Adding together the angles <strong>of</strong> the turning factors involved, namely<br />
180°+180°, gives 360°, or a turn <strong>of</strong> the positive number through a complete circle.<br />
This means that the result is the same as turning through no angle at all, so you<br />
remain facing in the positive direction.<br />
You can obtain the same result by thinking <strong>of</strong> -1 as being a line <strong>of</strong> length one unit<br />
inclined at an angle <strong>of</strong> 180° to the unit length line +1. Incorporating imaginary<br />
numbers into this picture involves extending into a plane. Wessel proposed that the<br />
imaginary number i is a line <strong>of</strong> unit length inclined at 90° to the positive number +1.<br />
and that -i is a line <strong>of</strong> unit length inclined at an angle <strong>of</strong> 270°.<br />
1 a Multiplying these lines <strong>of</strong> unit length by adding together the angles that they<br />
make with the number +1, show that you can interpret i as a line <strong>of</strong> unit length<br />
inclined to the positive unit at an angle <strong>of</strong> 90° + 90° = 180°. How is this consistent<br />
with the way the imaginary number i is defined?<br />
139