history of mathematics - National STEM Centre

Searching for the abstract
Activity 10.6 The Greek version
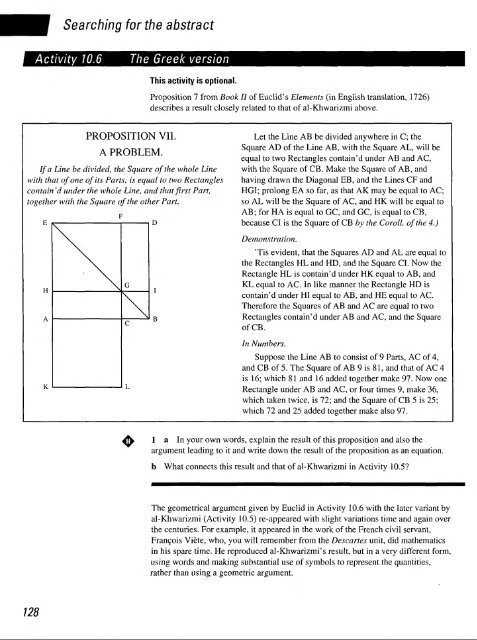
PROPOSITION VII.
A PROBLEM.
This activity is optional.
If a Line be divided, the Square of the whole Line
with that of one of its Parts, is equal to two Rectangles
contain 'd under the whole Line, and that first Part,
together with the Square of the other Part.
128
Proposition 7 from Book II of Euclid's Elements (in English translation, 1726)
describes a result closely related to that of al-Khwarizmi above.
Let the Line AB be divided anywhere in C; the
Square AD of the Line AB, with the Square AL, will be
equal to two Rectangles contain'd under AB and AC,
with the Square of CB. Make the Square of AB, and
having drawn the Diagonal EB, and the Lines CF and
HGI; prolong EA so far, as that AK may be equal to AC;
so AL will be the Square of AC, and HK will be equal to
AB; for HA is equal to GC, and GC, is equal to CB,
because CI is the Square of CB by the Coroll. of the 4.)
Demonstration.
"Pis evident, that the Squares AD and AL are equal to
the Rectangles HL and HD, and the Square CI. Now the
Rectangle HL is contain'd under HK equal to AB, and
KL equal to AC. In like manner the Rectangle HD is
contain'd under HI equal to AB, and HE equal to AC.
Therefore the Squares of AB and AC are equal to two
Rectangles contain'd under AB and AC, and the Square
ofCB.
In Numbers.
Suppose the Line AB to consist of 9 Parts, AC of 4,
and CB of 5. The Square of AB 9 is 81, and that of AC 4
is 16; which 81 and 16 added together make 97. Now one
Rectangle under AB and AC, or four times 9, make 36,
which taken twice, is 72; and the Square of CB 5 is 25;
which 72 and 25 added together make also 97.
1 a In your own words, explain the result of this proposition and also the
argument leading to it and write down the result of the proposition as an equation.
b What connects this result and that of al-Khwarizmi in Activity 10.5?
The geometrical argument given by Euclid in Activity 10.6 with the later variant by
al-Khwarizmi (Activity 10.5) re-appeared with slight variations time and again over
the centuries. For example, it appeared in the work of the French civil servant,
Fran9ois Viete, who, you will remember from the Descartes unit, did mathematics
in his spare time. He reproduced al-Khwarizmi's result, but in a very different form,
using words and making substantial use of symbols to represent the quantities,
rather than using a geometric argument.