history of mathematics - National STEM Centre

Activity 7.2 Reflecting on Descartes, 7
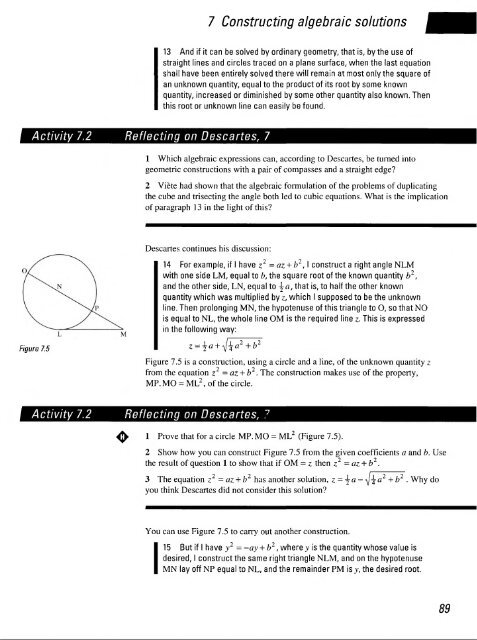
Figure 7.5
Activity 7.3
7 Constructing algebraic solutions
13 And if it can be solved by ordinary geometry, that is, by the use of
straight lines and circles traced on a plane surface, when the last equation
shall have been entirely solved there will remain at most only the square of
an unknown quantity, equal to the product of its root by some known
quantity, increased or diminished by some other quantity also known. Then
this root or unknown line can easily be found.
1 Which algebraic expressions can, according to Descartes, be turned into
geometric constructions with a pair of compasses and a straight edge?
2 Viete had shown that the algebraic formulation of the problems of duplicating
the cube and trisecting the angle both led to cubic equations. What is the implication
of paragraph 13 in the light of this?
Descartes continues his discussion:
14 For example, if I have z 2 = az + b 2 , 1 construct a right angle NLM
with one side LM, equal to b, the square root of the known quantity b 2 ,
and the other side, LN, equal to ^-a,that is, to half the other known
quantity which was multiplied by z, which I supposed to be the unknown
line. Then prolonging MN, the hypotenuse of this triangle to O, so that NO
is equal to NL, the whole line OM is the required line z. This is expressed
in the following way:
Figure 7.5 is a construction, using a circle and a line, of the unknown quantity z
from the equation z = az + b . The construction makes use of the property,
MP. MO = ML2 , of the circle.
Reflecting on Descartes, 8
1 Prove that for a circle MP. MO = ML2 (Figure 7.5).
2 Show how you can construct Figure 7.5 from the given coefficients a and b. Use
the result of question 1 to show that if OM = z then z 2 = az + b 2 .
3 The equation z 2 = az + b 2 has another solution, z =
you think Descartes did not consider this solution?
a 2 + b 2 . Why do
You can use Figure 7.5 to carry out another construction.
1
15 But if I have y 2 =-ay + b 2 , where y is the quantity whose value is
desired, I construct the same right triangle NLM, and on the hypotenuse
MN lay off NP equal to NL, and the remainder PM is y, the desired root.
89