history of mathematics - National STEM Centre

68
The Arabs
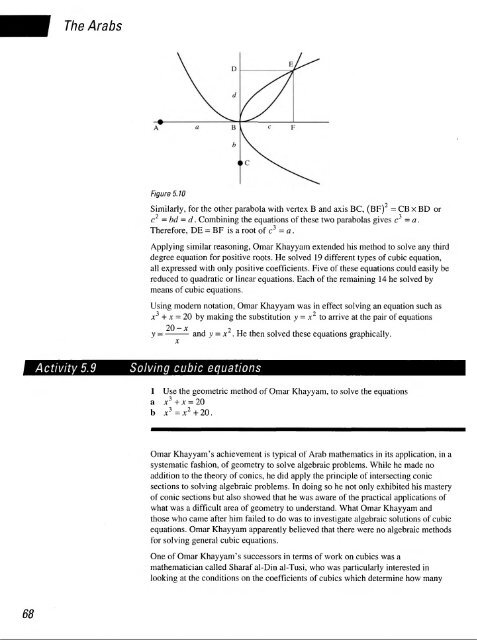
Figure 5.10
Activity 5.9 Solving cubic equations
Similarly, for the other parabola with vertex B and axis BC, (BF) = CB x BD or
c2 = bd = d. Combining the equations of these two parabolas gives c3 = a.
Therefore, DE = BF is a root of c 3 = a.
Applying similar reasoning, Omar Khayyam extended his method to solve any third
degree equation for positive roots. He solved 19 different types of cubic equation,
all expressed with only positive coefficients. Five of these equations could easily be
reduced to quadratic or linear equations. Each of the remaining 14 he solved by
means of cubic equations.
Using modern notation, Omar Khayyam was in effect solving an equation such as
x 3 + x = 20 by making the substitution y = x2 to arrive at the pair of equations
y = ——— and y = x2 . He then solved these equations graphically.
1 Use the geometric method of Omar Khayyam, to solve the equations
a ;t 3 + x = 20
b x 3 =i'
Omar Khayyam's achievement is typical of Arab mathematics in its application, in a
systematic fashion, of geometry to solve algebraic problems. While he made no
addition to the theory of conies, he did apply the principle of intersecting conic
sections to solving algebraic problems. In doing so he not only exhibited his mastery
of conic sections but also showed that he was aware of the practical applications of
what was a difficult area of geometry to understand. What Omar Khayyam and
those who came after him failed to do was to investigate algebraic solutions of cubic
equations. Omar Khayyam apparently believed that there were no algebraic methods
for solving general cubic equations.
One of Omar Khayyam's successors in terms of work on cubics was a
mathematician called Sharaf al-Din al-Tusi, who was particularly interested in
looking at the conditions on the coefficients of cubics which determine how many